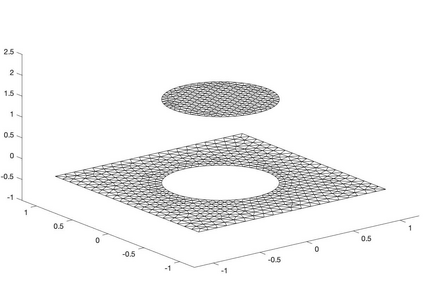
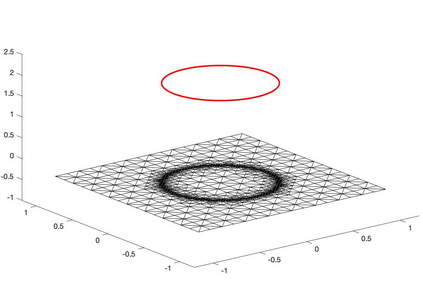
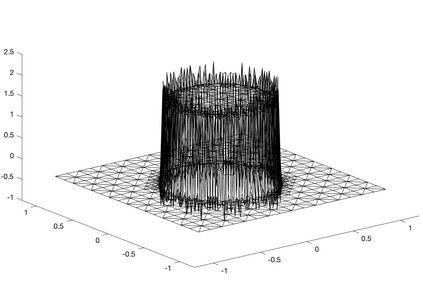
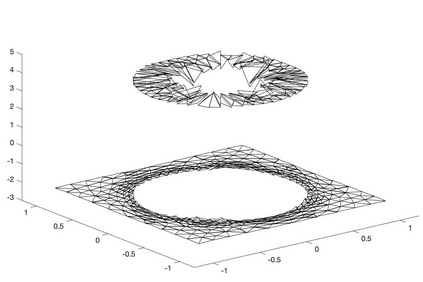
We consider the numerical approximation of a sharp-interface model for two-phase flow, which is given by the incompressible Navier-Stokes equations in the bulk domain together with the classical interface conditions on the interface. We propose structure-preserving finite element methods for the model, meaning in particular that volume preservation and energy decay are satisfied on the discrete level. For the evolving fluid interface, we employ parametric finite element approximations that introduce an implicit tangential velocity to improve the quality of the interface mesh. For the two-phase Navier-Stokes equations, we consider two different approaches: an unfitted and a fitted finite element method, respectively. In the unfitted approach, the constructed method is based on an Eulerian weak formulation, while in the fitted approach a novel arbitrary Lagrangian-Eulerian (ALE) weak formulation is introduced. Using suitable discretizations of these two formulations, we introduce two finite element methods and prove their structure-preserving properties. Numerical results are presented to show the accuracy and efficiency of the introduced methods.
翻译:我们考虑两相流程的锐界模型的数值逼近,该模型由流体力学的不可压缩的纳维-斯托克斯方程在大部分区域中与界面上的经典界面条件组成。我们为该模型提出了结构保持的有限元方法,特别是体积保持和能量衰减在离散级别上均满足。对于不断演化的流体界面,我们采用参数化有限元逼近,引入一种隐式切向速度来改善界面网格的质量。对于两相纳维-斯托克斯方程,我们考虑了两种不同的方法:拟合方法和非拟合方法。在非拟合方法中,构造的方法基于欧拉弱形式,而在拟合方法中则引入了一种新的任意拉格朗日-欧拉(ALE)弱形式。利用适当的这两种公式的离散化方法,我们引入了两种有限元方法,并证明它们的结构保持性质。为了展示所介绍的方法的准确性和效率,我们提供了数字结果。