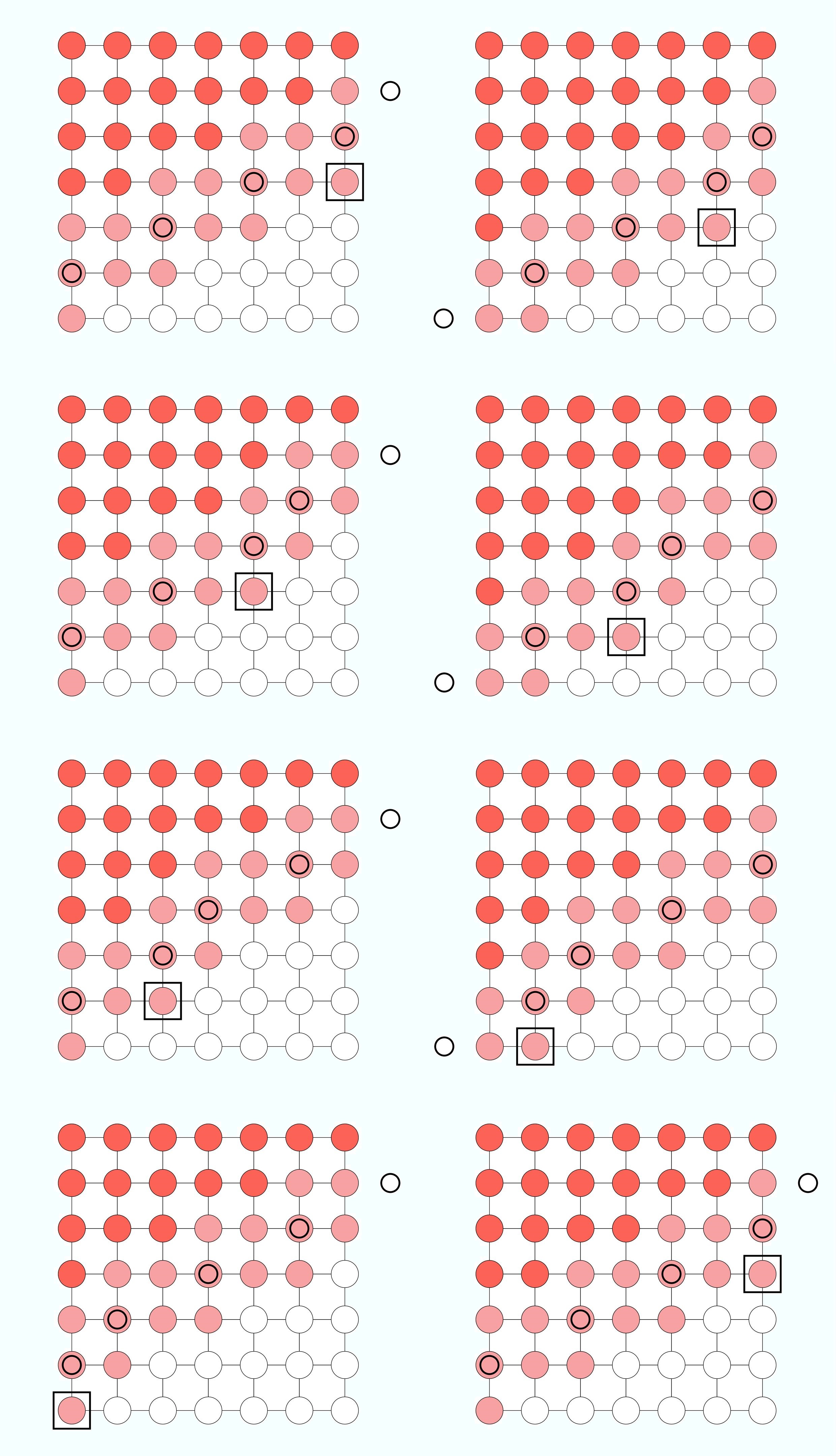
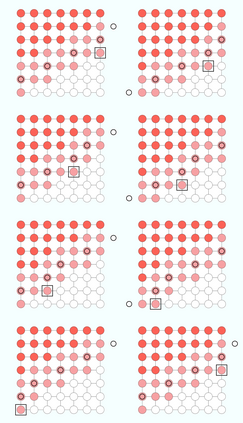
We introduce a variant of the Localization game in which the cops only have visibility one, along with the corresponding optimization parameter, the one-visibility localization number $\zeta_1$. By developing lower bounds using isoperimetric inequalities, we give upper and lower bounds for $\zeta_1$ on $k$-ary trees with $k\ge 2$ that differ by a multiplicative constant, showing that the parameter is unbounded on $k$-ary trees. We provide a $O(\sqrt{n})$ bound for $K_h$-minor free graphs of order $n$, and we show Cartesian grids meet this bound by determining their one-visibility localization number up to four values. We present upper bounds on $\zeta_1$ using pathwidth and the domination number and give upper bounds on trees via their depth and order. We conclude with open problems.
翻译:我们引入了一个本地化游戏的变式, 警察只有可见度之一, 加上相应的优化参数, 即一可见本地化数字$\zeta_ 1美元。 通过使用等光度不平等来开发较低界限, 我们给$Zeta_ 1美元给$k-marine树设定上下界值, 美元2美元与倍数常数不同, 显示该参数在 $k$- ary 树上不受约束。 我们提供了一可见本地化数字$( scrt{n} $_ h$- minor free point points $n$, 我们通过确定其一可见本地化数字最多为 4 个值, 显示Cartesian 电网达到了这一约束值。 我们使用路径维度和控制码显示 $\zeta_ 1 美元上界值, 并通过树的深度和顺序给树以上界值设定上界值。 我们以开放式问题结束 。