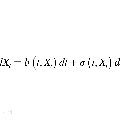We prove weak convergence of order one for a class of exponential based integrators for SDEs with non-globally Lipschtiz drift. Our analysis covers tamed versions of Geometric Brownian Motion (GBM) based methods as well as the standard exponential schemes. The numerical performance of both the GBM and exponential tamed methods through four different multi-level Monte Carlo techniques are compared. We observe that for linear noise the standard exponential tamed method requires severe restrictions on the stepsize unlike the GBM tamed method.
翻译:我们证明了一类SDE的指数积分器具有一阶弱收敛性质,其中漂移函数是非全局Lipschtiz的。我们的分析覆盖了自然版本的几何布朗运动(GBM)基础方法,以及标准指数方案的被驯服版本。我们比较了GBM和指数驯服方法的数值性能,并通过四种不同的多级蒙特卡洛技术进行了比较。我们观察到,在线性噪声的情况下,标准指数被驯服法需要对步长施加严格的限制,而GBM被驯服法则不需要。
相关内容
专知会员服务
46+阅读 · 2020年2月23日
专知会员服务
36+阅读 · 2019年10月17日
Arxiv
0+阅读 · 2023年6月5日
Arxiv
0+阅读 · 2023年6月3日
Arxiv
17+阅读 · 2022年11月2日