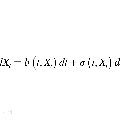分数Brown运动驱动的随机微分方程随机分岔与遍历性的研究

项目名称： 分数Brown运动驱动的随机微分方程随机分岔与遍历性的研究

项目编号： No.11501216

项目类型： 青年科学基金项目

立项/批准年度： 2016

项目学科： 数理科学和化学

项目作者： 曾才斌

作者单位： 华南理工大学

项目金额： 18万元

中文摘要： 分数Brown运动具有非半鞅性与非Markov性，因而其驱动的随机微分方程的研究方法与传统Itô分析的研究方法有本质的区别，需要新的数学理论和方法。本项目主要研究分数Brown运动驱动的随机微分方程的随机分岔与遍历性，这具有一定的挑战性和较强的应用意义。我们一方面有机结合Oseledec乘积遍历理论、分数Itô公式、Parseval公式及Bochner定理等方法，提出分数Brown运动驱动的随机分岔的合理定义，进一步探讨分数噪声诱导随机分岔的机理；另一方面，基于Malliavin理论和粗糙路径理论，借助截断函数技巧来改进正则情形遍历性的已有结果，利用Jacobi流上的先验估计深入研究粗糙路径情形遍历性。通过本项目的研究，期望对分数Brown运动驱动的随机微分方程复杂动力学行为的研究获得新的和更深刻的认识。

中文关键词： 随机分岔；遍历性；分数Brown运动；随机微分方程；粗糙路径

英文摘要： Fractional Brownian motion is non-semimartingale and non-Markov, so there are essential differences between stochastic differential equations driven by fractional Brownian motion and classical Itô analysis in research methodology, and new mathematical theory and method should be presented. This project is mainly devoted to study the stochastic bifurcation and ergodicity of stochastic differential equations driven by fractional Brownian motion, so it is challenging certainly and has strong application significance. On the one hand, we will propose the reasonable definitions of stochastic bifurcation driven by fractional Brownian motion, and further explore the undergoing mechanism of stochastic bifurcation by integrating the Oseledec's multiplicative ergodic theorem, fractional Itô formula, Parseval formula and Bochner theorem. On the other hand, based on the Malliavin theory and rough paths theory, we will improve the known results on ergodicity in the regular case with the help of the truncation function, and further study the ergodicity in the rough paths case by using prior estimates on the Jacobian of the flow. Therefore, the purpose of this project is to gain new and more profound understanding of the complex dynamics of stochastic differential equations driven by fractional Brownian motion.

英文关键词： stochastic bifurcation;ergodicity;fractional Brownian motion;stochastic differential equation;rough path