Joint Metrics for EMF Exposure and Coverage in Real-World Homogeneous and Inhomogeneous Cellular Networks

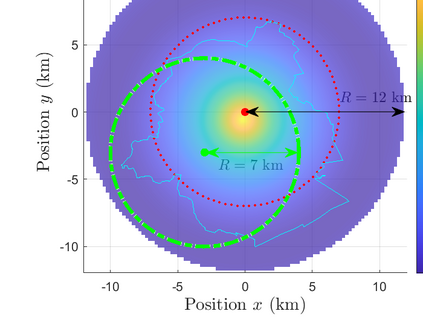
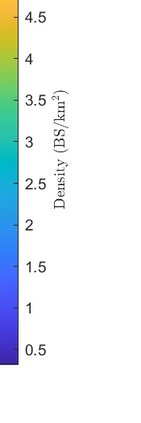
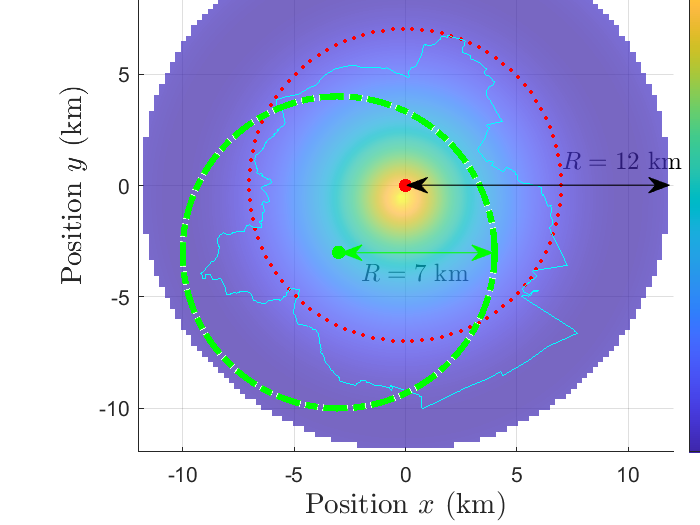
This paper evaluates the downlink performance of cellular networks in terms of coverage and electromagnetic field (EMF) exposure, in the framework of stochastic geometry. The model is constructed based on datasets for sub-6~GHz macro cellular networks but it is general enough to be applicable to millimeter-wave networks as well. On the one hand, performance metrics are calculated for $\beta$-Ginibre point processes which are shown to faithfully model a large number of motion-invariant networks. On the other hand, performance metrics are derived for inhomogeneous Poisson point processes with a radial intensity measure, which are shown to be a good approximation for motion-variant networks. For both cases, joint and marginal distributions of the EMF exposure and the coverage, and the first moments of the EMF exposure are provided and validated by Monte Carlo simulations using realistic sets of parameters from two sub-6~GHz macro urban cellular networks, i.e., 5G~NR~2100 (Paris, France) and LTE~1800 (Brussels, Belgium) datasets. In addition, this paper includes the analysis of the impact of the network parameters and discusses the achievable trade-off between coverage and EMF exposure.