Perpetual maintenance of machines with different urgency requirements

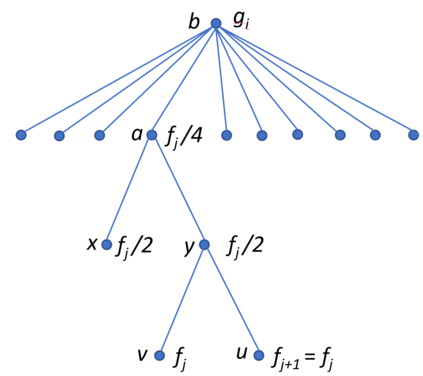
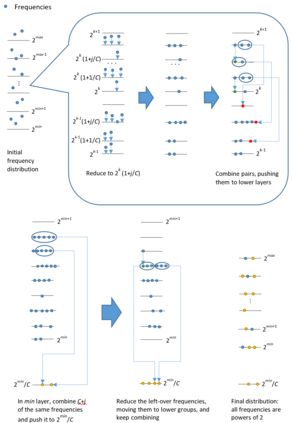
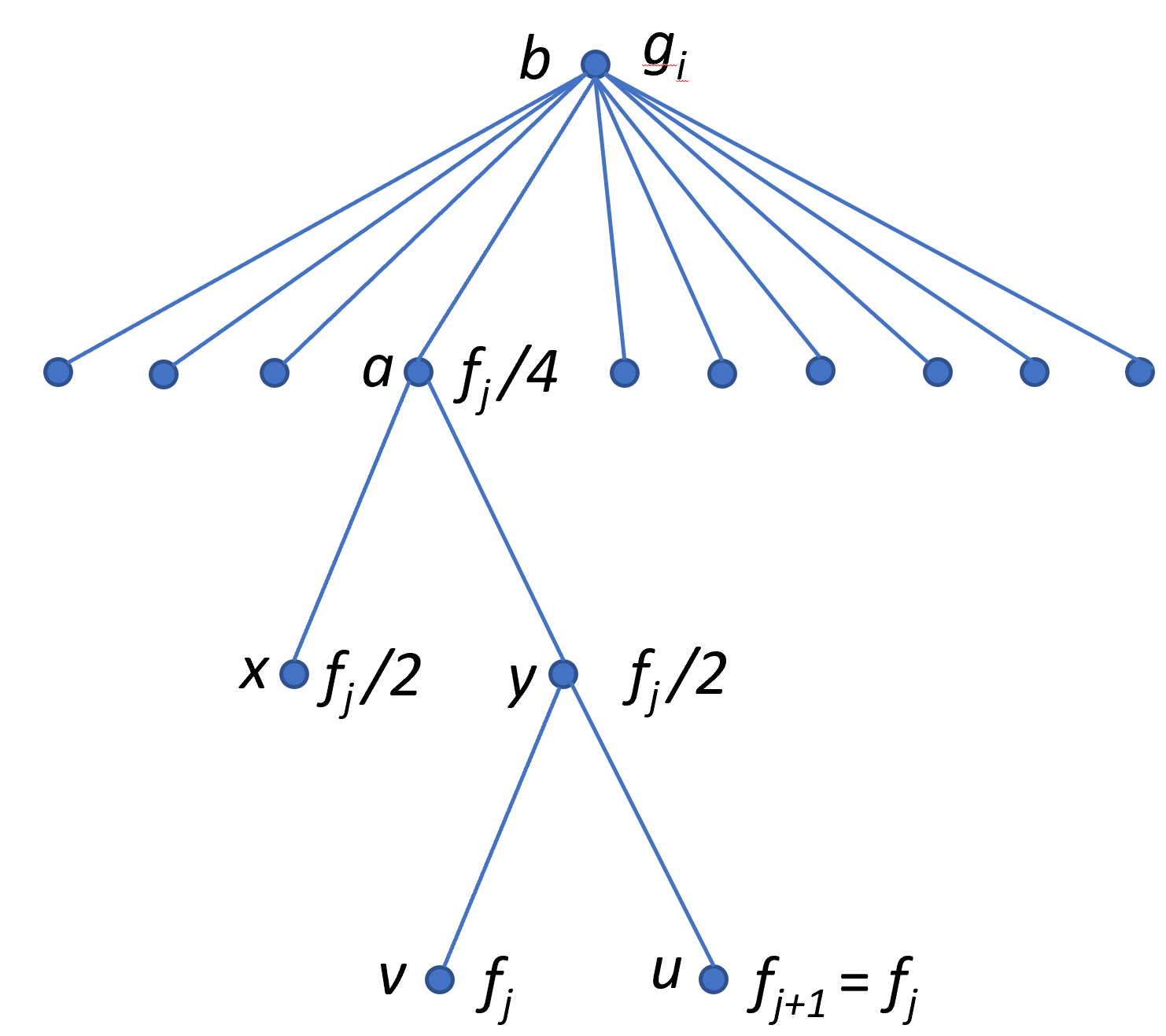
A garden $G$ is populated by $n\ge 1$ bamboos $b_1, b_2, ..., b_n$ with the respective daily growth rates $h_1 \ge h_2 \ge \dots \ge h_n$. It is assumed that the initial heights of bamboos are zero. The robotic gardener maintaining the garden regularly attends bamboos and trims them to height zero according to some schedule. The Bamboo Garden Trimming Problem (BGT) is to design a perpetual schedule of cuts to maintain the elevation of the bamboo garden as low as possible. The bamboo garden is a metaphor for a collection of machines which have to be serviced, with different frequencies, by a robot which can service only one machine at a time. The objective is to design a perpetual schedule of servicing which minimizes the maximum (weighted) waiting time for servicing. We consider two variants of BGT. In discrete BGT the robot trims only one bamboo at the end of each day. In continuous BGT the bamboos can be cut at any time, however, the robot needs time to move from one bamboo to the next. For discrete BGT, we show a simple $4$-approximation algorithm and, by exploiting relationship between BGT and the classical Pinwheel scheduling problem, we derive a $2$-approximation algorithm for the general case and a tighter approximation when the growth rates are balanced. A by-product of this last approximation algorithm is that it settles one of the conjectures about the Pinwheel problem. For continuous BGT, we propose approximation algorithms which achieve approximation ratios $O(\log (h_1/h_n))$ and $O(\log n)$.