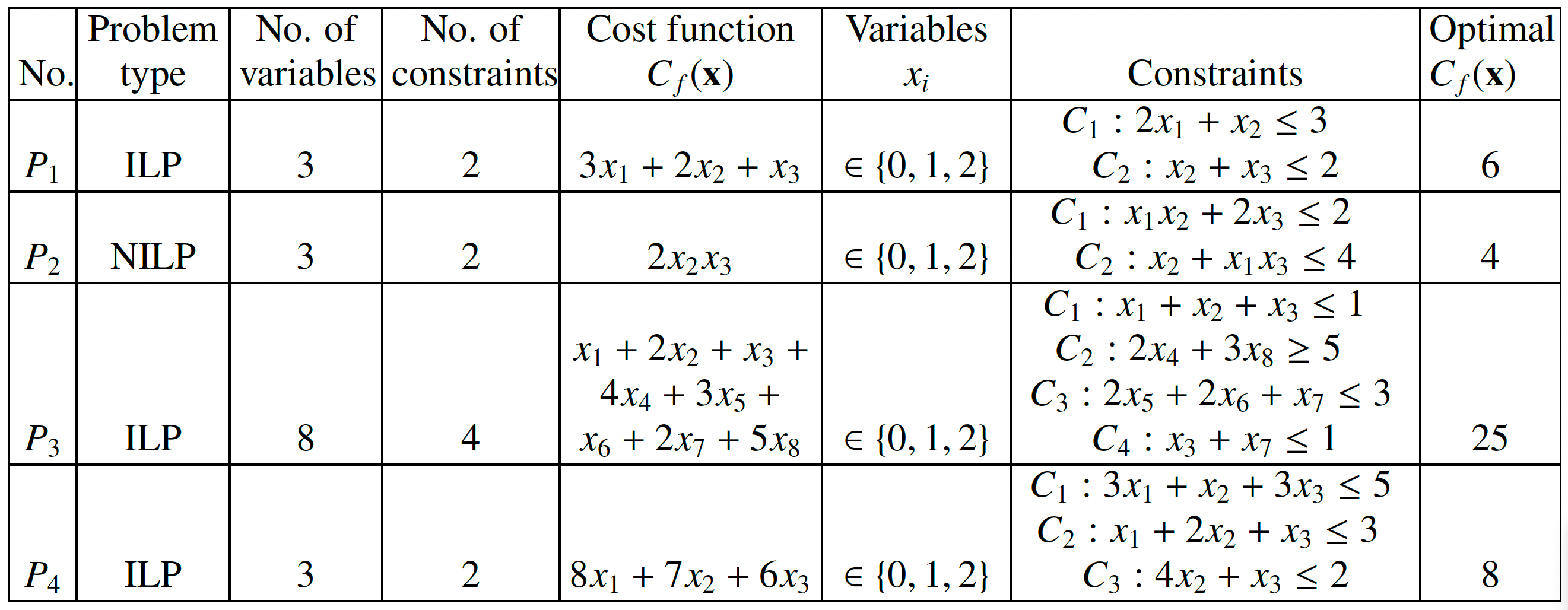
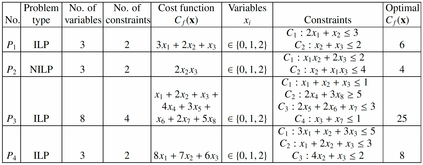
Integer programming (IP), as the name suggests is an integer-variable-based approach commonly used to formulate real-world optimization problems with constraints. Currently, quantum algorithms reformulate the IP into an unconstrained form through the use of binary variables, which is an indirect and resource-consuming way of solving it. We develop an algorithm that maps and solves an IP problem in its original form to any quantum system that possesses a large number of accessible internal degrees of freedom that can be controlled with sufficient accuracy. Using a single Rydberg atom as an example, we associate the integer values to electronic states belonging to different manifolds and implement a selective superposition of these different states to solve the full IP problem. The optimal solution is found within a few microseconds for prototypical IP problems with up to eight variables and a maximum number of four constraints. This also includes non-linear IP problems, which are usually harder to solve with classical algorithms when compared to their linear counterparts. Our algorithm for solving IP outperforms a well-known classical algorithm (branch and bound) in terms of the number of steps needed for convergence to the solution. Our approach carries the potential to improve bounds on the solution for larger problems when compared to the classical algorithms.
翻译:暂无翻译