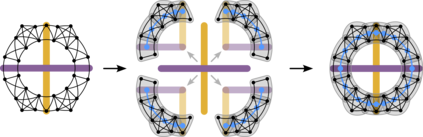
We provide a unique decomposition of every 4-connected graph into parts that are either quasi-5-connected, cycles of triangle-torsos and 3-connected torsos on $\leq 5$ vertices, generalised double-wheels, or thickened $K_{4,m}$'s. The decomposition can be described in terms of a tree-decomposition but with edges allowed in the adhesion-sets. Our construction is explicit, canonical, and exhibits a defining property of the Tutte-decomposition. As a corollary, we obtain a new Tutte-type canonical decomposition of 3-connected graphs into parts that are either quasi-4-connected, generalised wheels or thickened $K_{3,m}$'s. This decomposition is similar yet different from the tri-separation decomposition. As an application of the decomposition for 4-connectivity, we obtain a new theorem characterising all 4-connected vertex-transitive finite graphs as quasi-5-connected, the $K_4$-expansion of a quasi-5-connected graph, or on a short explicit list of graphs.
翻译:暂无翻译