Recovery of a Space-Time Dependent Diffusion Coefficient in Subdiffusion: Stability, Approximation and Error Analysis

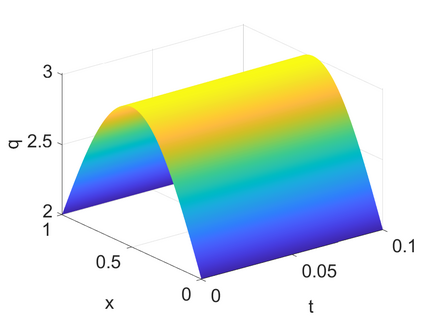
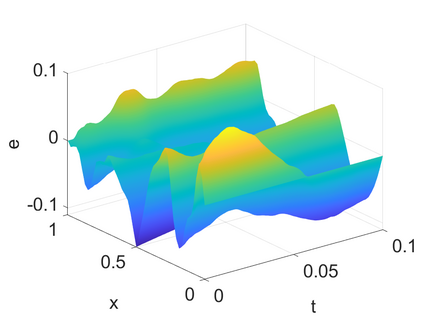
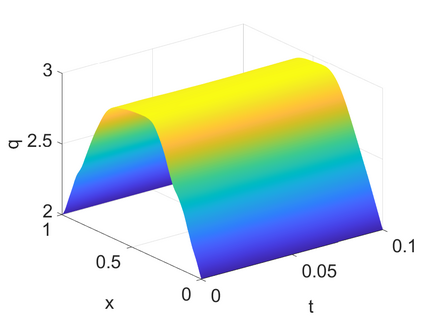
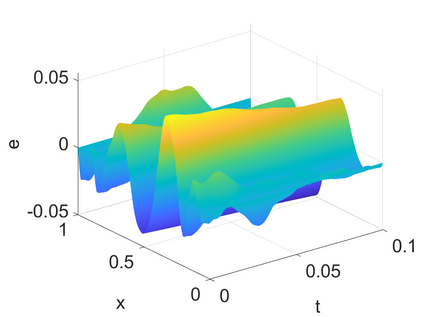
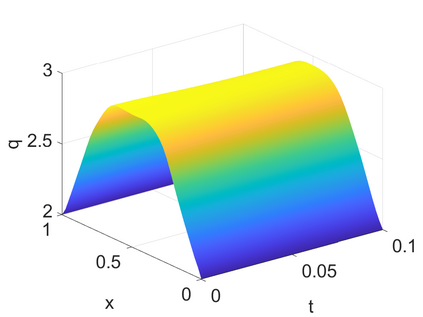
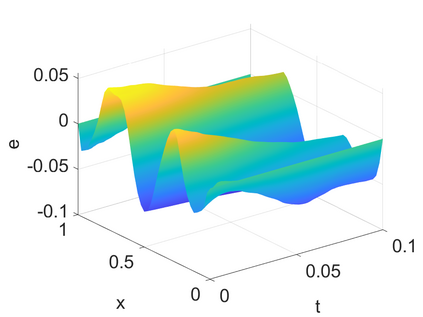
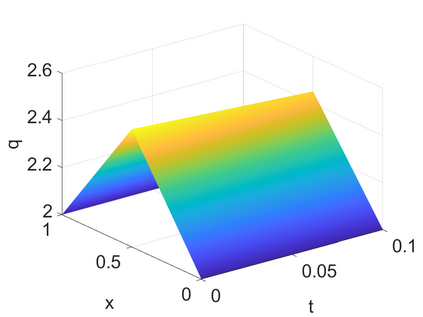
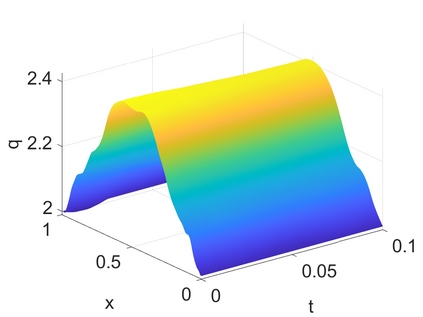
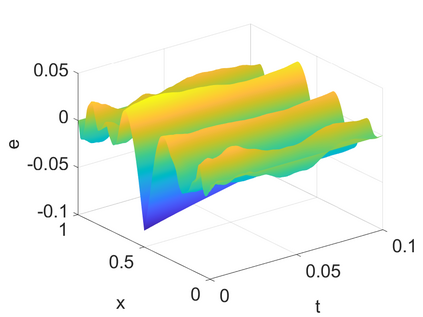
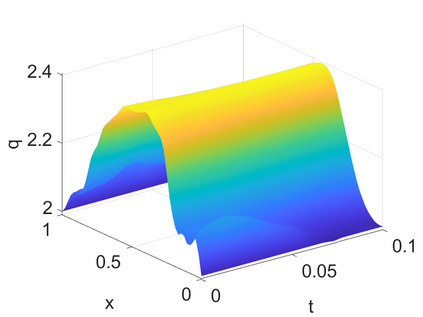
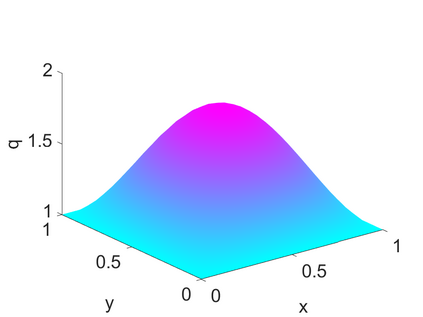
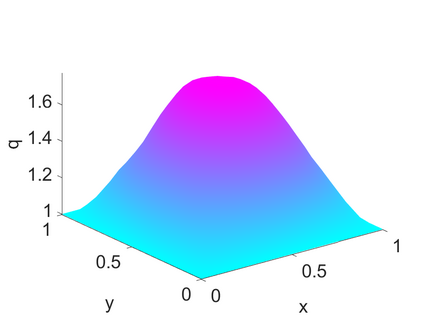
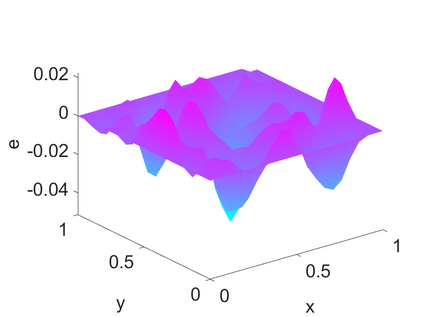
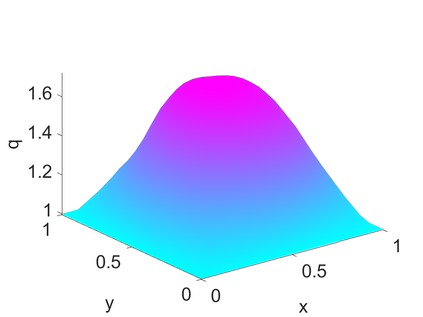
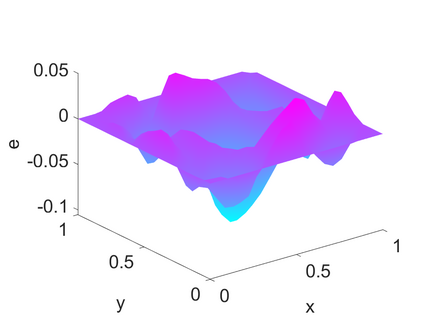
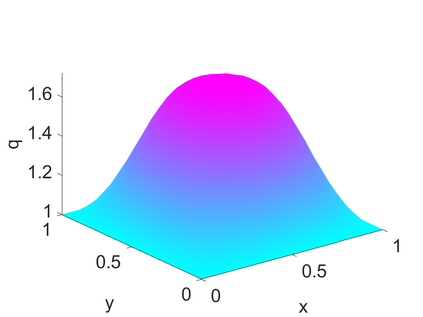
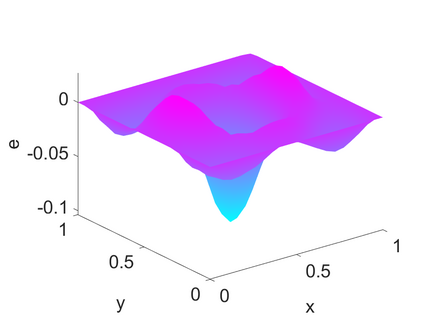
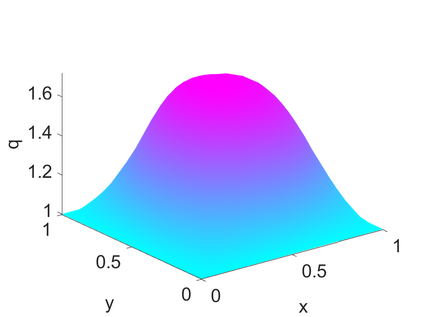
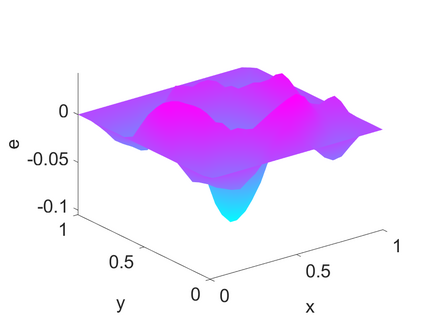
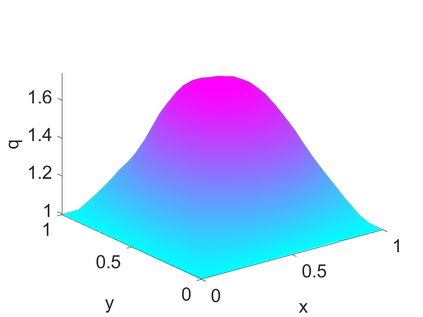
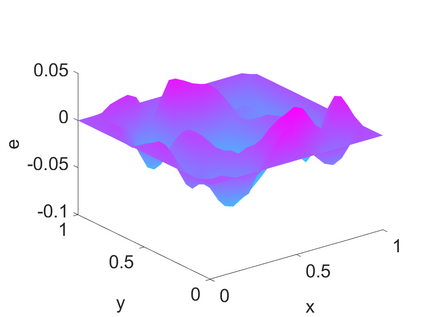
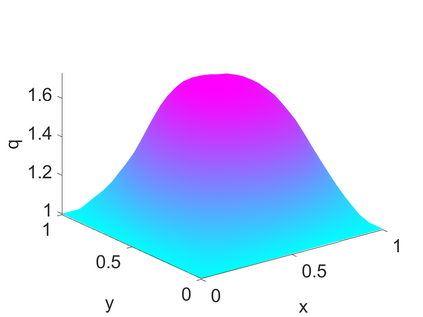
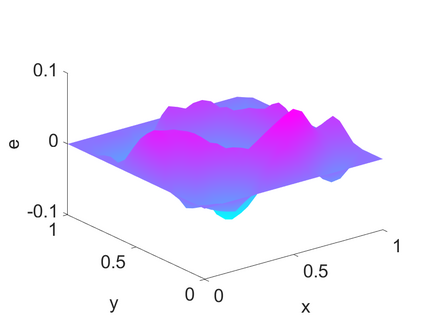
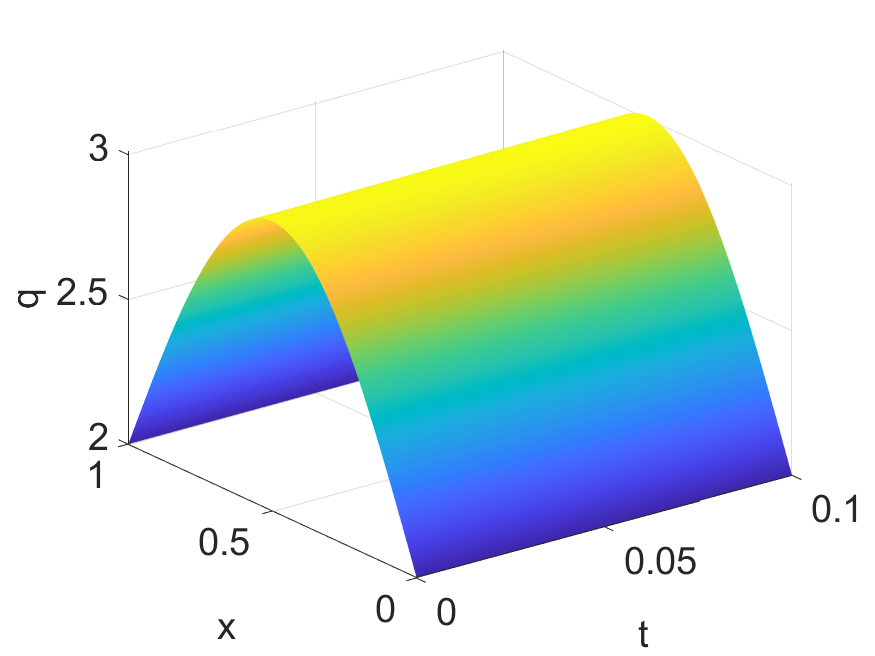
In this work, we study an inverse problem of recovering a space-time dependent diffusion coefficient in the subdiffusion model from the distributed observation, where the mathematical model involves a Djrbashian-Caputo fractional derivative of order $\alpha\in(0,1)$ in time. The main technical challenges of both theoretical and numerical analysis lie in the limited smoothing properties due to the fractional differential operator and the high degree of nonlinearity of the forward map from the unknown diffusion coefficient to the distributed observation. Theoretically, we establish two conditional stability results using a novel test function, which leads to a stability bound in $L^2(0,T;L^2(\Omega))$ under a suitable positivity condition. The positivity condition is verified for a large class of problem data. Numerically, we develop a rigorous procedure for the recovery of the diffusion coefficient based on a regularized least-squares formulation, which is then discretized by the standard Galerkin method with continuous piecewise linear elements in space and backward Euler convolution quadrature in time. We provide a complete error analysis of the fully discrete formulation, by combining several new error estimates for the direct problem (optimal in terms of data regularity), a discrete version of fractional maximal $L^p$ regularity, and a nonstandard energy argument. Under the positivity condition, we obtain a standard $L^2(0,T; L^2(\Omega))$ error estimate consistent with the conditional stability. Further, we illustrate the analysis with some numerical examples.