Recovery of the Order of Derivation for Fractional Diffusion Equations in an Unknown Medium

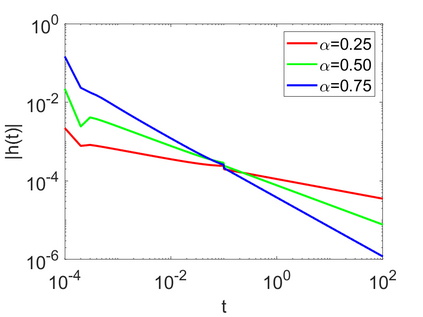
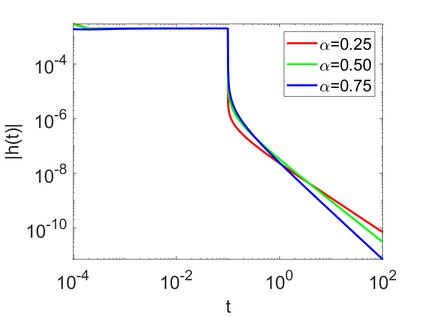
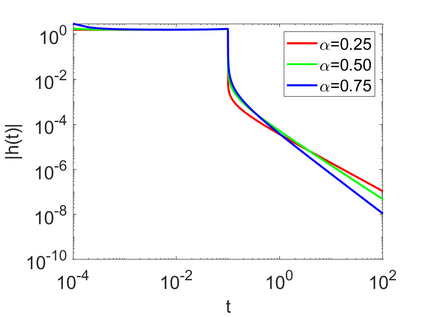
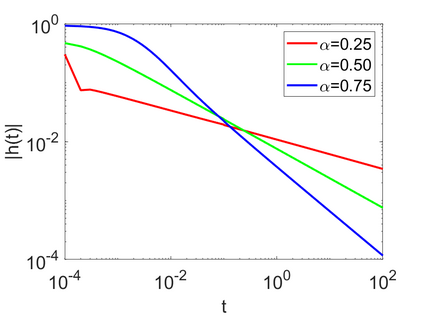
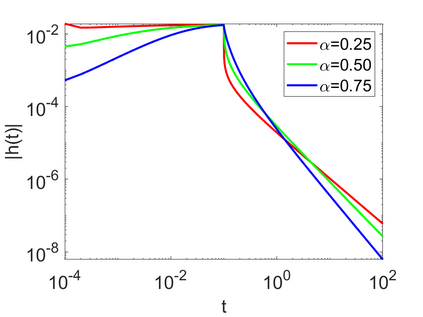
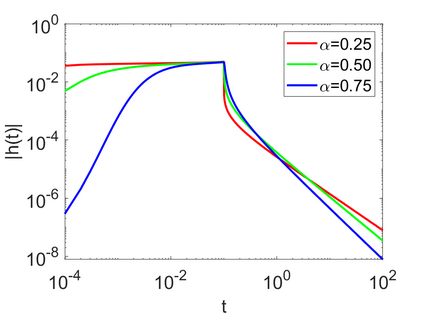
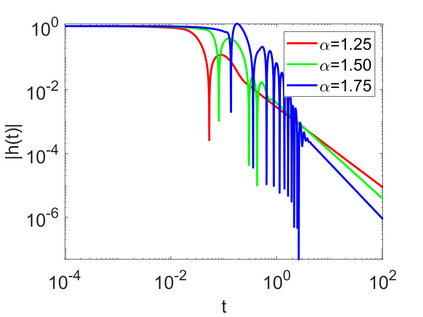
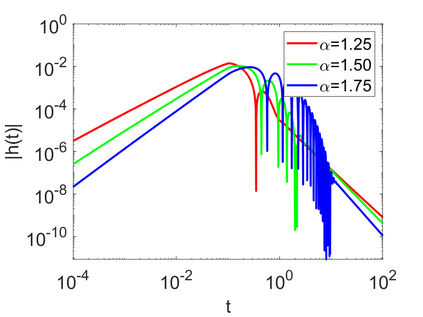
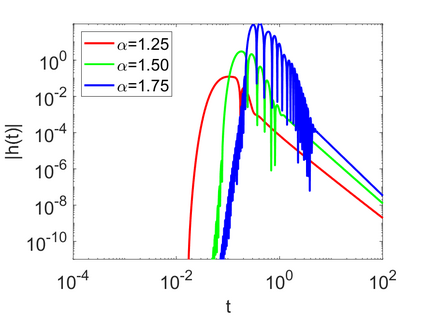
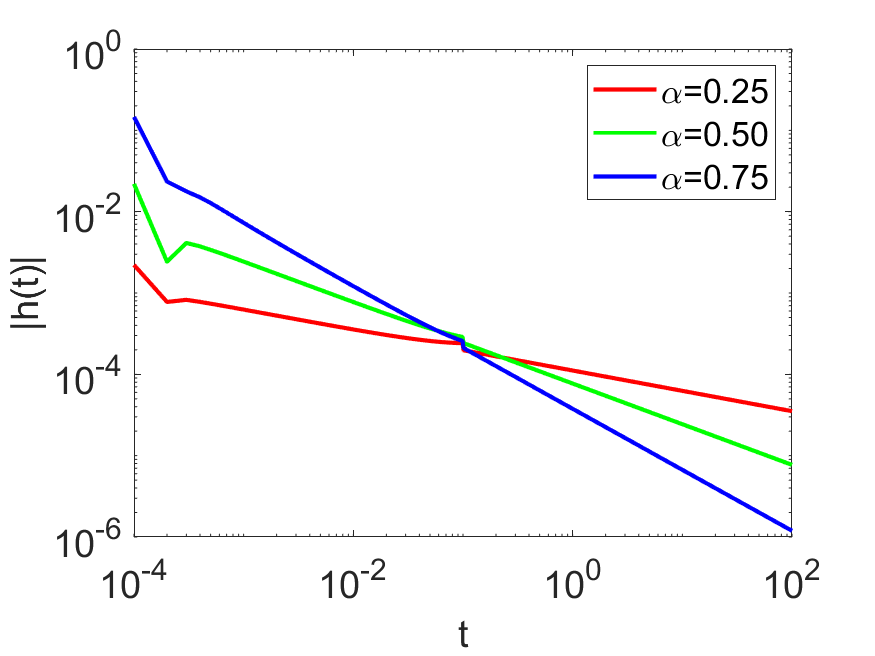
In this work, we investigate the recovery of a parameter in a diffusion process given by the order of derivation in time for a class of diffusion type equations, including both classical and time-fractional diffusion equations, from the flux measurement observed at one point on the boundary. The mathematical model for time-fractional diffusion equations involves a Djrbashian-Caputo fractional derivative in time. We prove a uniqueness result in an unknown medium (e.g., diffusion coefficients, obstacle, initial condition and source), i.e., the recovery of the order of derivation in a diffusion process having several pieces of unknown information. The proof relies on the analyticity of the solution at large time, asymptotic decay behavior, strong maximum principle of the elliptic problem and suitable application of the Hopf lemma. Further we provide an easy-to-implement reconstruction algorithm based on a nonlinear least-squares formulation, and several numerical experiments are presented to complement the theoretical analysis.