Risk Sensitive Portfolio Optimization with Regime-Switching and Default Contagion，香港理工大学应用数学系余翔助理教授，第八届全国社会媒体处理大会SMP2019

报告主题：Risk Sensitive Portfolio Optimization with Regime-Switching and Default Contagion

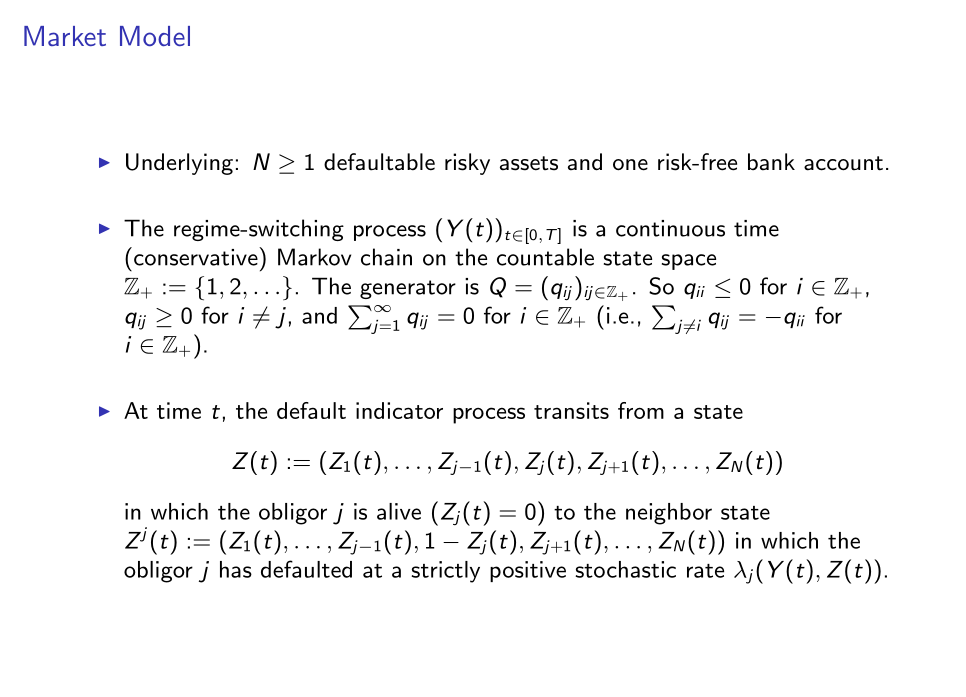
报告摘要：We study the dynamic risk-sensitive portfolio allocation in a regime-switching credit market with default contagion. The state space of the Markovian regime-switching process is assumed to be a countably infinite set. To characterize the value function of the risk sensitive stochastic control problem, we investigate the corresponding recursive infinite-dimensional nonlinear dynamical programming equations (DPEs) based on default states. We propose to work in the following procedure: Applying the theory of the monotone dynamical system, we first establish the existence and uniqueness of classical solutions to the recursive DPEs by a truncation argument in the finite state space. Moreover, the associated optimal feedback strategy is characterized by developing a rigorous verification theorem. Building upon results in the first stage, we construct a sequence of approximating risk sensitive control problems with finite state space and prove that the resulting smooth value functions will converge to the classical solution of the system of DPEs. The construction and approximation of the optimal feedback strategy for the original problem are thoroughly discussed. Some numerical results are also presented to illustrate our analytical conclusions. Joint work with Lijun Bo (USTC) and Huafu Liao (USTC).

嘉宾简介：余翔博士现任职于香港理工大学应用数学系助理教授。余博士2007年本科毕业于华中科技大学数学系信息与计算科学专业，并在2012年5月获得美国德州大学奥斯汀分校数学博士学位。 他的研究工作发表于 Annals of Applied Probability ， Mathematical Finance, SIAM Journal on Control and Optimization, Mathematics