Stable approximation of Helmholtz solutions by evanescent plane waves

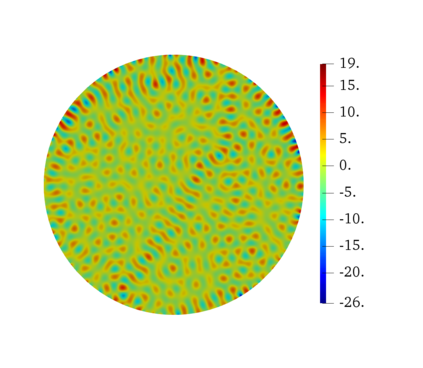
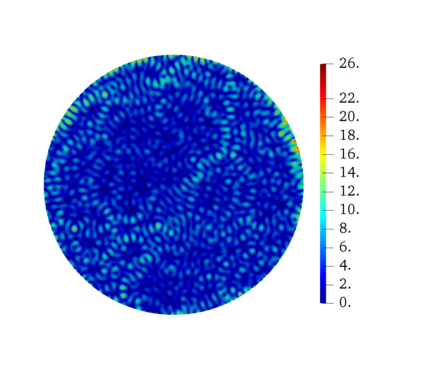
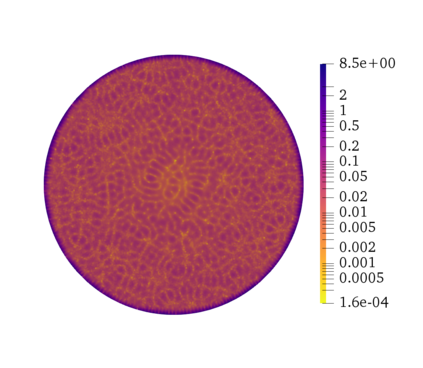
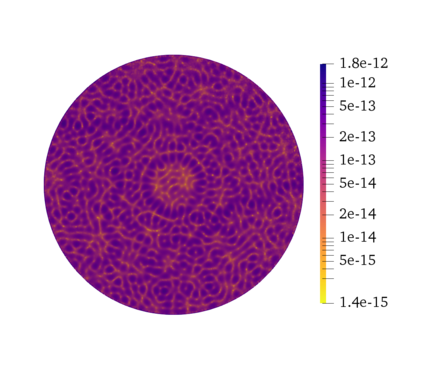
Solutions of the Helmholtz equation are known to be well approximated by superpositions of propagative plane waves. This observation is the foundation of successful Trefftz methods. However, when too many plane waves are used, the computation of the expansion is known to be numerically unstable. We prove that exponentially large coefficients in the expansion are unavoidable and we explain how this causes instability. In this work, we show that the Helmholtz solutions on a disk can be exactly represented by a continuous superposition of evanescent plane waves, generalizing the standard Herglotz representation. Here, by evanescent plane waves, we mean exponential plane waves with complex-valued propagation vector, whose absolute value decays exponentially in one direction. In addition, the density in this representation is proved to be uniformly bounded in a suitable weighted Lebesgue norm, hence overcoming the instability observed with propagative plane waves and paving the way for stable discrete expansions. In view of practical implementations, discretization strategies are investigated. We construct suitable finite-dimensional sets of evanescent plane waves using sampling strategies in a parametric domain. Provided one uses sufficient oversampling and regularization, numerical experiments show that the resulting approximations are both controllably accurate and numerically stable.