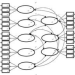
In this study, the combined use of structural equation modeling (SEM) and Bayesian network modeling (BNM) in causal inference analysis is revisited. The perspective highlights the debate between proponents of using BNM as either an exploratory phase or even as the sole phase in the definition of structural models, and those advocating for SEM as the superior alternative for exploratory analysis. The individual strengths and limitations of SEM and BNM are recognized, but this exploration evaluates the contention between utilizing SEM's robust structural inference capabilities and the dynamic probabilistic modeling offered by BNM. A case study of the work of, \citet{balaguer_2022} in a structural model for personal positive youth development (\textit{PYD}) as a function of positive parenting (\textit{PP}) and perception of the climate and functioning of the school (\textit{CFS}) is presented. The paper at last presents a clear stance on the analytical primacy of SEM in exploratory causal analysis, while acknowledging the potential of BNM in subsequent phases.
翻译:暂无翻译