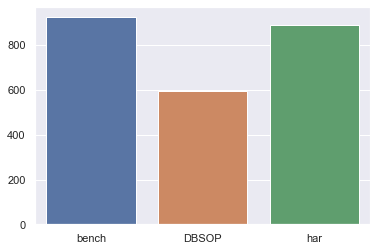
Markov Chain Monte Carlo (MCMC) techniques have long been studied in computational geometry subjects whereabouts the problems to be studied are complex geometric objects which by their nature require optimized techniques to be deployed or to gain useful insights by them. MCMC approaches are directly answering to geometric problems we are attempting to answer, and how these problems could be deployed from theory to practice. Polytope which is a limited volume in n-dimensional space specified by a collection of linear inequality constraints require specific approximation. Therefore, sampling across density based polytopes can not be performed without the use of such methods in which the amount of repetition required is defined as a property of error margin. In this work we propose a simple accurate sampling approach based on the triangulation (tessellation) of a polytope. Moreover, we propose an efficient algorithm named Density Based Sampling on Polytopes (DBSOP) for speedy MCMC sampling where the time required to perform sampling is significantly lower compared to existing approaches in low dimensions with complexity $\mathcal{O}^{*}\left(n^{3}\right)$. Ultimately, we highlight possible future aspects and how the proposed scheme can be further improved with the integration of reservoir-sampling based methods resulting in more speedy and efficient solution.
翻译:在计算几何主题中,已经对Markov链链蒙特卡洛(MCMC)技术进行了长期研究。 所研究的问题是复杂的几何物体,这些物体的性质要求部署最优化的技术或获得有用的见解。 MCMC方法直接回答了我们试图回答的几何问题,以及如何从理论到实践来处理这些问题。 由一系列线性不平等限制所指定的在正维空间中数量有限的聚合体,需要具体的近距离。 因此,如果不使用将所要求的重复程度界定为误差属性的方法,就无法进行基于密度的多面顶的取样。 在这项工作中,我们提出一个简单准确的抽样方法,其依据是多层的三角(相互连接)法。 此外,我们提议一种高效的算法,名为“基于多层取样法(DBSOP)”,用于快速进行MCMC取样所需的时间大大低于目前具有复杂性的低维度方法 $\mathcal{O ⁇ rev(n ⁇ 3 ⁇ right)$。 我们最后要强调一个基于多层三角(selation)的简单准确的抽样方法。 我们建议一个简单准确的抽样方法,其基础将如何使拟议的水库计划得到更迅速的整合。