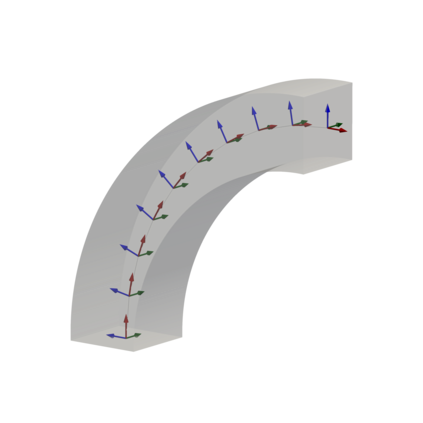
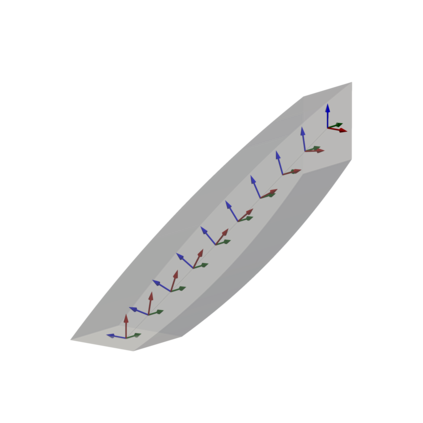
Based on more than three decades of rod finite element theory, this publication unifies all the successful contributions found in literature and eradicates the arising drawbacks like loss of objectivity, locking, path-dependence and redundant coordinates. Specifically, the idea of interpolating the nodal orientations using relative rotation vectors, proposed by Crisfield and Jeleni\'c in 1999, is extended to the interpolation of nodal Euclidean transformation matrices with the aid of relative twists; a strategy that arises from the SE(3)-structure of the Cosserat rod kinematics. Applying a Petrov-Galerkin projection method, we propose a novel rod finite element formulation where the virtual displacements and rotations as well as the translational and angular velocities are interpolated instead of using the consistent variations and time-derivatives of the introduced interpolation formula. Properties such as the intrinsic absence of locking, preservation of objectivity after discretization and parametrization in terms of a minimal number of nodal unknowns are demonstrated by conclusive numerical examples in both statics and dynamics.
翻译:根据30多年的棒子有限要素理论,本出版物统一了文献中发现的所有成功贡献,并消除了新出现的缺点,如丧失客观性、锁定、路径依赖和冗余坐标。具体地说,Crisfield和Jeleni\'c在1999年提出的利用相对旋转矢量对节点方向进行内插的想法,扩展至在相对扭曲的帮助下对诺达尔·欧几里得变矩阵的内插;这一战略产生于科塞拉特棒动因学的SE(3)结构。我们采用Petrov-Galerkin预测法,提出了一种新的棒子有限要素公式,即虚拟置换和旋转以及翻译和角速度的内插,而不是使用引入的内插方公式的一致变化和时间定型。静态和动态的决定性数字实例证明了内在缺乏锁定、在离散后保持客观性和在最低数目的无节点未知等属性。