Projective and Telescopic Projective Integration for Non-Linear Kinetic Mixtures

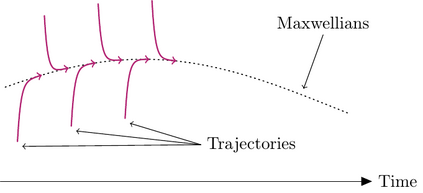
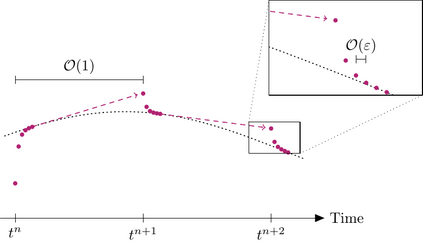
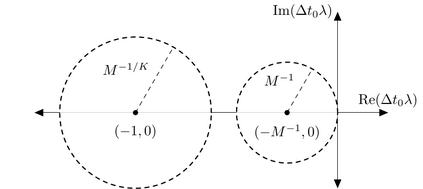
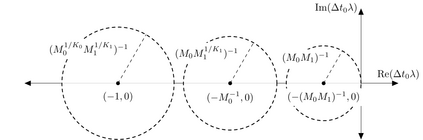
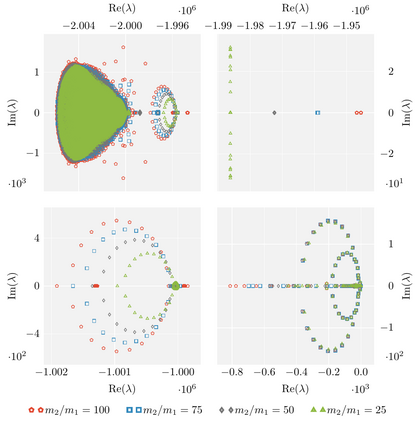
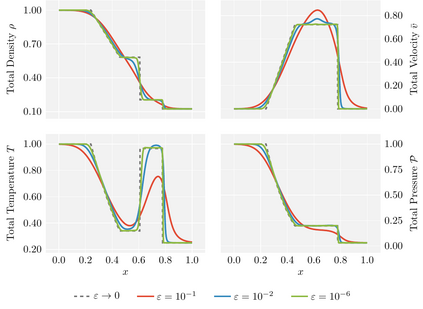
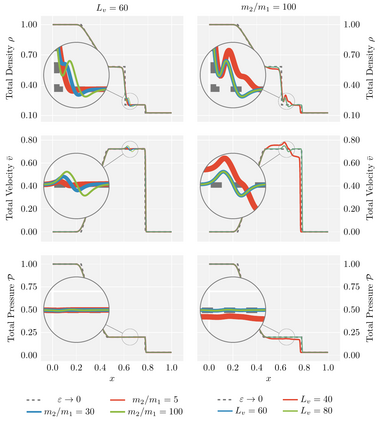
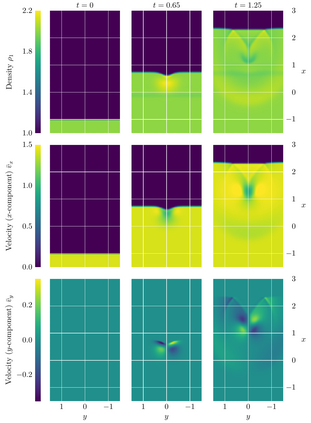
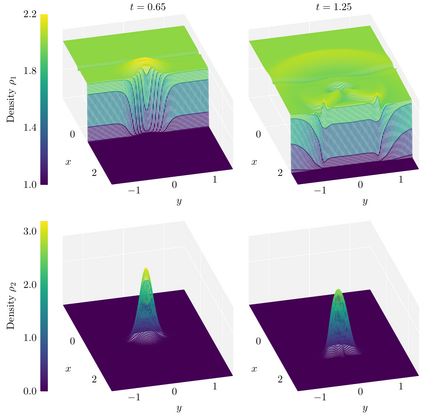
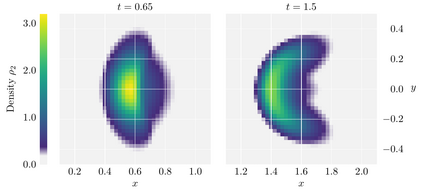
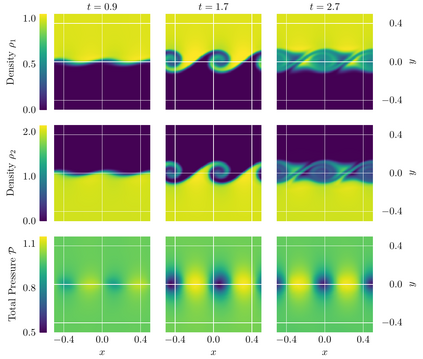
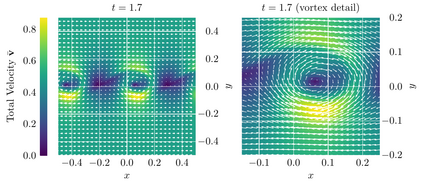
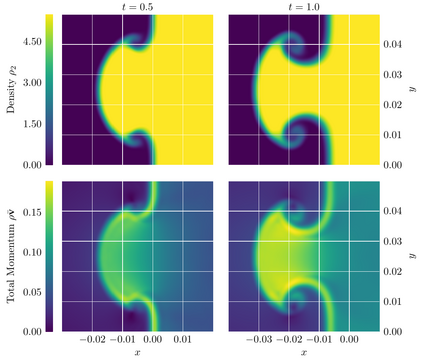
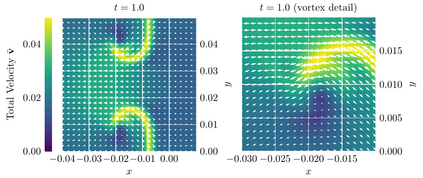
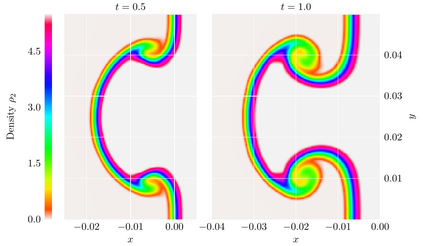
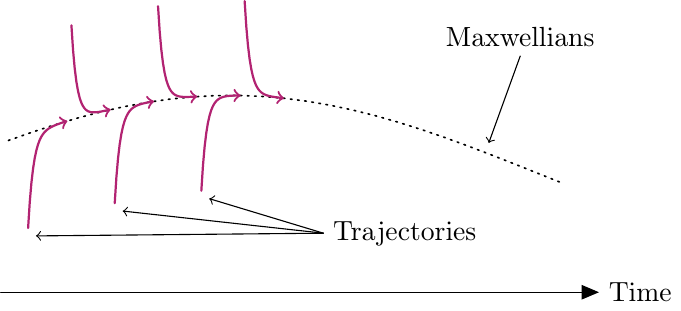
We propose fully explicit projective integration and telescopic projective integration schemes for the multispecies Boltzmann and \acf{BGK} equations. The methods employ a sequence of small forward-Euler steps, intercalated with large extrapolation steps. The telescopic approach repeats said extrapolations as the basis for an even larger step. This hierarchy renders the computational complexity of the method essentially independent of the stiffness of the problem, which permits the efficient solution of equations in the hyperbolic scaling with very small Knudsen numbers. We validate the schemes on a range of scenarios, demonstrating its prowess in dealing with extreme mass ratios, fluid instabilities, and other complex phenomena.