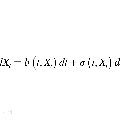几类扩散过程的逼近及应用

项目名称： 几类扩散过程的逼近及应用

项目编号： No.11471340

项目类型： 面上项目

立项/批准年度： 2015

项目学科： 数理科学和化学

项目作者： 任佳刚

作者单位： 中山大学

项目金额： 70万元

中文摘要： 本项目旨在研究几类扩散过程的逼近问题及其应用,包括：1.系数属于Holder空间的非马氏随机微分方程的Euler折线逼近的密度的收敛性与收敛速度的估计,并应用到利用Monte Carlo方法进行数值计算时的误差估计和方差缩减问题.为此将研究分数次Wiener泛函及Wiener-Poisson泛函的局部化及概率密度对于泛函的连续相依关系. 2.Brown运动驱动的法向反射随机微分方程在用光滑轨道所驱动的反射方程逼近时的收敛性及解的概率分布的支撑的刻画，以及该刻画在退化的二阶椭圆与抛物型偏微分方程的边界-内部极大值原理中的应用；推广该刻画到斜反射问题以及带跳的随机微分方程并应用到积分-偏微分方程的极大值原理. 3.反射随机微分方程及多值随机微分方程的挤压逼近的收敛性及在解的遍历性、不变测度的存在性、唯一性与正则性及Freidlin-Wentzell型大偏差原理等方面的应用.

中文关键词： Malliavin分析；随机微分方程；光滑逼近；欧拉逼近；挤压逼近

英文摘要： The aim of this project is to study the approximation problem of diffusion processes of several types, including: 1.The convergence and the estimation of convergence speed of densities of Euler scheme of non-Markov stochastic differential equations with coefficients in Holder spaces, and to the variance reduction problem in the numerical computation by Monte Carlo method. To this end we will study the localization of Wiener and Wiener-Poisson functionals of fractional order and the continuous dependence of their probability densities on the functionals. 2. Convergence of the approximating solutions of normally reflected stochastic differential equations driven by the Brownian motion by those of reflected differential equations driven by smooth paths, and the characterization of the support of densities of probability distributions of the solutions, and its application to the boundary-interior maximum principle of elliptic and parabolic partial differential equations of second order; extensions of this characterization to oblique reflection problems and stochastic differential equations equations driven by Levy processes and its application to the maximum principle for integro-partial differential equations; 3.Convergence of the penalization scheme for reflected stochastic differential equations and multivalued stochastic differential equations and its applications to the ergodicity of the solutions, existence,uniqueness and regularity of the invariant measures and the large deviation principle of Freidlin-Wentzell type.

英文关键词： Malliavin Calculus;stochastic differential equations;smooth approximation;Euler scheme;penalization scheme