Stabilized isogeometric formulation of the Stokes problem on overlapping patches

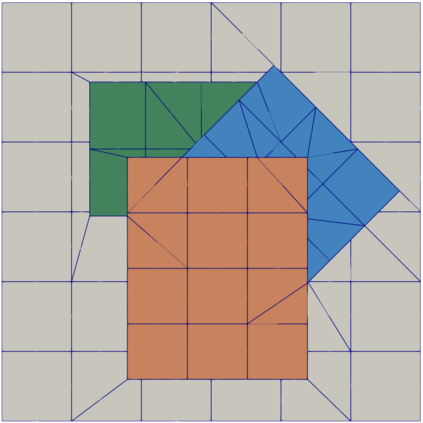
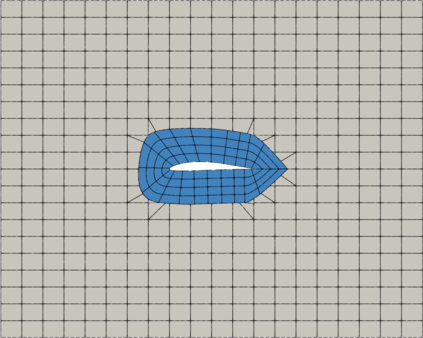
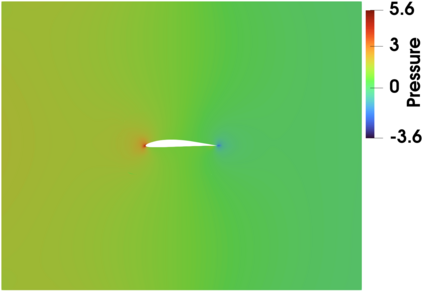
We present a novel stabilized isogeometric formulation for the Stokes problem, where the geometry of interest is obtained via overlapping NURBS (non-uniform rational B-spline) patches, i.e., one patch on top of another in an arbitrary but predefined hierarchical order. All the visible regions constitute the computational domain, whereas independent patches are coupled through visible interfaces using Nitsche's formulation. Such a geometric representation inevitably involves trimming, which may yield trimmed elements of extremely small measures (referred to as bad elements) and thus lead to the instability issue. Motivated by the minimal stabilization method that rigorously guarantees stability for trimmed geometries [1], in this work we generalize it to the Stokes problem on overlapping patches. Central to our method is the distinct treatments for the pressure and velocity spaces: Stabilization for velocity is carried out for the flux terms on interfaces, whereas pressure is stabilized in all the bad elements. We provide a priori error estimates with a comprehensive theoretical study. Through a suite of numerical tests, we first show that optimal convergence rates are achieved, which consistently agrees with our theoretical findings. Second, we show that the accuracy of pressure is significantly improved by several orders using the proposed stabilization method, compared to the results without stabilization. Finally, we also demonstrate the flexibility and efficiency of the proposed method in capturing local features in the solution field.