A Framework for Characterising the Value of Information in Hidden Markov Models

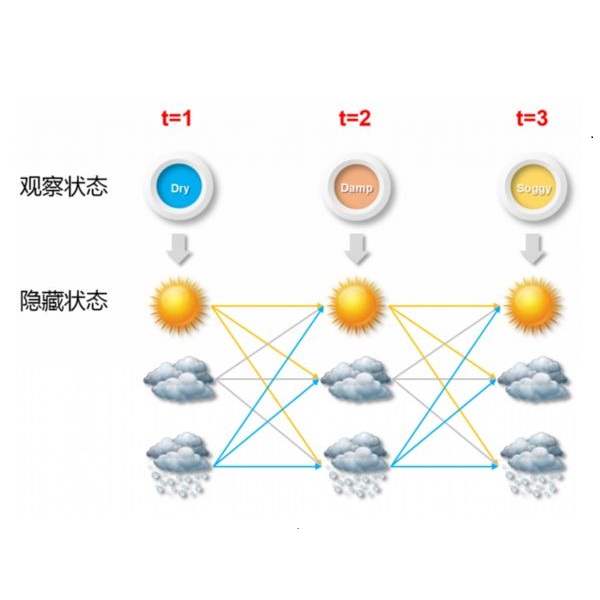
In this paper, a general framework is formalised to characterise the value of information (VoI) in hidden Markov models. Specifically, the VoI is defined as the mutual information between the current, unobserved status at the source and a sequence of observed measurements at the receiver, which can be interpreted as the reduction in the uncertainty of the current status given that we have noisy past observations of a hidden Markov process. We explore the VoI in the context of the noisy Ornstein-Uhlenbeck process and derive its closed-form expressions. Moreover, we investigate the effect of different sampling policies on VoI, deriving simplified expressions in different noise regimes and analysing statistical properties of the VoI in the worst case. We also study the optimal sampling policy to maximise the average information value under the sampling rate constraint. In simulations, the validity of theoretical results is verified, and the performance of VoI in Markov and hidden Markov models is also analysed. Numerical results further illustrate that the proposed VoI framework can support timely transmission in status update systems, and it can also capture the correlation properties of the underlying random process and the noise in the transmission environment.