Parameterized Verification of Disjunctive Timed Networks

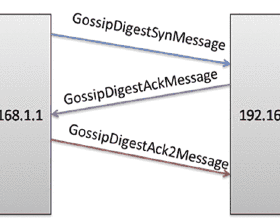
We introduce new techniques for the parameterized verification of disjunctive timed networks (DTNs), i.e., networks of timed automata (TAs) that communicate via location guards that enable a transition only if at least one process is in a given location. This computational model has been considered in the literature before, and example applications are gossiping clock synchronization protocols or planning problems. We address the minimum-time reachability problem (minreach) in DTNs, and show how to efficiently solve it based on a novel zone-graph algorithm. We further show that solving minreach allows us to construct a summary TA capturing exactly the possible behaviors of a single TA within a DTN of arbitrary size. The combination of these two results enables the parameterized verification of DTNs, while avoiding the construction of an exponential-size cutoff-system required by existing results. Our techniques are also implemented, and experiments show their practicality.