蛋团结合时压缩的QMC量和表面集成</s> (Compressed QMC volume and surface integration on union of balls) - 专知论文

会员服务 ·

0

Integration · Microsoft Surface · MATLAB · TOOLS · 线性的 ·

2023 年 3 月 2 日

Compressed QMC volume and surface integration on union of balls

翻译：蛋团结合时压缩的QMC量和表面集成

Giacomo Elefante,Alvise Sommariva,Marco Vianello

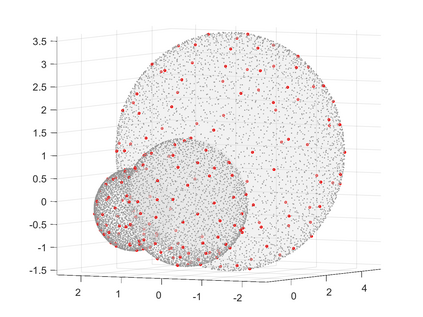
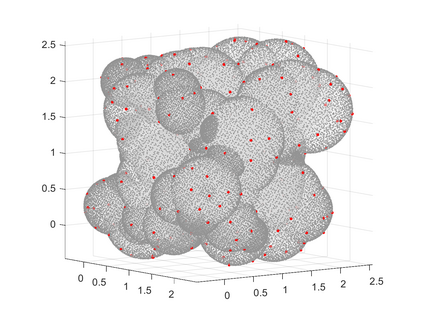
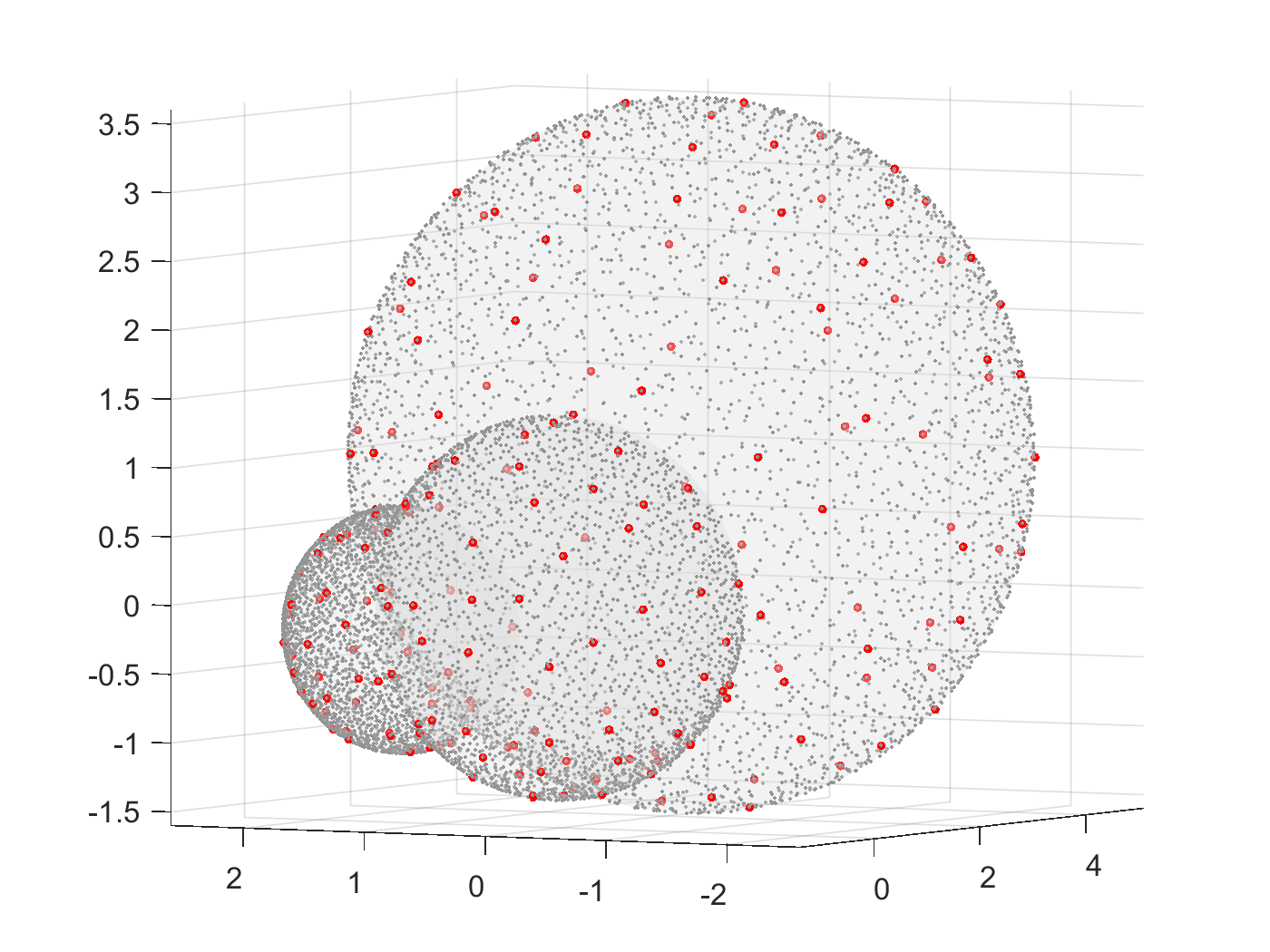
We discuss an algorithm for Tchakaloff-like compression of Quasi-MonteCarlo (QMC) volume/surface integration on union of balls (multibubbles). The key tools are Davis-Wilhelmsen theorem on the so-called Tchakaloff sets for positive linear functionals on polynomial spaces, and Lawson-Hanson algorithm for NNLS. We provide the corresponding Matlab package together with several examples.

翻译：我们讨论“卡西-蒙特卡洛(QMC)”(Quasi-MonteCarlo)体积/地表结合(多泡泡)的算法。关键工具是所谓的“Tchakaloff ” 模拟多糖空间正线功能的Davis-Wilhelmsen定理和“NNLLS”的Lawson-Hanson算法。我们提供了相应的“Matlab”软件包以及几个例子。</s>

0

相关内容

Integration

Integration：Integration, the VLSI Journal。 Explanation：集成，VLSI杂志。 Publisher：Elsevier。 SIT：http://dblp.uni-trier.de/db/journals/integration/

【NUS-Xavier 教授】图神经网络应用概述，15页ppt

专知会员服务

50+阅读 · 2021年6月30日

【ETH】最新《几何数据分析》2020课程，附PPT下载

专知会员服务

42+阅读 · 2020年12月18日

INRIA 最新《机器学习理论》课程笔记，176页pdf

专知会员服务

50+阅读 · 2020年12月14日

神经常微分方程教程，50页ppt，A brief tutorial on Neural ODEs

神经常微分方程教程，50页ppt，A brief tutorial on Neural ODEs

专知会员服务

71+阅读 · 2020年8月2日

图像分类技巧集，17页ppt《Bag of Tricks for Image Classification》

图像分类技巧集，17页ppt《Bag of Tricks for Image Classification》

专知会员服务

94+阅读 · 2020年3月12日

【机器学习基础最新版】（Mathematics for Machine Learning），417页pdf

【机器学习基础最新版】（Mathematics for Machine Learning），417页pdf

专知会员服务

239+阅读 · 2019年10月21日

Connections between Support Vector Machines, Wasserstein distance and gradient-penalty GANs

Connections between Support Vector Machines, Wasserstein distance and gradient-penalty GANs

专知会员服务

34+阅读 · 2019年10月17日

Deep Learning Based Detection and Correction of Cardiac MR Motion Artefacts During Reconstruction for High-Quality Segmentation

Deep Learning Based Detection and Correction of Cardiac MR Motion Artefacts During Reconstruction for High-Quality Segmentation

专知会员服务

56+阅读 · 2019年10月17日

强化学习最新教程，17页pdf

强化学习最新教程，17页pdf

专知会员服务

174+阅读 · 2019年10月11日

【人工智能在2019：一年回顾】反人工智能，AI in 2019: A Year in Review

【人工智能在2019：一年回顾】反人工智能，AI in 2019: A Year in Review

专知会员服务

79+阅读 · 2019年10月10日

VCIP 2022 Call for Demos

VCIP 2022 Call for Demos

CCF多媒体专委会

1+阅读 · 2022年6月6日

征稿 | International Joint Conference on Knowledge Graphs (IJCKG)

征稿 | International Joint Conference on Knowledge Graphs (IJCKG)

开放知识图谱

2+阅读 · 2022年5月20日

局部学习的特征选择：Local-Learning-Based Feature Selection

局部学习的特征选择：Local-Learning-Based Feature Selection

我爱读PAMI

14+阅读 · 2019年9月20日

Hierarchically Structured Meta-learning

Hierarchically Structured Meta-learning

CreateAMind

26+阅读 · 2019年5月22日

Transferring Knowledge across Learning Processes

Transferring Knowledge across Learning Processes

CreateAMind

27+阅读 · 2019年5月18日

深度自进化聚类：Deep Self-Evolution Clustering

深度自进化聚类：Deep Self-Evolution Clustering

我爱读PAMI

15+阅读 · 2019年4月13日

R工程化—Rest API 之plumber包

R工程化—Rest API 之plumber包

R语言中文社区

11+阅读 · 2018年12月25日

A Technical Overview of AI & ML in 2018 & Trends for 2019

A Technical Overview of AI & ML in 2018 & Trends for 2019

待字闺中

16+阅读 · 2018年12月24日

disentangled-representation-papers

disentangled-representation-papers

CreateAMind

26+阅读 · 2018年9月12日

【论文】变分推断（Variational inference)的总结

【论文】变分推断（Variational inference)的总结

机器学习研究会

39+阅读 · 2017年11月16日

两类带导数的非线性Schrodinger方程拟周期解的存在性

国家自然科学基金

0+阅读 · 2015年12月31日

Poisson流形上的修正Hamilton方法

国家自然科学基金

0+阅读 · 2014年12月31日

具有临界指数的Schrodinger-Poisson系统的解

国家自然科学基金

0+阅读 · 2013年12月31日

拟南芥DIF（DRIP1-Interacting Factor）在胁迫信号应答中的功能分析

国家自然科学基金

0+阅读 · 2012年12月31日

Cocycle动力学和拟周期薛定谔算子的谱

国家自然科学基金

0+阅读 · 2012年12月31日

三维流形上的Heegaard分解及其在纽结理论中应用

国家自然科学基金

0+阅读 · 2011年12月31日

遍历哈密顿系统的谱理论

国家自然科学基金

0+阅读 · 2009年12月31日

约化群酉表示的branching law及其应用

国家自然科学基金

0+阅读 · 2009年12月31日

Mather理论与Hamilton系统的不稳定性

国家自然科学基金

0+阅读 · 2008年12月31日

仿射流形上的非线性分析

国家自然科学基金

0+阅读 · 2008年12月31日

ExCalibR: Expected Calibration of Recommendations

Arxiv

0+阅读 · 2023年4月24日

Ordinal time series analysis with the R package otsfeatures

Ordinal time series analysis with the R package otsfeatures

Arxiv

0+阅读 · 2023年4月24日

Fuzzy clustering of ordinal time series based on two novel distances with economic applications

Arxiv

0+阅读 · 2023年4月24日

On the Performance of a Novel Class of Linear System Solvers and Comparison with State-of-The-Art Algorithms

Arxiv

0+阅读 · 2023年4月24日

Compressed sensing with l0-norm: statistical physics analysis and algorithms for signal recovery

Arxiv

0+阅读 · 2023年4月24日

TransPimLib: A Library for Efficient Transcendental Functions on Processing-in-Memory Systems

Arxiv

0+阅读 · 2023年4月23日

Local Bounded Commuting Projection Operators for Discrete Gradgrad Complexes

Arxiv

0+阅读 · 2023年4月23日

Computing the optimal error exponential function for fixed-length lossy coding in discrete memoryless sources

Arxiv

0+阅读 · 2023年4月23日

Intersection Queries for Flat Semi-Algebraic Objects in Three Dimensions and Related Problems

Arxiv

0+阅读 · 2023年4月23日

Under-Approximate Reachability Analysis for a Class of Linear Systems with Inputs

Arxiv

0+阅读 · 2023年4月20日

VIP会员

文章信息

相关主题

Microsoft Surface

相关VIP内容

【NUS-Xavier 教授】图神经网络应用概述，15页ppt

专知会员服务

50+阅读 · 2021年6月30日

【ETH】最新《几何数据分析》2020课程，附PPT下载

专知会员服务

42+阅读 · 2020年12月18日

INRIA 最新《机器学习理论》课程笔记，176页pdf

专知会员服务

50+阅读 · 2020年12月14日

神经常微分方程教程，50页ppt，A brief tutorial on Neural ODEs

神经常微分方程教程，50页ppt，A brief tutorial on Neural ODEs

专知会员服务

71+阅读 · 2020年8月2日

图像分类技巧集，17页ppt《Bag of Tricks for Image Classification》

图像分类技巧集，17页ppt《Bag of Tricks for Image Classification》

专知会员服务

94+阅读 · 2020年3月12日

【机器学习基础最新版】（Mathematics for Machine Learning），417页pdf

【机器学习基础最新版】（Mathematics for Machine Learning），417页pdf

专知会员服务

239+阅读 · 2019年10月21日

Connections between Support Vector Machines, Wasserstein distance and gradient-penalty GANs

Connections between Support Vector Machines, Wasserstein distance and gradient-penalty GANs

专知会员服务

34+阅读 · 2019年10月17日

Deep Learning Based Detection and Correction of Cardiac MR Motion Artefacts During Reconstruction for High-Quality Segmentation

Deep Learning Based Detection and Correction of Cardiac MR Motion Artefacts During Reconstruction for High-Quality Segmentation

专知会员服务

56+阅读 · 2019年10月17日

强化学习最新教程，17页pdf

强化学习最新教程，17页pdf

专知会员服务

174+阅读 · 2019年10月11日

【人工智能在2019：一年回顾】反人工智能，AI in 2019: A Year in Review

【人工智能在2019：一年回顾】反人工智能，AI in 2019: A Year in Review

专知会员服务

79+阅读 · 2019年10月10日

热门VIP内容

相关资讯

VCIP 2022 Call for Demos

VCIP 2022 Call for Demos

CCF多媒体专委会

1+阅读 · 2022年6月6日

征稿 | International Joint Conference on Knowledge Graphs (IJCKG)

征稿 | International Joint Conference on Knowledge Graphs (IJCKG)

开放知识图谱

2+阅读 · 2022年5月20日

局部学习的特征选择：Local-Learning-Based Feature Selection

局部学习的特征选择：Local-Learning-Based Feature Selection

我爱读PAMI

14+阅读 · 2019年9月20日

Hierarchically Structured Meta-learning

Hierarchically Structured Meta-learning

CreateAMind

26+阅读 · 2019年5月22日

Transferring Knowledge across Learning Processes

Transferring Knowledge across Learning Processes

CreateAMind

27+阅读 · 2019年5月18日

深度自进化聚类：Deep Self-Evolution Clustering

深度自进化聚类：Deep Self-Evolution Clustering

我爱读PAMI

15+阅读 · 2019年4月13日

R工程化—Rest API 之plumber包

R工程化—Rest API 之plumber包

R语言中文社区

11+阅读 · 2018年12月25日

A Technical Overview of AI & ML in 2018 & Trends for 2019

A Technical Overview of AI & ML in 2018 & Trends for 2019

待字闺中

16+阅读 · 2018年12月24日

disentangled-representation-papers

disentangled-representation-papers

CreateAMind

26+阅读 · 2018年9月12日

【论文】变分推断（Variational inference)的总结

【论文】变分推断（Variational inference)的总结

机器学习研究会

39+阅读 · 2017年11月16日

相关论文

ExCalibR: Expected Calibration of Recommendations

Arxiv

0+阅读 · 2023年4月24日

Ordinal time series analysis with the R package otsfeatures

Ordinal time series analysis with the R package otsfeatures

Arxiv

0+阅读 · 2023年4月24日

Fuzzy clustering of ordinal time series based on two novel distances with economic applications

Arxiv

0+阅读 · 2023年4月24日

On the Performance of a Novel Class of Linear System Solvers and Comparison with State-of-The-Art Algorithms

Arxiv

0+阅读 · 2023年4月24日

Compressed sensing with l0-norm: statistical physics analysis and algorithms for signal recovery

Arxiv

0+阅读 · 2023年4月24日

TransPimLib: A Library for Efficient Transcendental Functions on Processing-in-Memory Systems

Arxiv

0+阅读 · 2023年4月23日

Local Bounded Commuting Projection Operators for Discrete Gradgrad Complexes

Arxiv

0+阅读 · 2023年4月23日

Computing the optimal error exponential function for fixed-length lossy coding in discrete memoryless sources

Arxiv

0+阅读 · 2023年4月23日

Intersection Queries for Flat Semi-Algebraic Objects in Three Dimensions and Related Problems

Arxiv

0+阅读 · 2023年4月23日

Under-Approximate Reachability Analysis for a Class of Linear Systems with Inputs

Arxiv

0+阅读 · 2023年4月20日

相关基金

两类带导数的非线性Schrodinger方程拟周期解的存在性

国家自然科学基金

0+阅读 · 2015年12月31日

Poisson流形上的修正Hamilton方法

国家自然科学基金

0+阅读 · 2014年12月31日

具有临界指数的Schrodinger-Poisson系统的解

国家自然科学基金

0+阅读 · 2013年12月31日

拟南芥DIF（DRIP1-Interacting Factor）在胁迫信号应答中的功能分析

国家自然科学基金

0+阅读 · 2012年12月31日

Cocycle动力学和拟周期薛定谔算子的谱

国家自然科学基金

0+阅读 · 2012年12月31日

三维流形上的Heegaard分解及其在纽结理论中应用

国家自然科学基金

0+阅读 · 2011年12月31日

遍历哈密顿系统的谱理论

国家自然科学基金

0+阅读 · 2009年12月31日

约化群酉表示的branching law及其应用

国家自然科学基金

0+阅读 · 2009年12月31日

Mather理论与Hamilton系统的不稳定性

国家自然科学基金

0+阅读 · 2008年12月31日

仿射流形上的非线性分析

国家自然科学基金

0+阅读 · 2008年12月31日

微信扫码咨询专知VIP会员