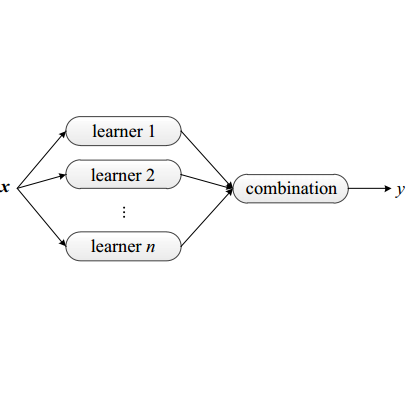
Math word problems (MWPs) require analyzing text descriptions and generating mathematical equations to derive solutions. Existing works focus on solving MWPs with two types of solvers: tree-based solver and large language model (LLM) solver. However, these approaches always solve MWPs by a single solver, which will bring the following problems: (1) Single type of solver is hard to solve all types of MWPs well. (2) A single solver will result in poor performance due to over-fitting. To address these challenges, this paper utilizes multiple ensemble approaches to improve MWP-solving ability. Firstly, We propose a problem type classifier that combines the strengths of the tree-based solver and the LLM solver. This ensemble approach leverages their respective advantages and broadens the range of MWPs that can be solved. Furthermore, we also apply ensemble techniques to both tree-based solver and LLM solver to improve their performance. For the tree-based solver, we propose an ensemble learning framework based on ten-fold cross-validation and voting mechanism. In the LLM solver, we adopt self-consistency (SC) method to improve answer selection. Experimental results demonstrate the effectiveness of these ensemble approaches in enhancing MWP-solving ability. The comprehensive evaluation showcases improved performance, validating the advantages of our proposed approach. Our code is available at this url: https://github.com/zhouzihao501/NLPCC2023-Shared-Task3-ChineseMWP.
翻译:暂无翻译