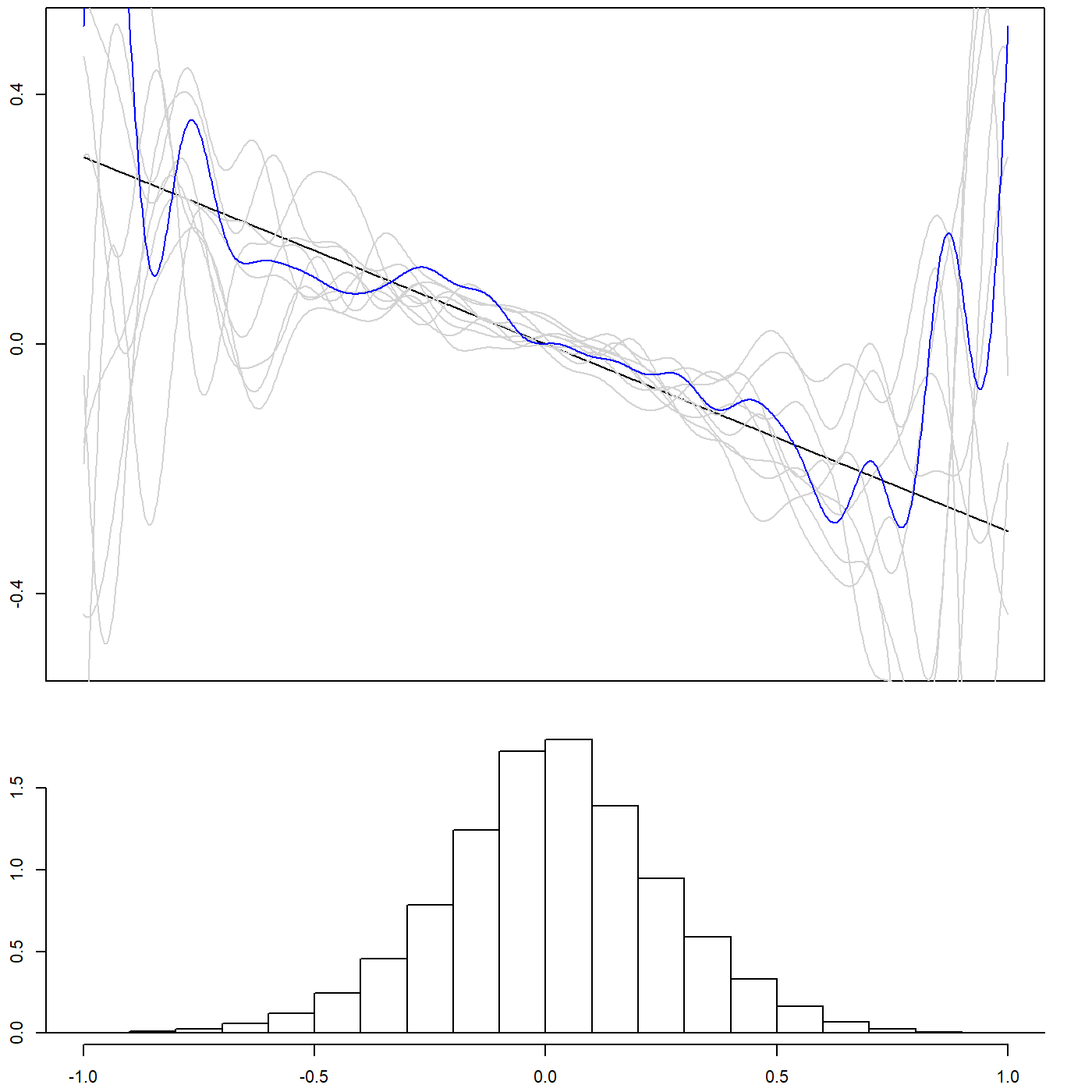
Nonparametric calibration for stochastic reaction-diffusion equations based on discrete observations

Nonparametric estimation for semilinear SPDEs, namely stochastic reaction-diffusion equations in one space dimension, is studied. We consider observations of the solution field on a discrete grid in time and space with infill asymptotics in both coordinates. Firstly, based on a precise analysis of the H\"older regularity of the solution process and its nonlinear component, we show that the asymptotic properties of diffusivity and volatility estimators derived from realized quadratic variations in the linear setup generalize to the semilinear SPDE. In particular, we obtain a rate-optimal joint estimator of the two parameters. Secondly, we derive a nonparametric estimator for the reaction function specifying the underlying equation. The estimate is chosen from a finite-dimensional function space based on a simple least squares criterion. Oracle inequalities with respect to both the empirical and usual $L^2$-risk provide conditions for the estimator to achieve the usual nonparametric rate of convergence. Adaptivity is provided via model selection.