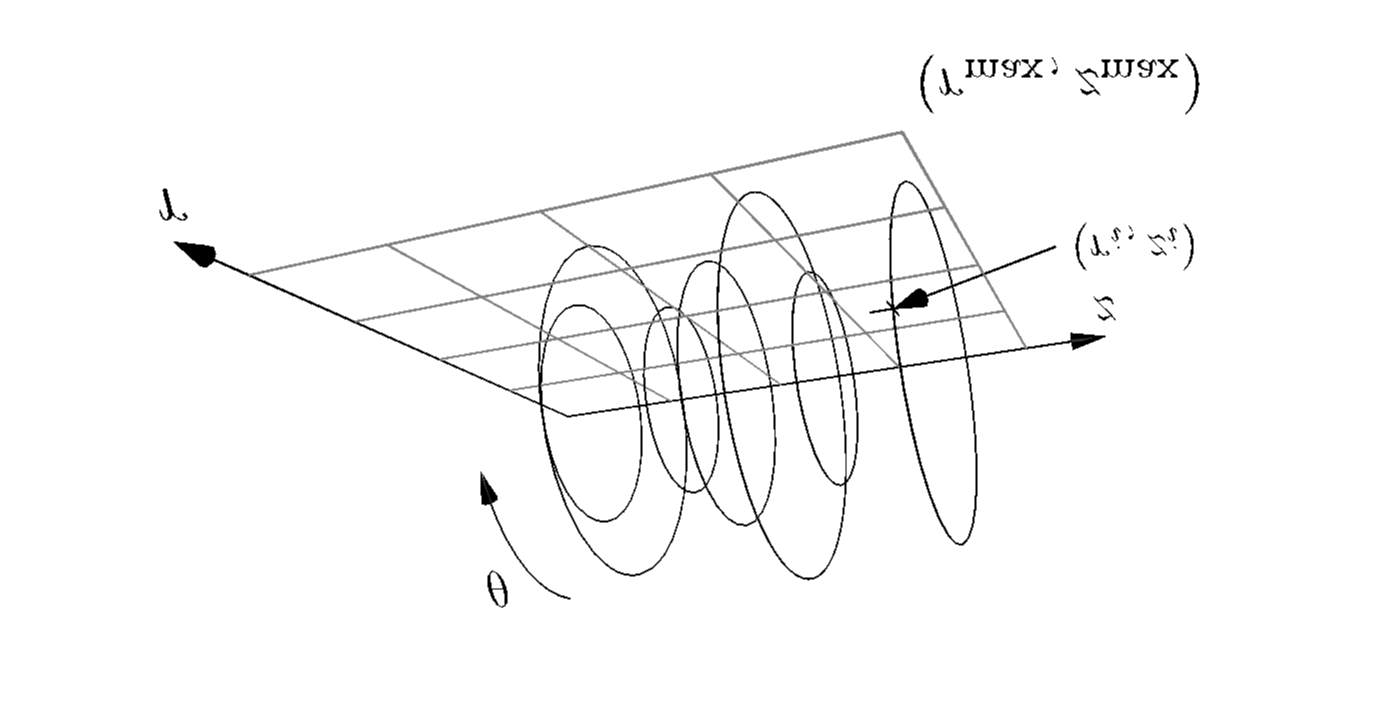
The Fast Multipole Method (FMM) for the Poisson equation is extended to the case of non-axisymmetric problems in an axisymmetric domain, described by cylindrical coordinates. The method is based on a Fourier decomposition of the source into a modal expansion and the evaluation of the corresponding modes of the field using a two-dimensional tree decomposition in the radial and axial coordinate. The field coefficients are evaluated using a modal Green's function which can be evaluated using well-known recursions for the Legendre function of the second kind, and whose derivatives can be found recursively using the Laplace equation in cylindrical coordinates. The principal difference between the cylindrical and Cartesian problems is the lack of translation invariance in the evaluation of local interactions, leading to an increase in computational effort for the axisymmetric domain. Results are presented for solution accuracy and convergence and for computation time compared to direct evaluation. The method is found to converge well, with ten digit accuracy being achieved for the test cases presented. Computation time is controlled by the balance between initialization and the evaluation of local interactions between source and field points, and is about two orders of magnitude less than that required for direct evaluation, depending on expansion order.
翻译:Poisson 方程式的快速多极法(FMM) 扩展至以圆柱体坐标描述的轴对称域的非轴对称问题,该方法基于源的Fourier分解成模式扩展,以及使用辐射和轴坐标二维树分解对相应字段模式的评价。使用模型格林的函数对外地系数进行评估,该函数可以使用第二类传说函数众所周知的回溯来评估,其衍生物可以在圆柱体坐标中利用拉比方方对等相反复发现。圆柱体和Cartesian问题的主要区别是,在评价当地互动时缺乏变异性,导致增加对轴线坐标域的计算努力。对解决办法的准确性和趋同性以及计算时间与直接评价相比较的结果,发现该方法十分一致,对提出的测试案例实现了十位准确性,其衍生物可以在圆柱体坐标坐标中反复发现。对圆柱体和Cartesa 问题的主要区别在于,在评估的初始来源与实地评估之间,对实地评估的顺序之间的平衡程度,对实地评估需要的顺序和两个直径的顺序进行了较慢的控制。