Euler scheme for approximation of solution of nonlinear ODEs under inexact information

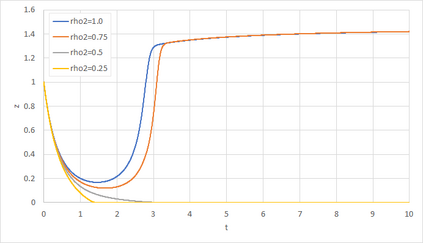
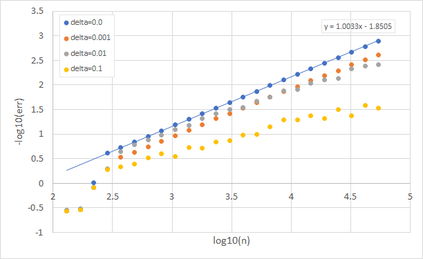
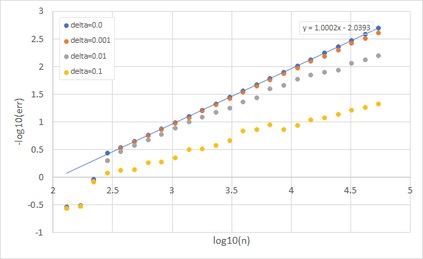
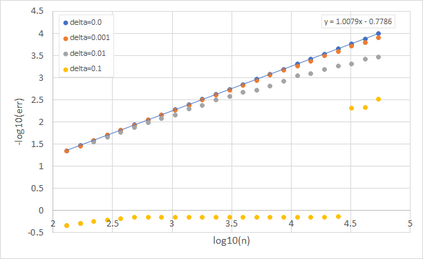
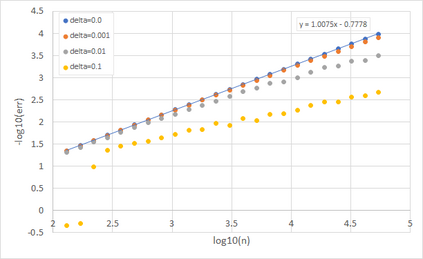
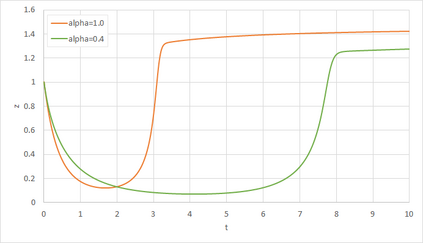
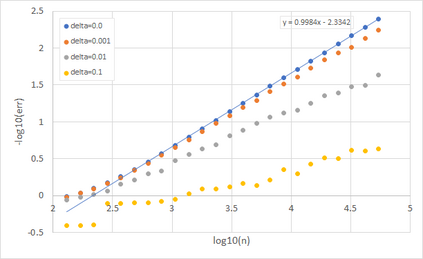
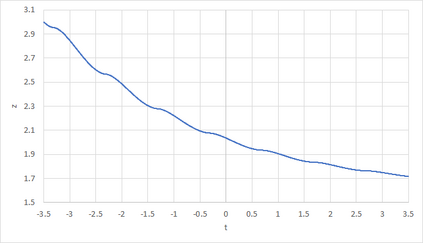
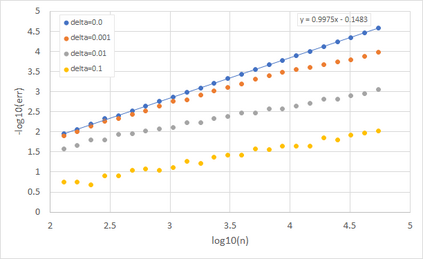
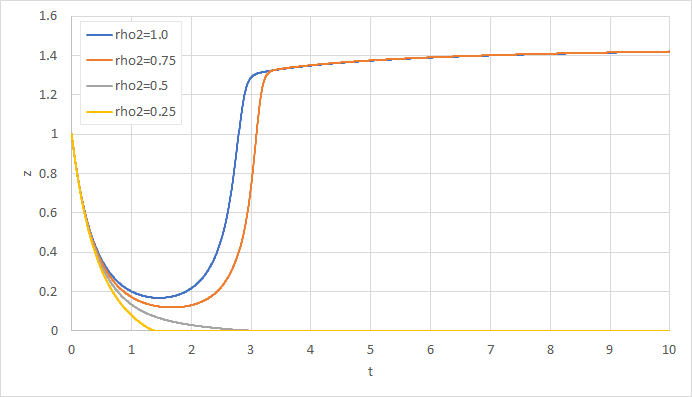
We investigate error of the Euler scheme in the case when the right-hand side function of the underlying ODE satisfies nonstandard assumptions such as local one-side Lipschitz condition and local H\"older continuity. Moreover, we assume two cases in regards to information availability: exact and noisy with respect to the right-hand side function. Optimality analysis of the Euler scheme is also provided. Lastly, we present the results of some numerical experiments.