Projected distances for multi-parameter persistence modules

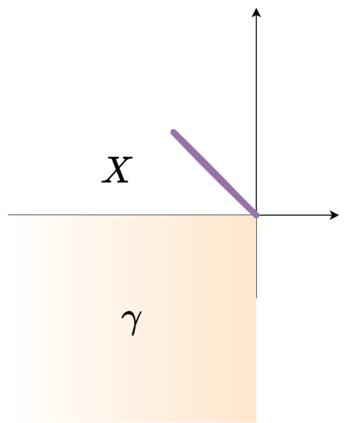
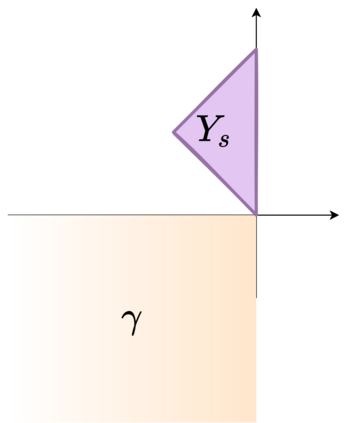
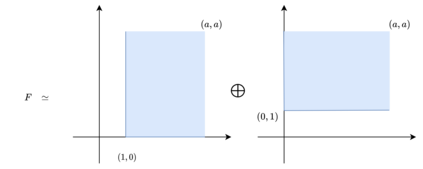
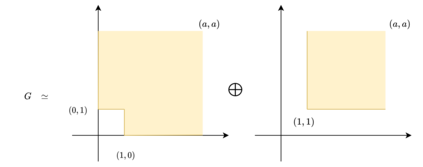
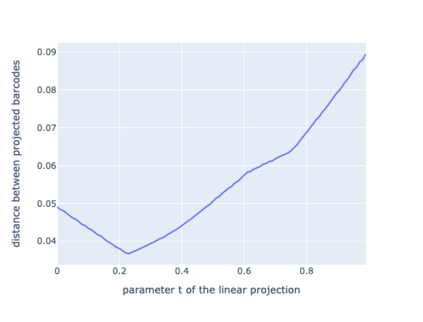
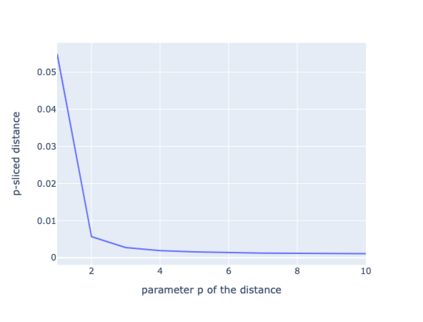
We introduce the new notions of projected distances and projected barcodes for multi-parameter persistence modules. Projected barcodes are defined as derived pushforward of persistence modules onto $\mathbb{R}$. Projected distances come in two flavors: the integral sheaf metrics (ISM) and the sliced convolution distances (SCD). We conduct a systematic study of the stability of projected barcodes and show that the fibered barcode is a particular instance of projected barcodes. We prove that the ISM and the SCD provide lower bounds for the convolution distance. Furthermore, we show that the $\gamma$-linear ISM and the $\gamma$-linear SCD which are projected distances tailored for $\gamma$-sheaves can be computed using TDA software dedicated to one-parameter persistence modules. Moreover, the time and memory complexity required to compute these two metrics are advantageous, since our approach does not require computing nor storing an entire $n$-persistence module.