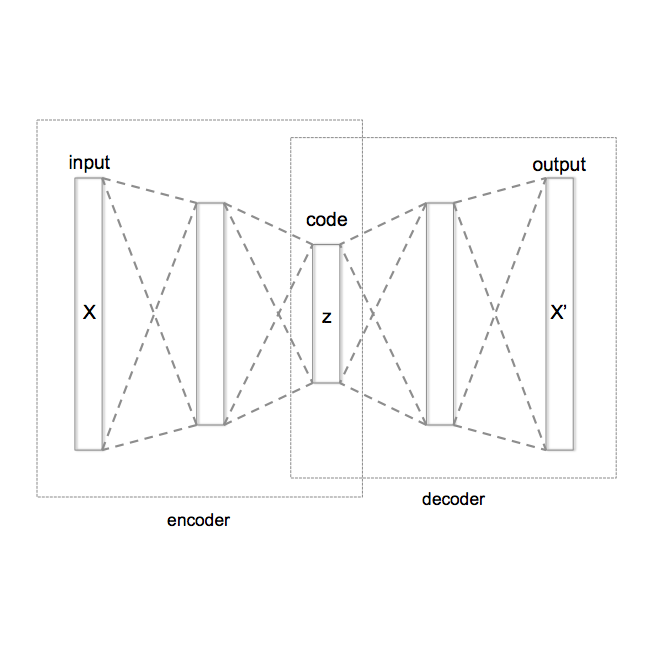
Incorporating unstructured data into physical models is a challenging problem that is emerging in data assimilation. Traditional approaches focus on well-defined observation operators whose functional forms are typically assumed to be known. This prevents these methods from achieving a consistent model-data synthesis in configurations where the mapping from data-space to model-space is unknown. To address these shortcomings, in this paper we develop a physics-informed dynamical variational autoencoder ($\Phi$-DVAE) to embed diverse data streams into time-evolving physical systems described by differential equations. Our approach combines a standard, possibly nonlinear, filter for the latent state-space model and a VAE, to assimilate the unstructured data into the latent dynamical system. Unstructured data, in our example systems, comes in the form of video data and velocity field measurements, however the methodology is suitably generic to allow for arbitrary unknown observation operators. A variational Bayesian framework is used for the joint estimation of the encoding, latent states, and unknown system parameters. To demonstrate the method, we provide case studies with the Lorenz-63 ordinary differential equation, and the advection and Korteweg-de Vries partial differential equations. Our results, with synthetic data, show that $\Phi$-DVAE provides a data efficient dynamics encoding methodology which is competitive with standard approaches. Unknown parameters are recovered with uncertainty quantification, and unseen data are accurately predicted.
翻译:暂无翻译