A Simple and Tighter Derivation of Achievability for Classical Communication over Quantum Channels

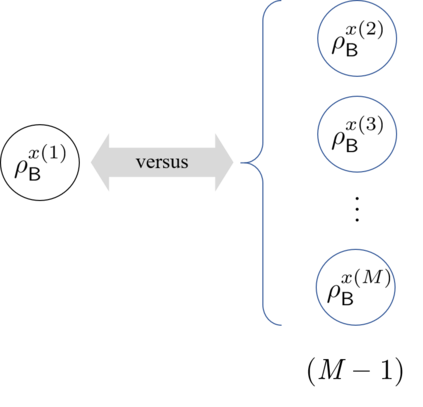
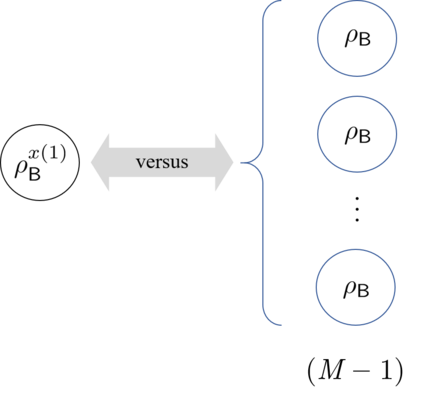
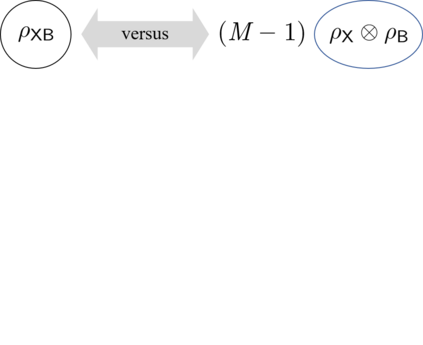
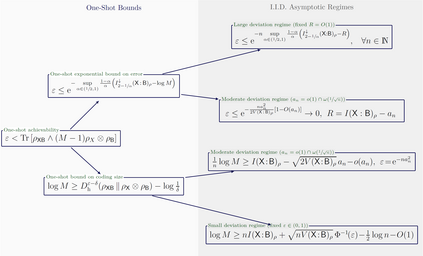
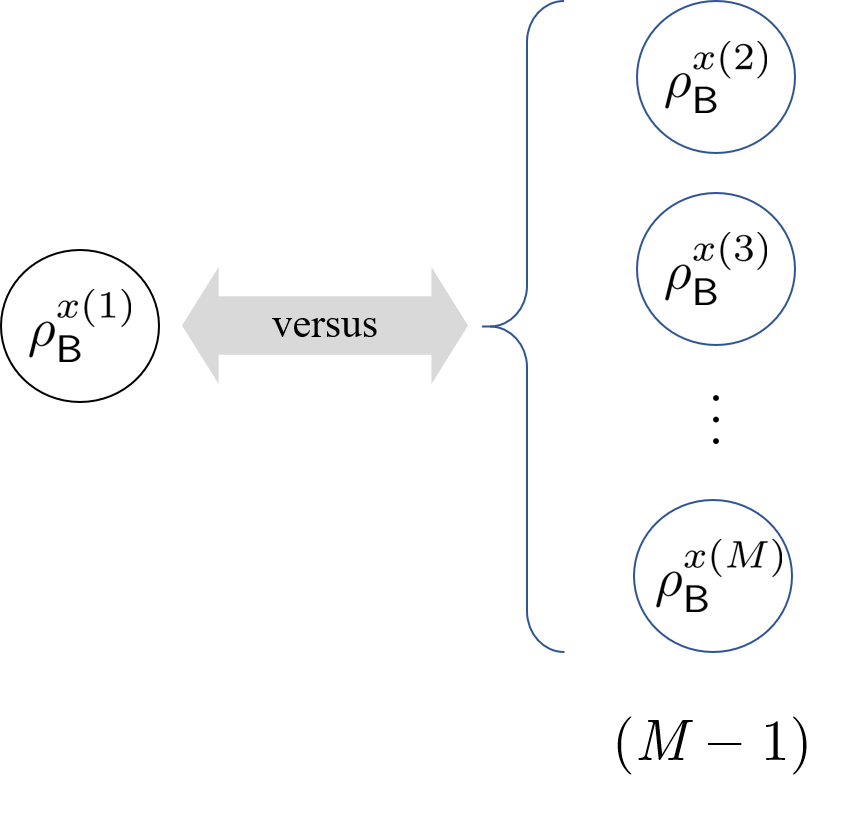
Achievability in information theory refers to demonstrating a coding strategy that accomplishes a prescribed performance benchmark for the underlying task. In quantum information theory, the crafted Hayashi-Nagaoka operator inequality is an essential technique in proving a wealth of one-shot achievability bounds since it effectively resembles a union bound in various problems. In this work, we show that the pretty-good measurement naturally plays a role as the union bound as well. A judicious application of it considerably simplifies the derivation of one-shot achievability for classical-quantum (c-q) channel coding via an elegant three-line proof. The proposed analysis enjoys the following favorable features: (i) The established one-shot bound admits a closed-form expression as in the celebrated Holevo-Helstrom Theorem. Namely, the average error probability of sending $M$ messages through a c-q channel is upper bounded by the error of distinguishing the joint state between channel input and output against $(M-1)$-many products of its marginals. (ii) Our bound directly yields asymptotic results in the large deviation, small deviation, and moderate deviation regimes in a unified manner. (iii) The coefficients incurred in applying the Hayashi-Nagaoka operator inequality are no longer needed. Hence, the derived one-shot bound sharpens existing results that rely on the Hayashi-Nagaoka operator inequality. In particular, we obtain the tightest achievable $\epsilon$-one-shot capacity for c-q channel heretofore, and it improves the third-order coding rate in the asymptotic scenario. (iv) Our result holds for infinite-dimensional Hilbert space. (v) The proposed method applies to deriving one-shot bounds for data compression with quantum side information, entanglement-assisted classical communication over quantum channels, and various quantum network information-processing protocols.