Artificial Neural Network evaluation of Poincaré constant for Voronoi polygons

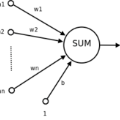
We propose a method, based on Artificial Neural Networks, that learns the dependence of the constant in the Poincar\'e inequality on polygonal elements of Voronoi meshes, on some geometrical metrics of the element. The cost of this kind of algorithms mainly resides in the data preprocessing and learning phases, that can be performed offline once and for all, constructing an efficient method for computing the constant, which is needed in the design of a posteriori error estimates in numerical mesh-based schemes for the solution of Partial Differential Equations.