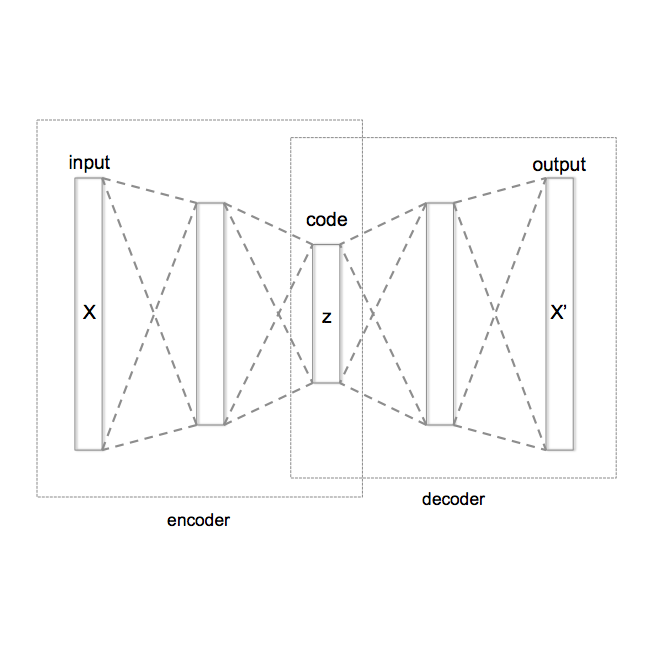
Variational autoencoder (VAE) estimates the posterior parameters (mean and variance) of latent variables corresponding to each input data. While it is used for many tasks, the transparency of the model is still an underlying issue. This paper provides a quantitative understanding of VAE property through the differential geometric and information-theoretic interpretations of VAE. According to the Rate-distortion theory, the optimal transform coding is achieved by using an orthonormal transform with PCA basis where the transform space is isometric to the input. Considering the analogy of transform coding to VAE, we clarify theoretically and experimentally that VAE can be mapped to an implicit isometric embedding with a scale factor derived from the posterior parameter. As a result, we can estimate the data probabilities in the input space from the prior, loss metrics, and corresponding posterior parameters, and further, the quantitative importance of each latent variable can be evaluated like the eigenvalue of PCA.
翻译:变量自动编码器(VAE)估算了与每项输入数据相对应的潜在变量的后方参数(平均值和差异),虽然该模型用于许多任务,但其透明度仍然是一个基本问题。本文件通过对 VAE 的不同几何和信息理论解释,从数量上理解VAE属性。根据率扭曲理论,最佳变异编码是通过使用一种正态变换法实现的,这种变异法以CPA为基础,使变异空间与输入相等。考虑到将编码转换为VAE的类比,我们从理论上和实验上澄清,VAE可被映为隐含的等离子嵌嵌嵌嵌,而该隐含因子参数而得出一个比例系数。因此,我们可以估计从先前的、损失指标和相应的后方参数中输入空间中的数据概率,此外,每个潜在变量的量化重要性可以像五氯苯甲醚的乙基因值一样加以评估。