Training Recurrent Neural Networks by Sequential Least Squares and the Alternating Direction Method of Multipliers

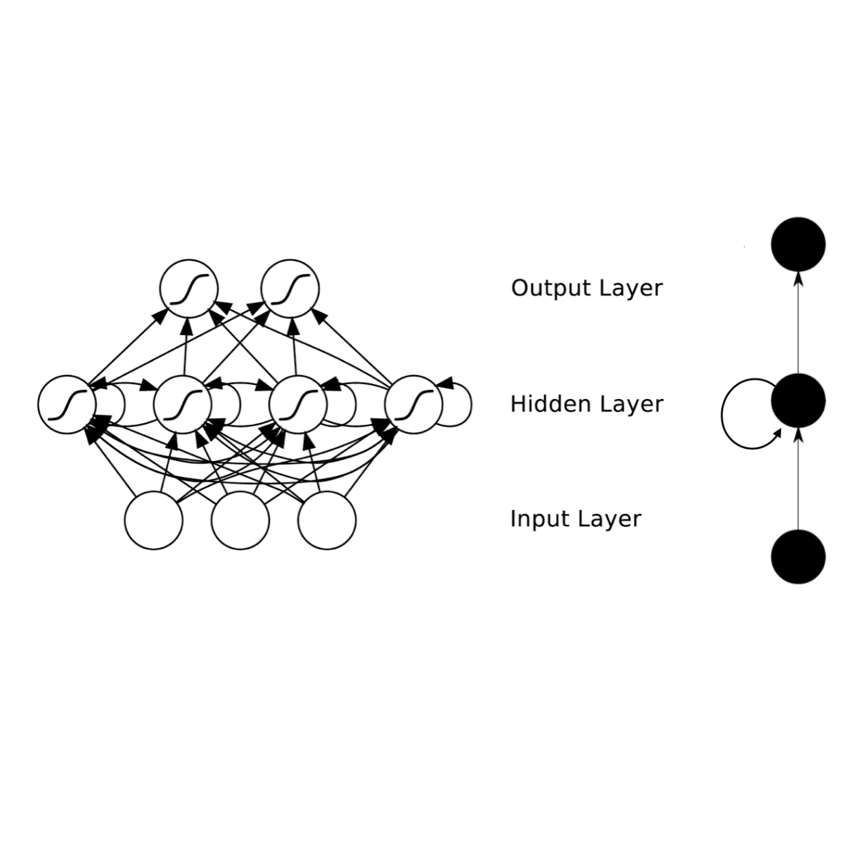
This paper proposes a novel algorithm for training recurrent neural network models of nonlinear dynamical systems from an input/output training dataset. Arbitrary convex and twice-differentiable loss functions and regularization terms are handled by sequential least squares and either a line-search (LS) or a trust-region method \`a la Levenberg-Marquardt (LM) for ensuring convergence. In addition, to handle non-smooth regularization terms such as $\ell_1$, $\ell_0$, and group-Lasso regularizers, as well as to impose possibly non-convex constraints such as integer and mixed-integer constraints, we combine sequential least squares with the alternating direction method of multipliers (ADMM). We call the resulting algorithm NAILS (nonconvex ADMM iterations and least squares) in the case line search (LS) is used, or NAILM if a trust-region method (LM) is employed instead. The training method, which is also applicable to feedforward neural networks as a special case, is tested in two nonlinear system identification problems.