The Impacts of Dimensionality, Diffusion, and Directedness on Intrinsic Cross-Model Simulation in Tile-Based Self-Assembly

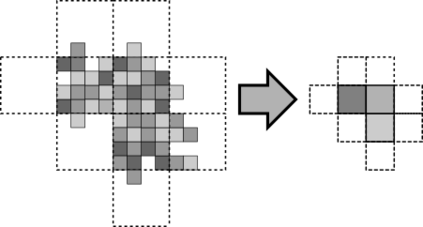
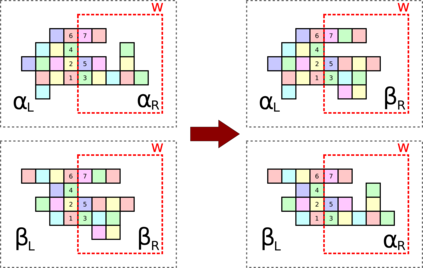
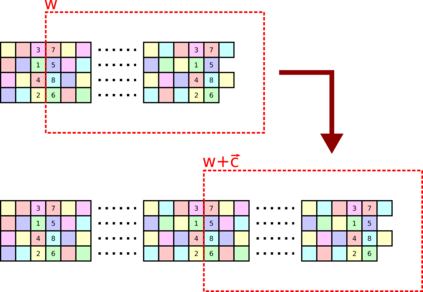
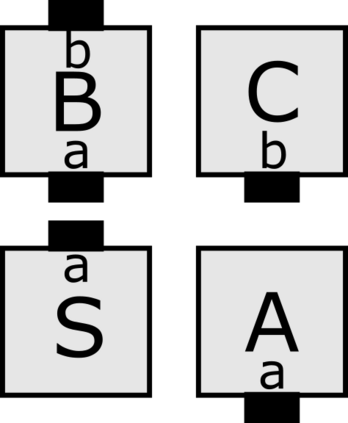
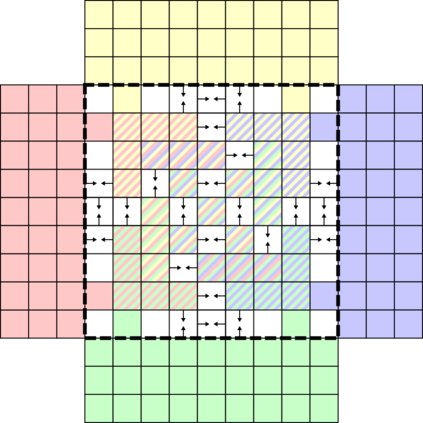
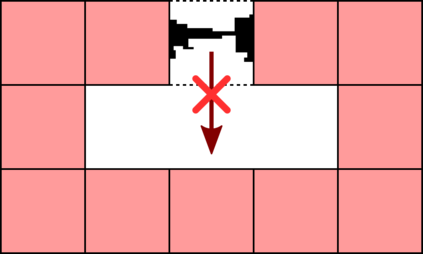
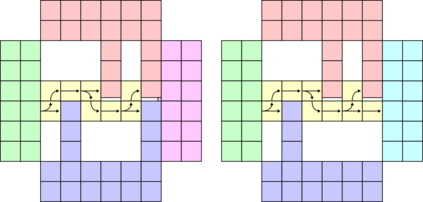
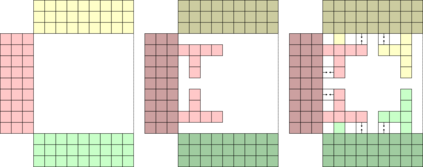
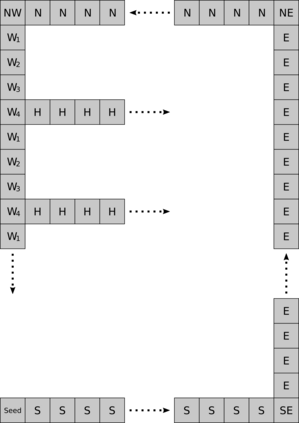
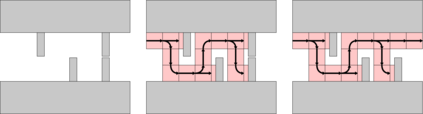
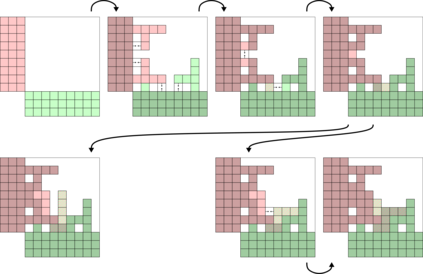
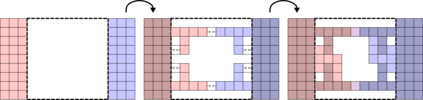
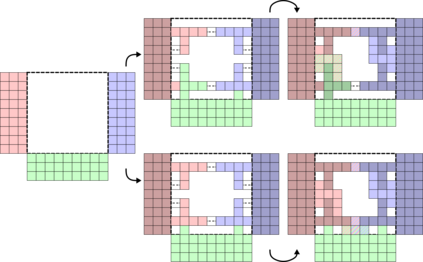
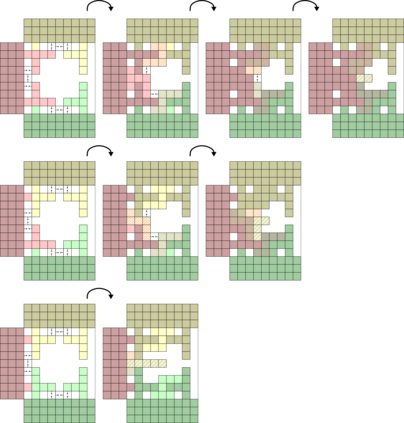
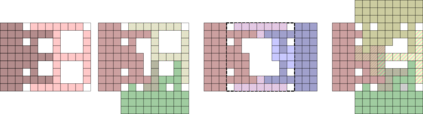
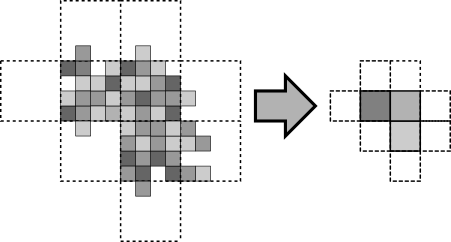
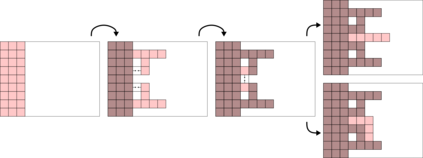Algorithmic self-assembly occurs when disorganized components autonomously combine to form structures and, by their design and the dynamics of the system, are forced to follow the execution of algorithms. Motivated by applications in DNA-nanotechnology, investigations in algorithmic tile-based self-assembly have blossomed into a mature theory with research leveraging tools from computability theory, complexity theory, information theory, and graph theory to develop a wide range of models and show that many are computationally universal, while also exposing powers and limitations of each. Beyond computational universality, the abstract Tile Assembly Model (aTAM) was shown to be intrinsically universal (IU), a strong notion of completeness where a single tile set is capable of simulating all systems within the model; however, this result required non-deterministic tile attachments. This was later confirmed necessary when it was shown that the class of directed aTAM systems is not IU. Building on these results to further investigate the impacts of other dynamics, Hader et al. examined several tile-assembly models which varied across (1) the numbers of dimensions used, (2) restrictions based on diffusion of tiles through space, and (3) whether each system is directed, and showed which models are IU. Such results have shed much light on the roles of various aspects of the dynamics of tile-assembly and their effects on the intrinsic universality of each model. Here we provide direct comparisons of the various models by considering intrinsic simulations between models. We show that in some cases one model is more powerful than another, and in others, pairs of models have mutually exclusive capabilities. This comparison helps to expose the impacts of these three important aspects and further helps define a hierarchy of tile-assembly models.