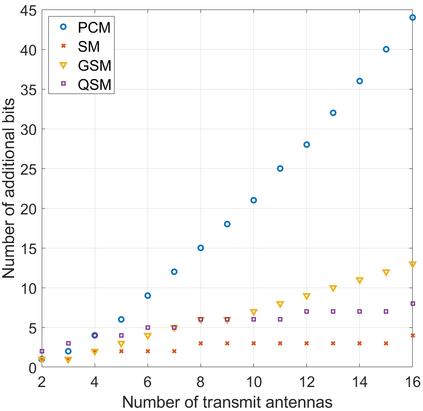
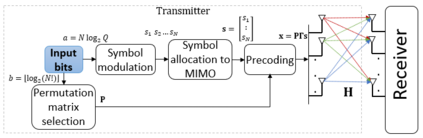
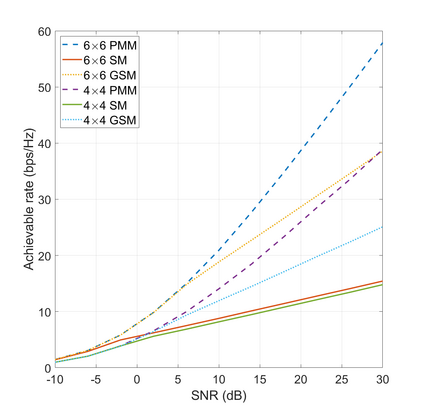
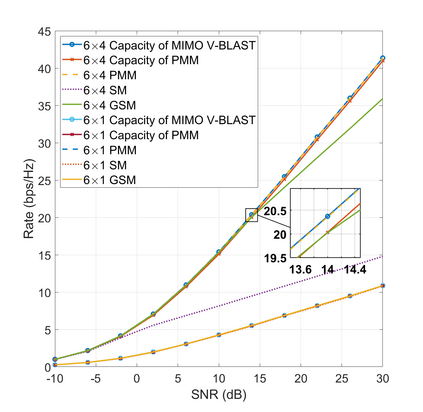
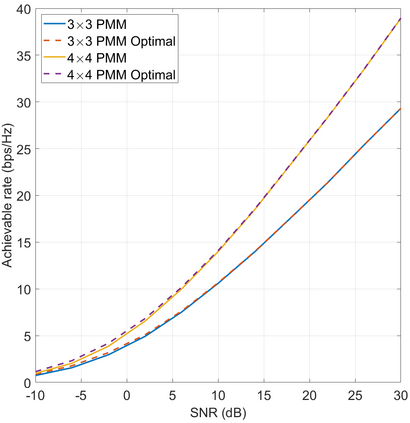
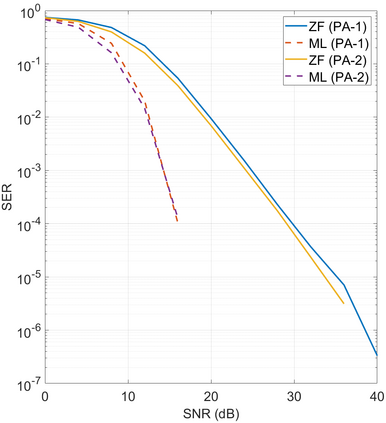
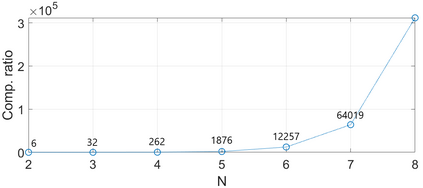
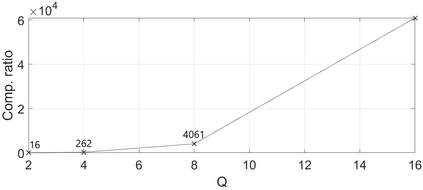
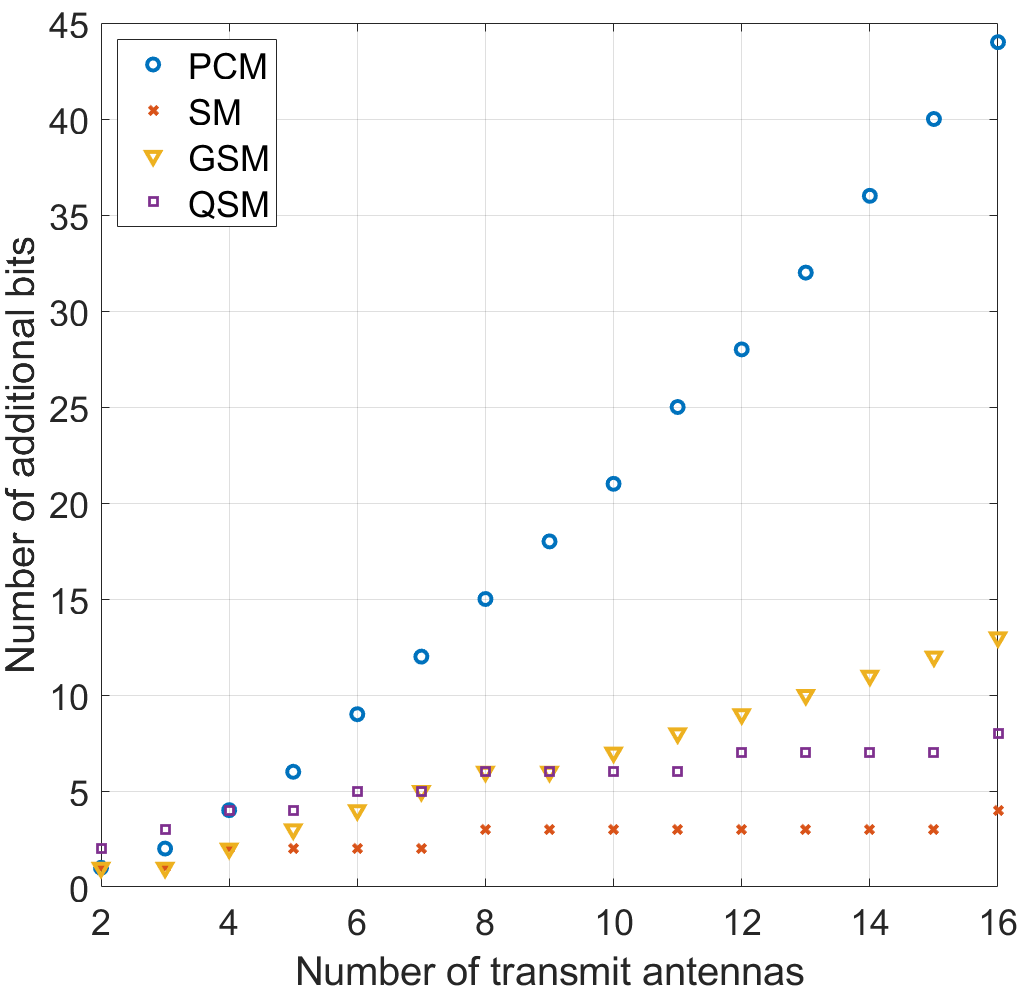
We propose a novel scheme that allows MIMO system to modulate a set of permutation matrices to send more information bits, extending our initial work on the topic. This system is called Permutation Matrix Modulation (PMM). The basic idea is to employ a permutation matrix as a precoder and treat it as a modulated symbol. We continue the evolution of index modulation in MIMO by adopting all-antenna activation and obtaining a set of unique symbols from altering the positions of the antenna transmit power. We provide the analysis of the achievable rate of PMM under Gaussian Mixture Model (GMM) distribution and evaluate the numerical results by comparing it with the other existing systems. The result shows that PMM outperforms the existing systems under a fair parameter setting. We also present a way to attain the optimal achievable rate of PMM by solving a maximization problem via interior-point method. A low complexity detection scheme based on zero-forcing (ZF) is proposed, and maximum likelihood (ML) detection is discussed. We demonstrate the trade-off between simulation of the symbol error rate (SER) and the computational complexity where ZF performs worse in the SER simulation but requires much less computational complexity than ML.
翻译:我们提出一个新方案,让IMIMO系统能够调整一套调整矩阵,以发送更多的信息比特,扩大我们关于这个专题的初步工作。这个系统称为“变异矩阵模型模型(PMM) ” 。其基本想法是使用一个变异矩阵矩阵作为预解解器,并将其作为一个调制符号。我们继续通过采用全ANETnn纳的激活和从改变天天天传输功传输力位置改变天天线传输力位置获得一套独特的符号,在MOIM上演一套新方案,使MIM系统能够调制成一套新的矩阵,以发送更多的信息比特,扩展我们的初始工作。这个系统称为“变异矩阵矩阵模型”的最初工作。这个系统称为“变异矩阵模型”的最初工作。这个系统的基本想法是使用一个变异变矩阵作为预解器,将它作为调制符号错误率的模型,并把它当作一个调制符号传输信号传输传输能力最优化的标志调控点问题。我们提议了一个基于零叉(ZF)的低复杂探测机制,并讨论最大可能性(ML)探测。我们演示了在模拟符号错误率(SER)的模拟中,但更差的模拟需要更低的MZMZ进行比MZ的模拟,但更低的模拟需要,进行更低的MZ(MZ)和计算,进行更更更低的MZL)的比的模拟,进行。