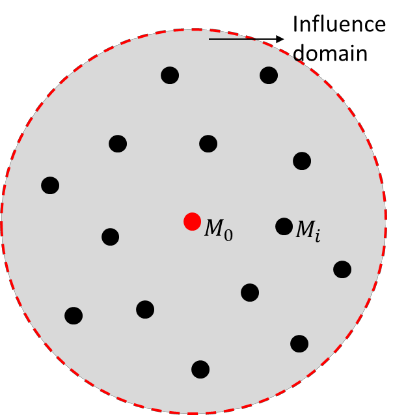
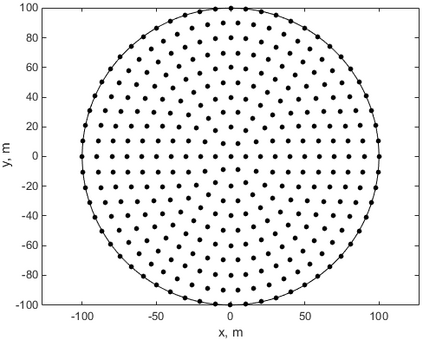
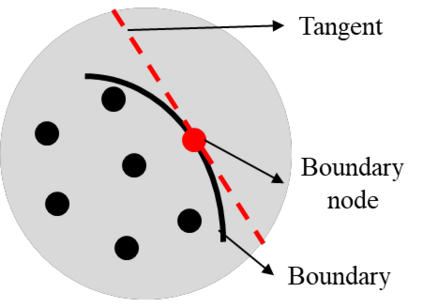
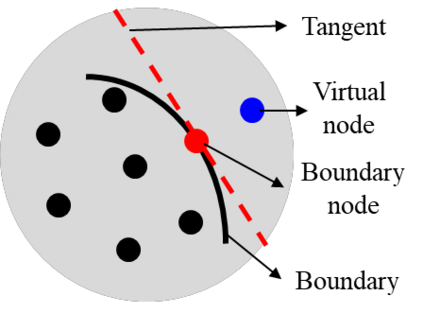
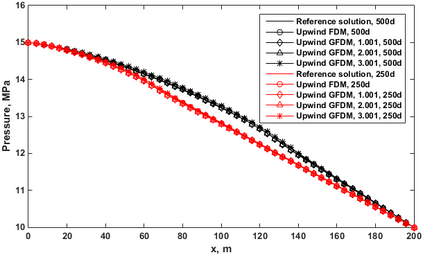
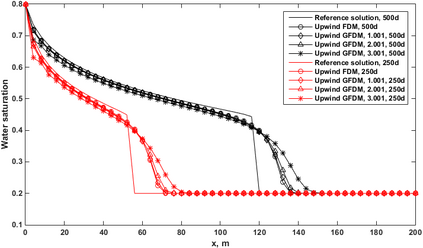
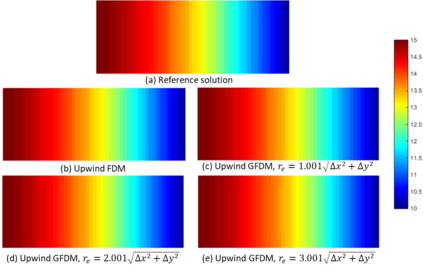
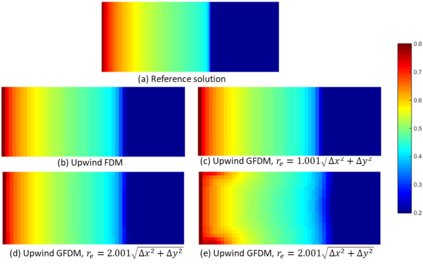
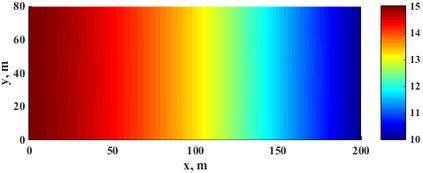
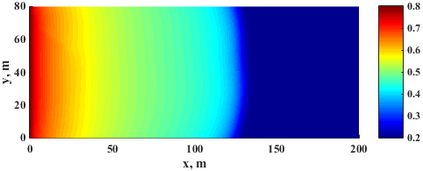
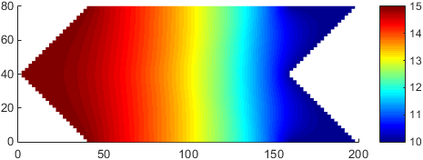
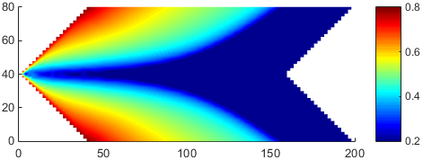
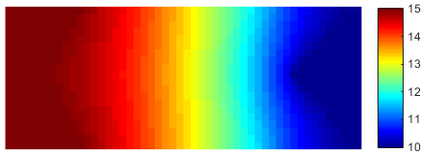
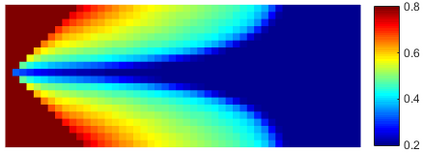
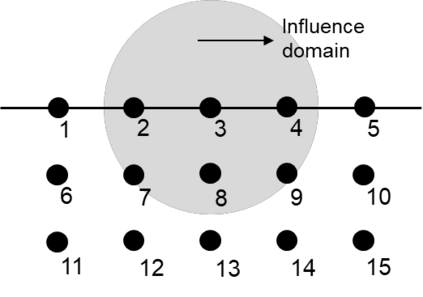
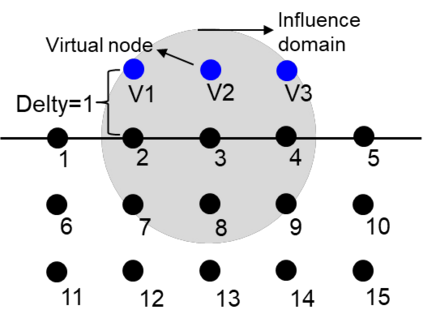
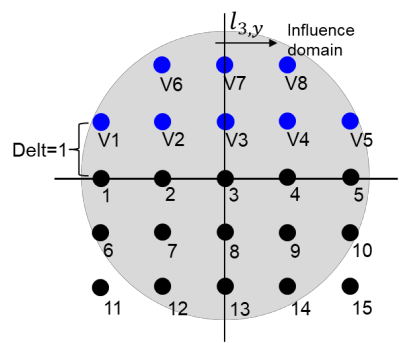
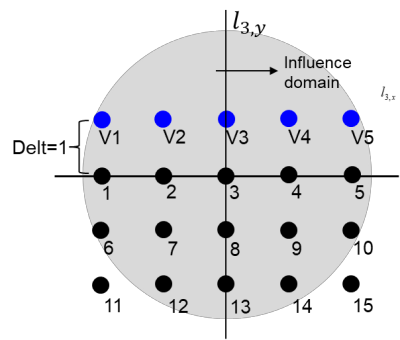
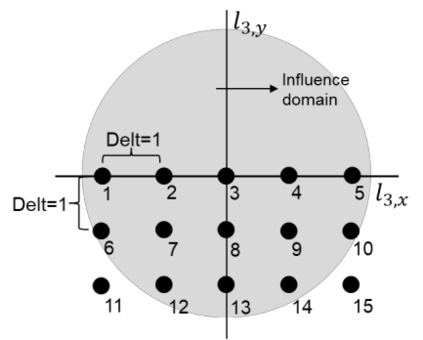
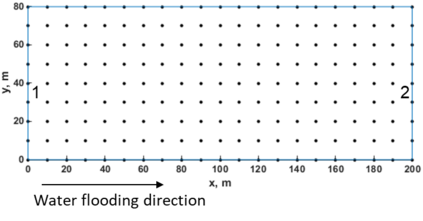
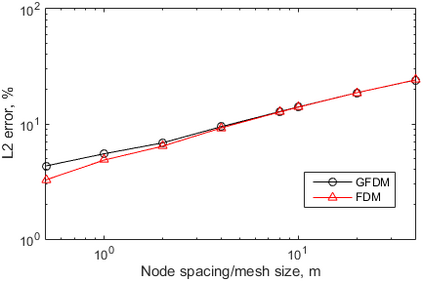
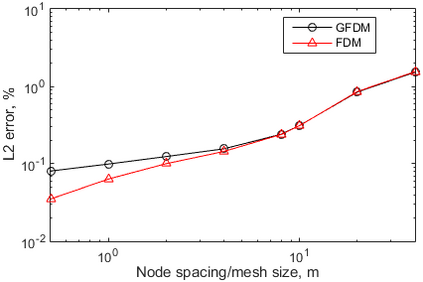
This paper makes the first attempt to apply newly developed upwind GFDM for the meshless solution of two-phase porous flow equations. In the presented method, node cloud is used to flexibly discretize the computational domain, instead of complicated mesh generation. Combining with moving least square approximation and local Taylor expansion, spatial derivatives of oil-phase pressure at a node are approximated by generalized difference operators in the local influence domain of the node. By introducing the first-order upwind scheme of phase relative permeability, and combining the discrete boundary conditions, fully-implicit GFDM-based nonlinear discrete equations of the immiscible two-phase porous flow are obtained and solved by the nonlinear solver based on the Newton iteration method with the automatic differentiation, to avoid the additional computational cost and possible computational instability caused by sequentially coupled scheme. Two numerical examples are implemented to test the computational performances of the presented method. Detailed error analysis finds the two sources of the calculation error, roughly studies the convergence order thus find that the low-order error of GFDM makes the convergence order of GFDM lower than that of FDM when node spacing is small, and points out the significant effect of the symmetry or uniformity of the node collocation in the node influence domain on the accuracy of generalized difference operators, and the radius of the node influence domain should be small to achieve high calculation accuracy, which is a significant difference between the studied hyperbolic two-phase porous flow problem and the elliptic problems when GFDM is applied.
翻译:本文首次尝试将新开发的上风GFDM应用于两阶段多孔流量方程式的无孔不入的解决方案。 在所采用的方法中,节点云用于使计算域灵活分散,而不是复杂的网状生成。结合移动最小平方近似值和局部泰勒扩展,节点的石油阶段压力的空间衍生物被节点当地影响域的普遍差异操作者所近似。通过引入第一级低端流方程式相对渗透性,并结合离散边界条件,完全模糊的基于GFDMDM的双阶段多孔流动非线性离散方程式,由基于牛顿重复法的非线性求解器获取和解决,以避免额外的计算成本和可能的计算不稳定性。采用两个数字示例来测试所介绍的方法的计算性性能。 详细错误分析发现计算误差的两个来源, 仔细研究后发现,GFDDDM的低端非线性非线性非线性等方方方方方方方方方方程式的精确性方程式,使GFDDM的轨道的平稳流流流率高于FDM的两点,因此, 的平流流流流流流流流流流流流流流流流流流流流流的精度不能的精度的精度的精度的精度不能的精度不能达的精度不能达到高。