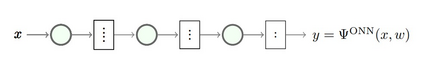
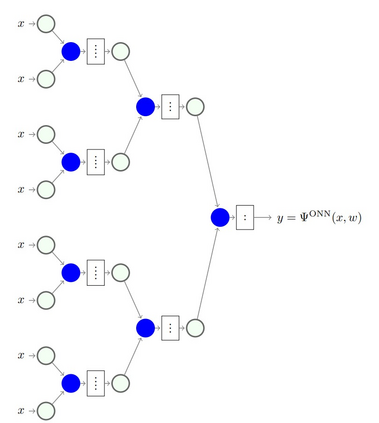
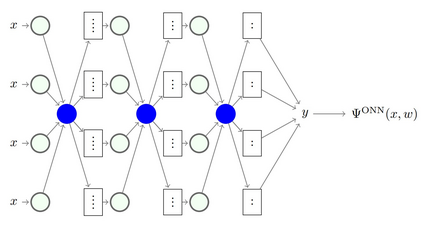
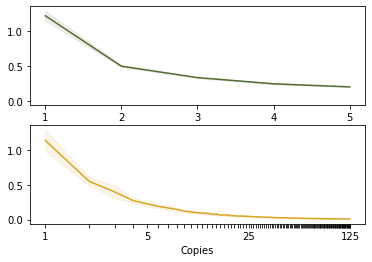
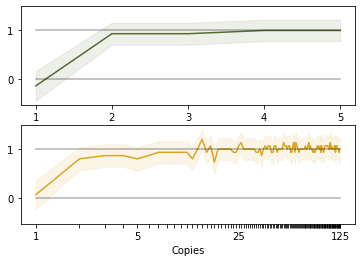
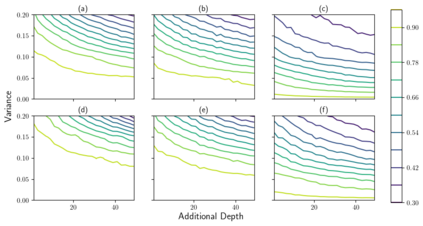
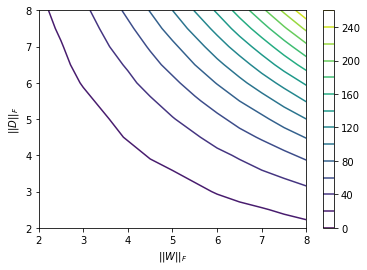
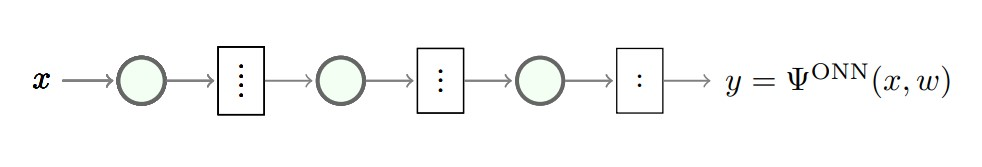
All analog signal processing is fundamentally subject to noise, and this is also the case in modern implementations of Optical Neural Networks (ONNs). Therefore, to mitigate noise in ONNs, we propose two designs that are constructed from a given, possibly trained, Neural Network (NN) that one wishes to implement. Both designs have the capability that the resulting ONNs gives outputs close to the desired NN. To establish the latter, we analyze the designs mathematically. Specifically, we investigate a probabilistic framework for the first design that establishes that the design is correct, i.e., for any feed-forward NN with Lipschitz continuous activation functions, an ONN can be constructed that produces output arbitrarily close to the original. ONNs constructed with the first design thus also inherit the universal approximation property of NNs. For the second design, we restrict the analysis to NNs with linear activation functions and characterize the ONNs' output distribution using exact formulas. Finally, we report on numerical experiments with LeNet ONNs that give insight into the number of components required in these designs for certain accuracy gains. We specifically study the effect of noise as a function of the depth of an ONN. The results indicate that in practice, adding just a few components in the manner of the first or the second design can already be expected to increase the accuracy of ONNs considerably.
翻译:暂无翻译