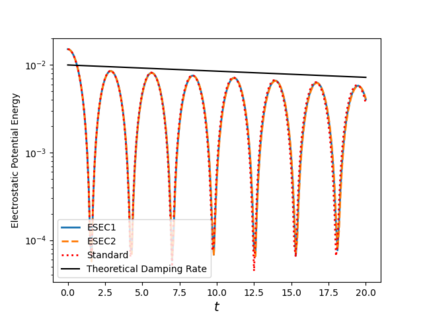
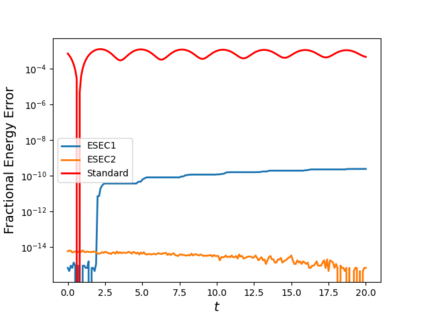
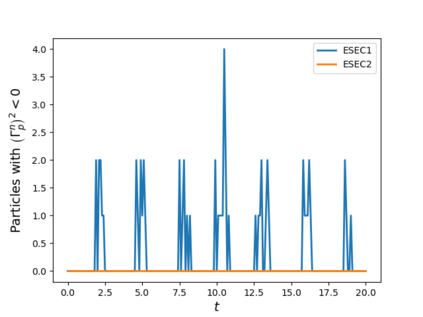
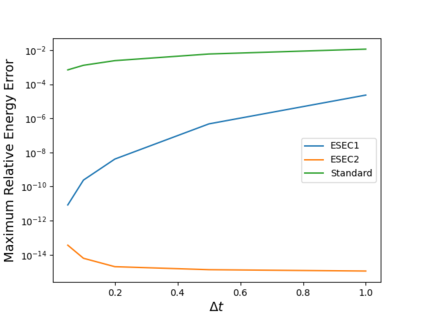
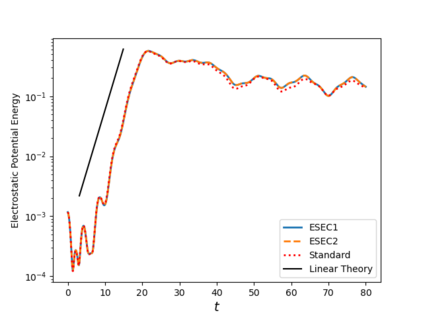
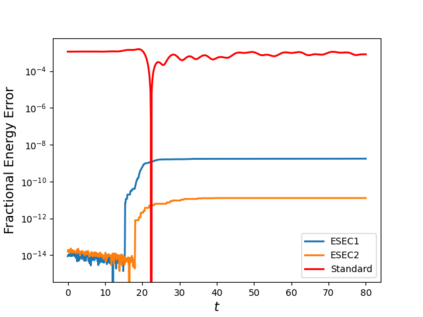
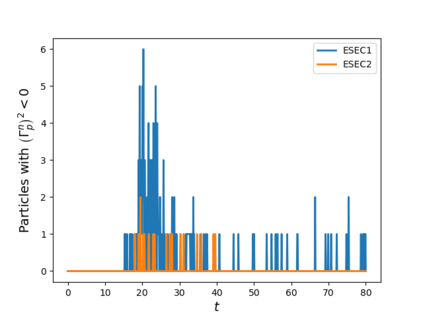
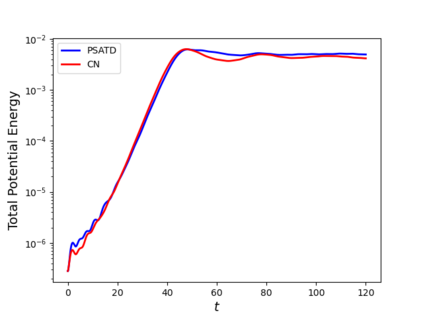
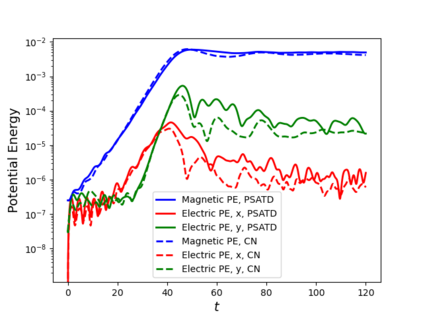
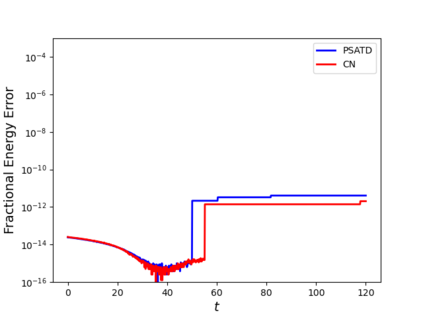
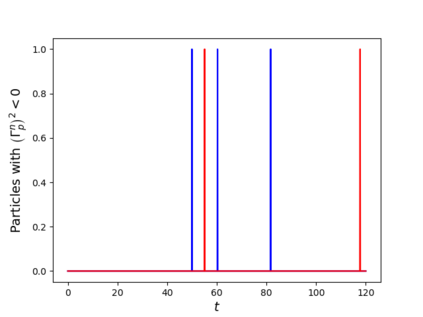
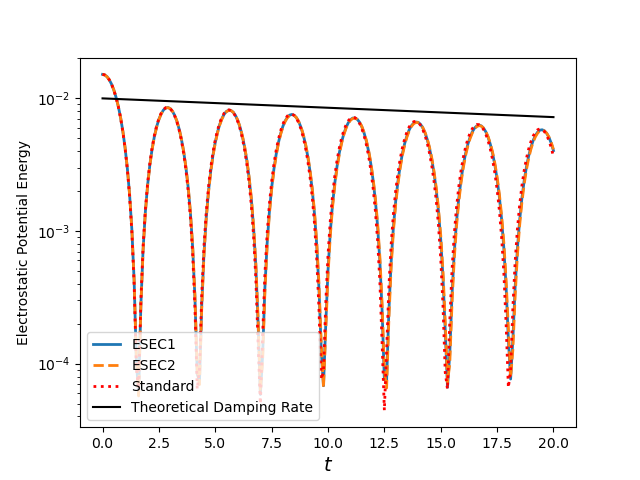
We present an explicit temporal discretization of particle-in-cell schemes for the Vlasov equation that results in exact energy conservation when combined with an appropriate spatial discretization. The scheme is inspired by a simple, second-order explicit scheme that conserves energy exactly in the Eulerian context. We show that direct translation to particle-in-cell does not result in strict conservation, but derive a simple correction based on an analytically solvable optimization problem that recovers conservation. While this optimization problem is not guaranteed to have a real solution for every particle, we provide a correction that makes imaginary values extremely rare and still admits $\mathcal{O}(10^{-12})$ fractional errors in energy for practical simulation parameters. We present the scheme in both electrostatic -- where we use the Amp\`{e}re formulation -- and electromagnetic contexts. With an electromagnetic field solve, the field update is most naturally linearly implicit, but the more computationally intensive particle update remains fully explicit. We also show how the scheme can be extended to use the fully explicit leapfrog and pseudospectral analytic time-domain (PSATD) field solvers. The scheme is tested on standard kinetic plasma problems, confirming its conservation properties.
翻译:暂无翻译