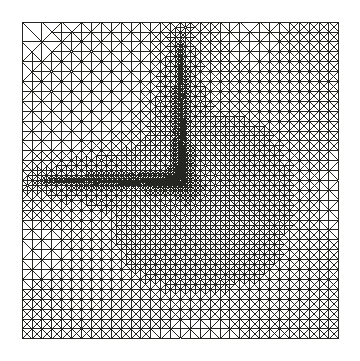
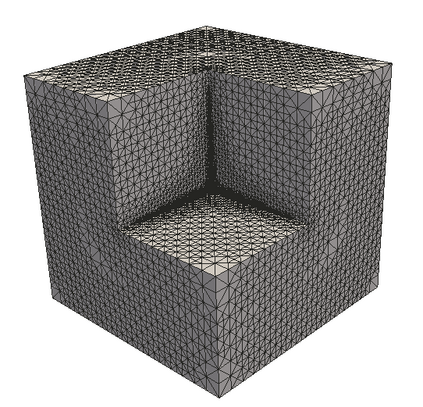
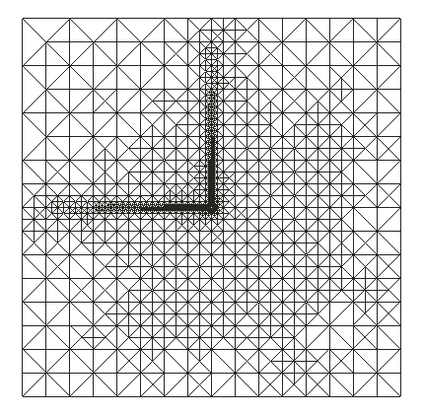
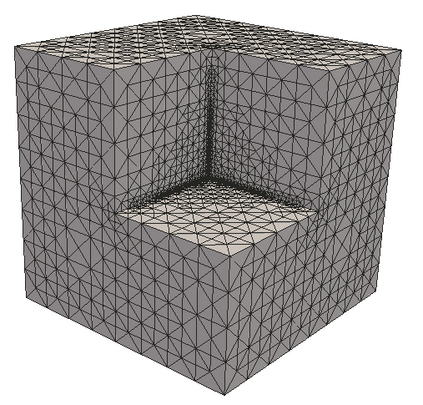
The aim of this paper is to propose an efficient adaptive finite element method for eigenvalue problems based on the multilevel correction scheme and inverse power method. This method involves solving associated boundary value problems on each adaptive partitions and very low dimensional eigenvalue problems on some special meshes which are controlled by the proposed algorithm. Since we Hence the efficiency of solving eigenvalue problems can be improved to be similar to the adaptive finite element method for the associated boundary value problems. The convergence and optimal complexity is theoretically verified and numerically demonstrated.
翻译：本文件的目的是根据多级校正办法和反向功率方法,提出一种高效的适应性定值方法,用于解决乙基值问题,这种方法涉及解决每个适应性分区的相关边界值问题,以及由拟议算法控制的某些特殊介质的非常低的维度乙基值问题。由于我们因此可以提高解决乙基值问题的效率,使之与相关边界值问题的适应性定值方法相类似。趋同性和最佳复杂性在理论上得到核实,并在数字上得到证明。
相关内容
微信扫码咨询专知VIP会员