Approximation of solutions of DDEs under nonstandard assumptions via Euler scheme

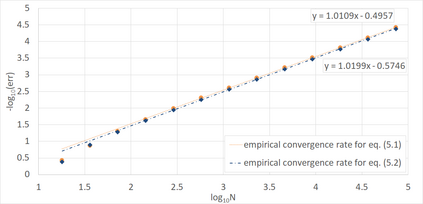
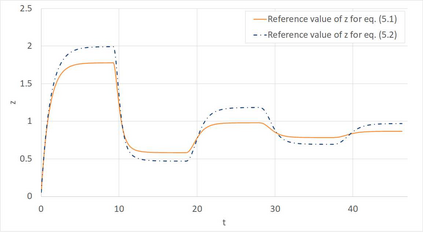
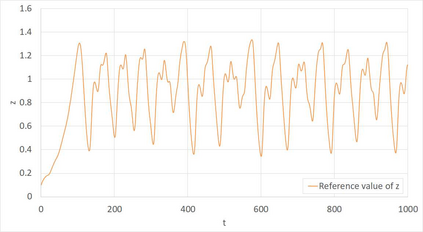
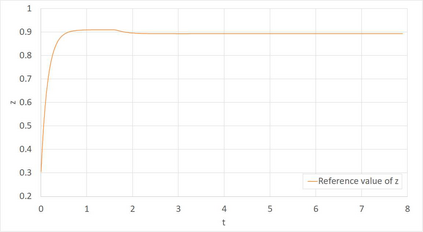
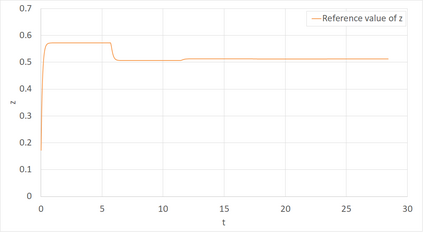
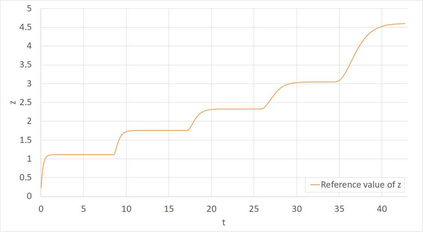
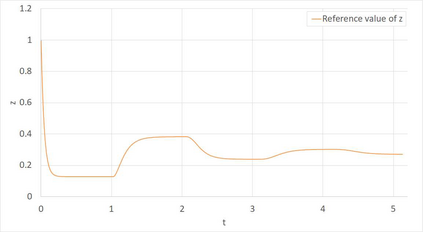
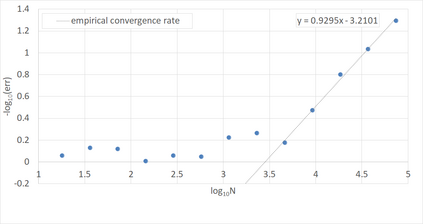
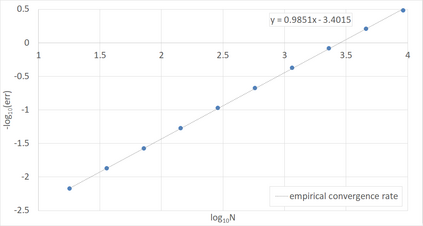
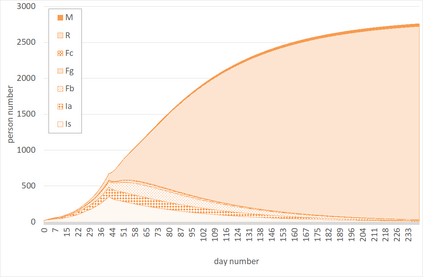
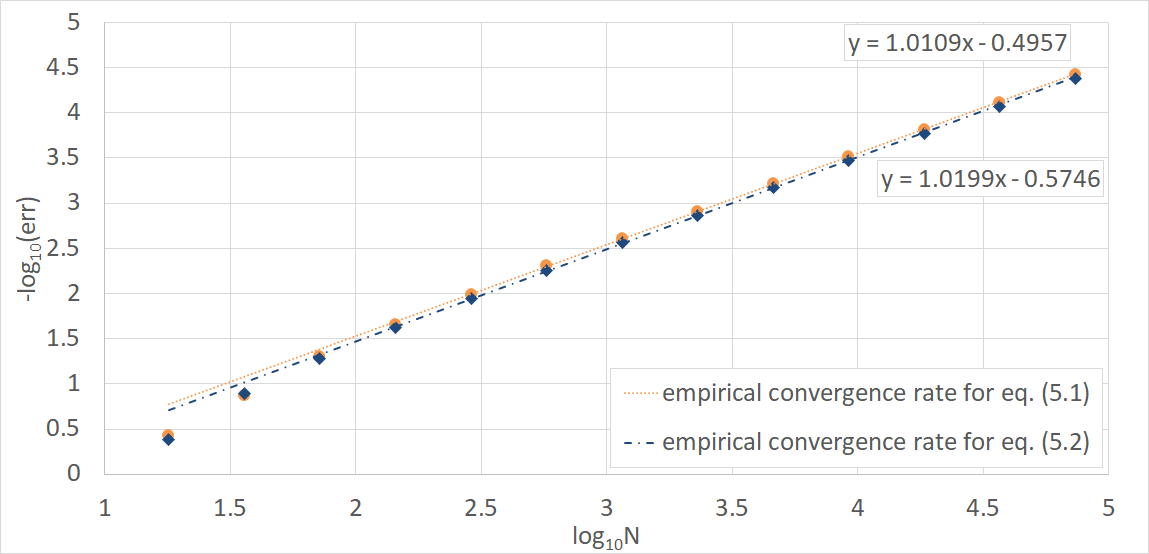
We deal with approximation of solutions of delay differential equations (DDEs) via the classical Euler algorithm. We investigate the pointwise error of the Euler scheme under nonstandard assumptions imposed on the right-hand side function $f$. Namely, we assume that $f$ is globally of at most linear growth, satisfies globally one-side Lipschitz condition but it is only locally H\"older continuous. We provide a detailed error analysis of the Euler algorithm under such nonstandard regularity conditions. Moreover, we report results of numerical experiments.