Global Convergence Rate Analysis of Nonsmooth Nonconvex-Nonconcave Minimax Optimization

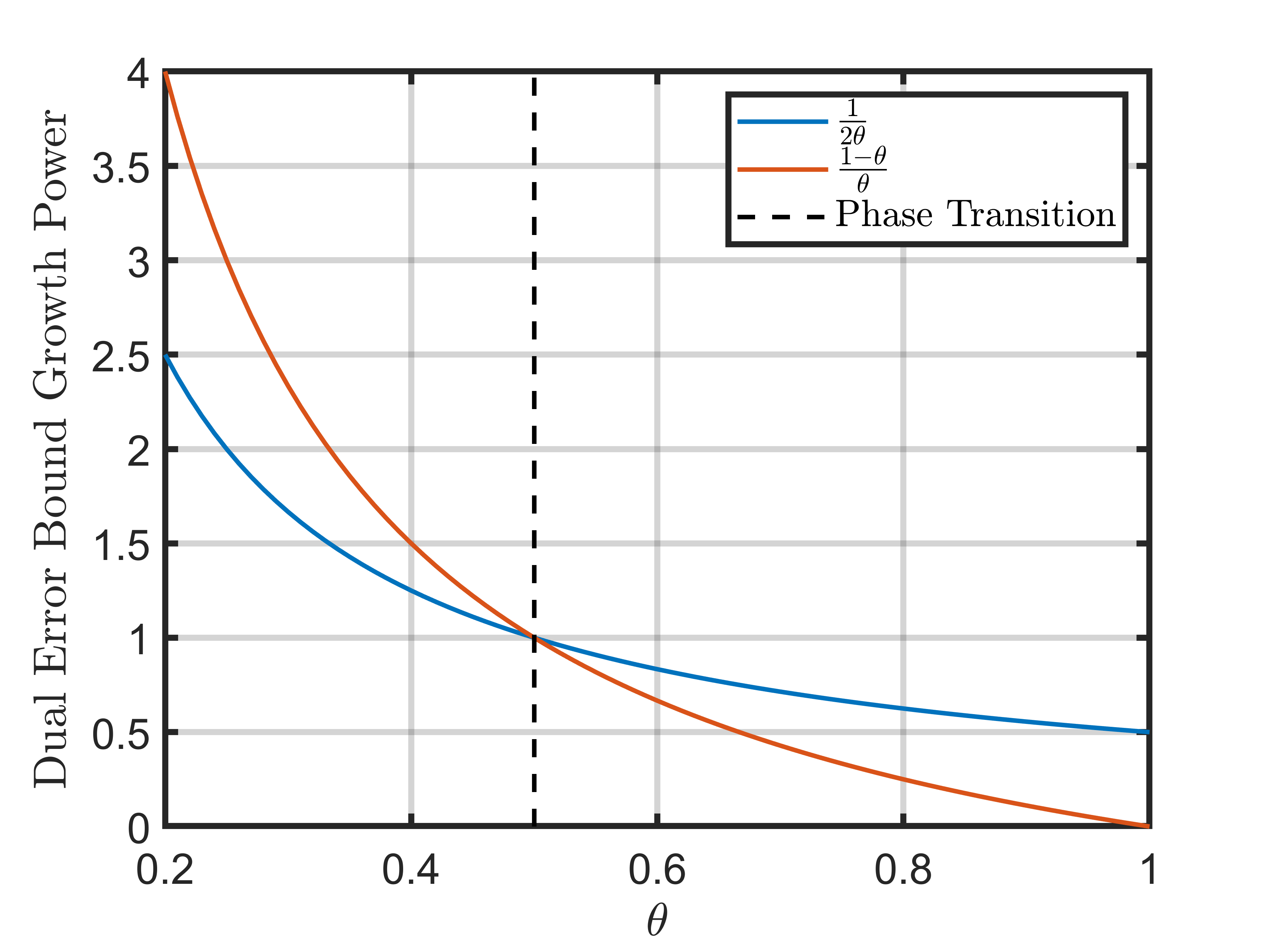
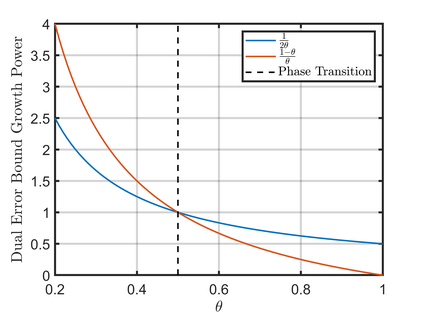
Nonconvex-nonconcave minimax optimization has gained widespread interest over the last decade. However, most existing work focuses on variants of gradient descent-ascent (GDA) algorithms, which are only applicable in smooth nonconvex-concave settings. To address this limitation, we propose a novel algorithm named smoothed proximal linear descent-ascent (smoothed PLDA), which can effectively handle a broad range of structured nonsmooth nonconvex-nonconcave minimax problems. Specifically, we consider the setting where the primal function has a nonsmooth composite structure and the dual function possesses the Kurdyka-\L{}ojasiewicz (K\L{}) property with exponent $\theta \in [0,1)$. We introduce a novel convergence analysis framework for smoothed PLDA, the key components of which are our newly developed nonsmooth primal error bound and dual error bound properties. Using this framework, we show that smoothed PLDA can find both $\epsilon$-game-stationary points and $\epsilon$-optimization-stationary points of the problems of interest in $\mathcal{O}(\epsilon^{-2\max\{2\theta,1\}})$ iterations. Furthermore, when $\theta \in [0,1/2]$, smoothed PLDA achieves the optimal iteration complexity of $\mathcal{O}(\epsilon^{-2})$. To further demonstrate the effectiveness and wide applicability of our analysis framework, we show that certain max-structure problem possesses the K\L{} property with exponent $\theta=0$ under mild assumptions. As a by-product, we establish algorithm-independent quantitative relationships among various stationarity concepts, which may be of independent interest.