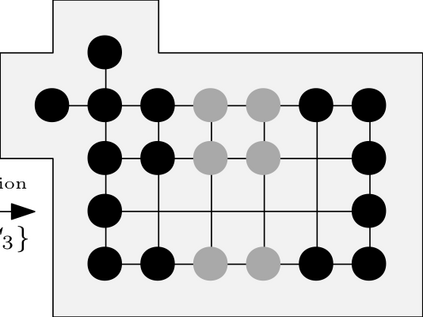
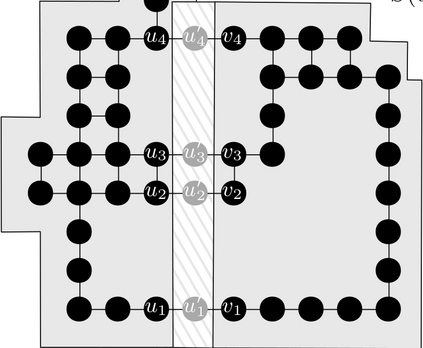
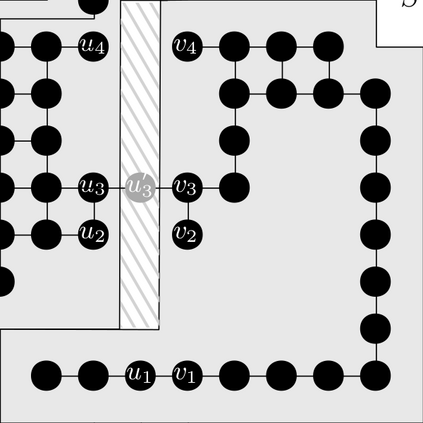
In this work, we investigate novel algorithmic growth processes. In particular, we propose three growth operations, full doubling, RC doubling and doubling, and explore the algorithmic and structural properties of their resulting processes under a geometric setting. In terms of modeling, our system runs on a 2-dimensional grid and operates in discrete time-steps. The process begins with an initial shape $S_I=S_0$ and, in every time-step $t \geq 1$, by applying (in parallel) one or more growth operations of a specific type to the current shape-instance $S_{t-1}$, generates the next instance $S_t$, always satisfying $|S_t| > |S_{t-1}|$. Our goal is to characterize the classes of shapes that can be constructed in $O(\log n)$ or polylog $n$ time-steps and determine whether a final shape $S_F$ can be constructed from an initial shape $S_I$ using a finite sequence of growth operations of a given type, called a constructor of $S_F$. For full doubling, in which, in every time-step, every node generates a new node in a given direction, we completely characterize the structure of the class of shapes that can be constructed from a given initial shape. For RC doubling, in which complete columns or rows double, our main contribution is a linear-time centralized algorithm that for any pair of shapes $S_I$, $S_F$ decides if $S_F$ can be constructed from $S_I$ and, if the answer is yes, returns an $O(\log n)$-time-step constructor of $S_F$ from $S_I$. For the most general doubling operation, where up to individual nodes can double, we show that some shapes cannot be constructed in sub-linear time-steps and give two universal constructors of any $S_F$ from a singleton $S_I$, which are efficient (i.e., up to polylogarithmic time-steps) for large classes of shapes. Both constructors can be computed by polynomial-time centralized algorithms for any shape $S_F$.
翻译:在这项工作中, 我们调查新的算法增长过程。 特别是, 我们提议三种增长操作, 完全翻番, RC翻番和翻番, 并在几何设置下探索其结果过程的算法和结构属性。 在建模方面, 我们的系统运行在二维的网格上, 运行在离散的时间步骤中。 这个过程以初始形状$S_ I=S_ 0美元开始, 在每次时间步骤 $t\ geq 1美元中, 通过( 平行) 将一种特定类型的增长操作应用到当前形状$S+1美元, 生成下一个系统$_ t$, 总是满足 $_S_ t _ > _ S\ t\\\\\\ t\\\\\\\\\\\\\\\\\\\ 美元。 我们的系统形状的形状可以定性为 $美元或 美元 美元 。 双倍的形状是美元 。 以任何S_ 美元 美元 开始的直线操作, 任何特定的成长序列, $_ $_ sal_ fremodeal_ a modeal_ modeal_ modeal_ modeal_ modeal_ modeal_ mas a.