Fully Modified Functional Principal Component Analysis for Cointegrated Functional Time Series

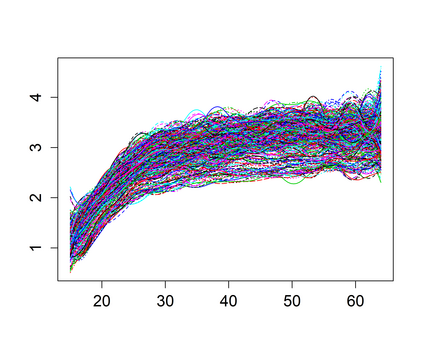
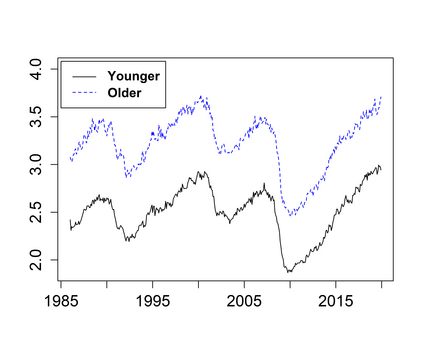
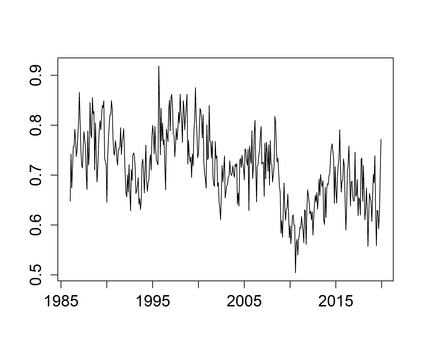
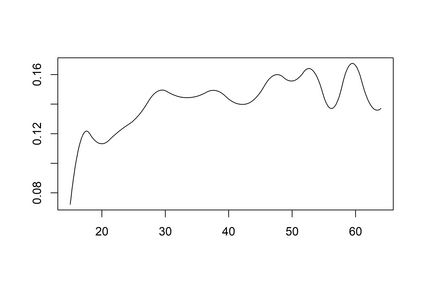
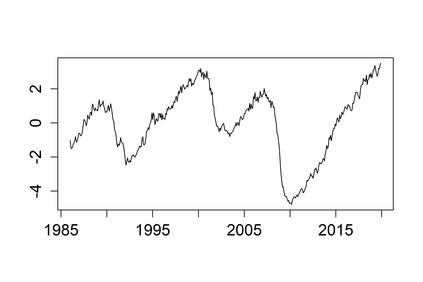
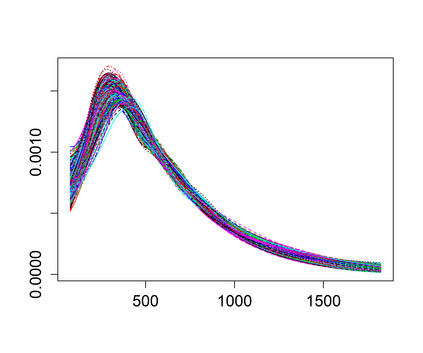
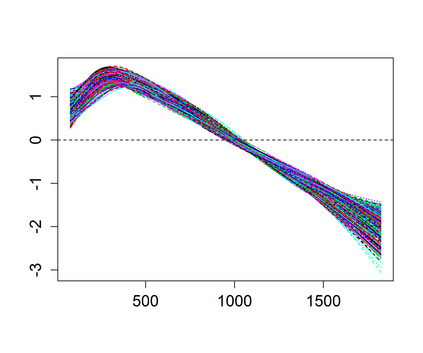
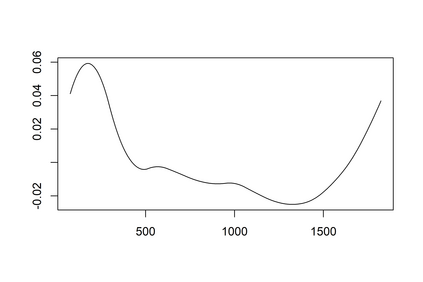
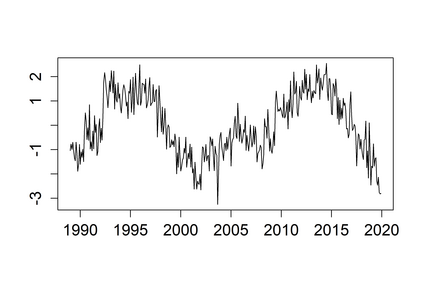
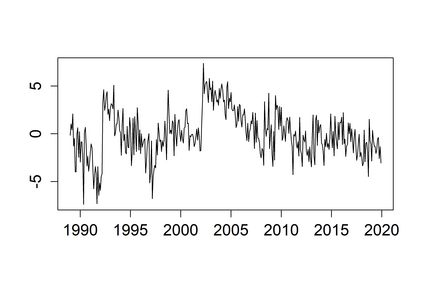
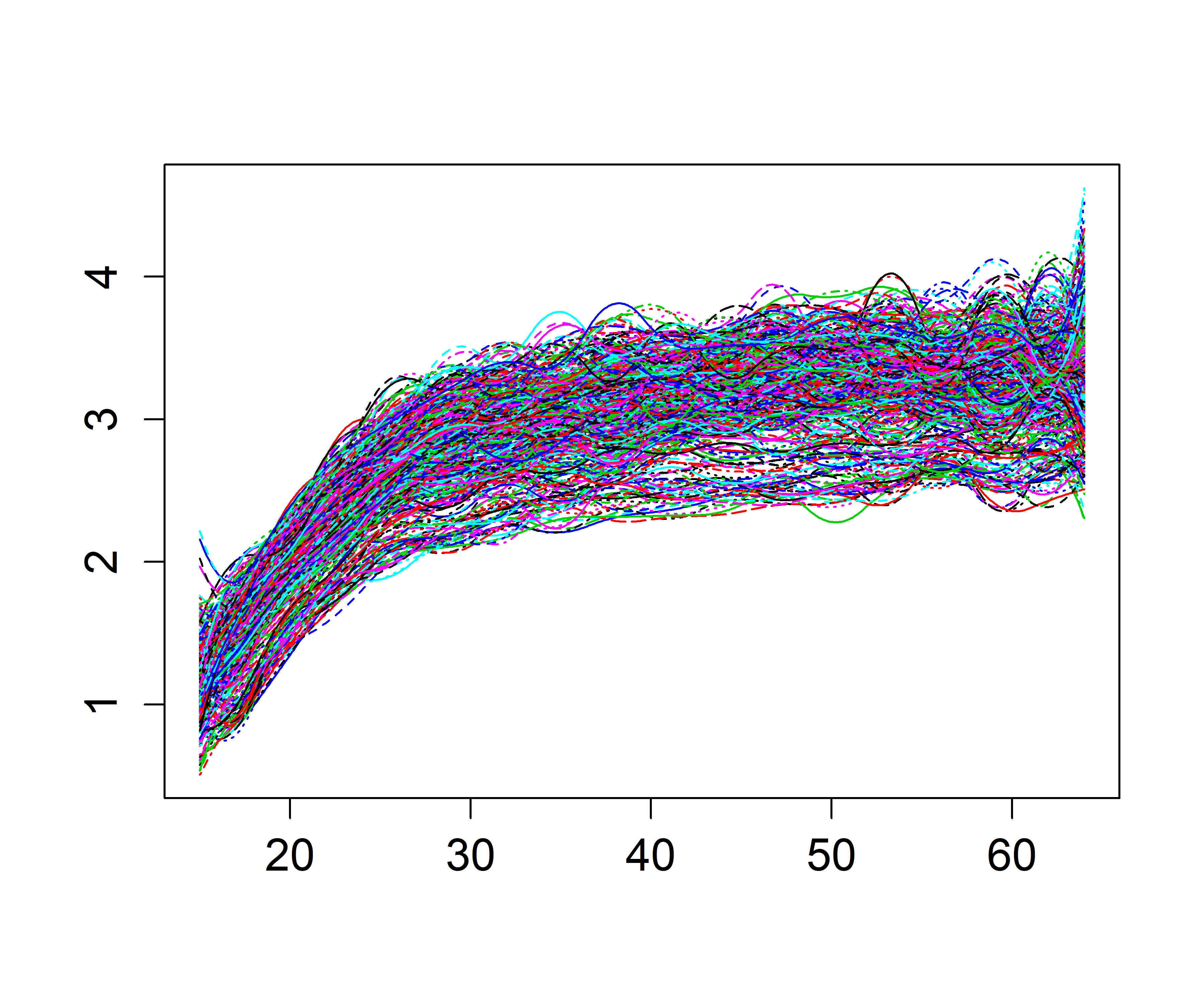
Functional principal component analysis (FPCA) has played an important role in the development of functional time series analysis. This paper investigates how FPCA can be used to analyze cointegrated functional time series and proposes a modification of FPCA as a novel statistical tool. Our modified FPCA not only provides an asymptotically more efficient estimator of the cointegrating vectors, but also leads to novel FPCA-based tests for examining some essential properties of cointegrated functional time series. We apply our methodology to two empirical examples: U.S. age-specific employment rates and earning densities.