Unified Fourier-based Kernel and Nonlinearity Design for Equivariant Networks on Homogeneous Spaces

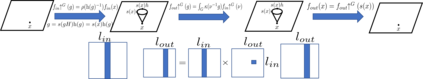
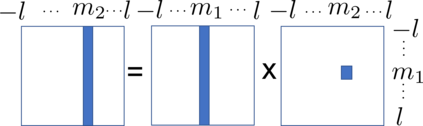
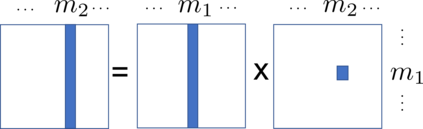
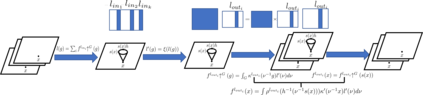
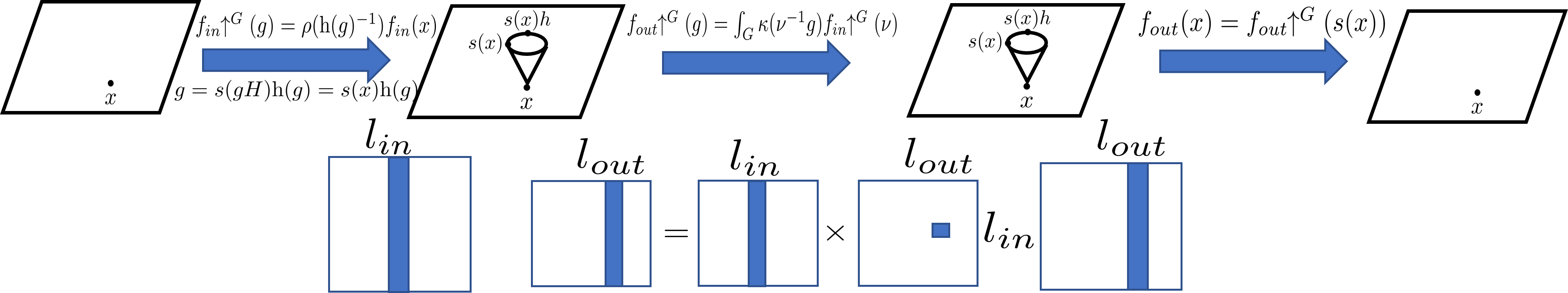
We introduce a unified framework for group equivariant networks on homogeneous spaces derived from a Fourier perspective. We address the case of feature fields being tensor valued before and after a convolutional layer. We present a unified derivation of kernels via the Fourier domain by taking advantage of the sparsity of Fourier coefficients of the lifted feature fields. The sparsity emerges when the stabilizer subgroup of the homogeneous space is a compact Lie group. We further introduce an activation method via an elementwise nonlinearity on the regular representation after lifting and projecting back to the field through an equivariant convolution. We show that other methods treating features as the Fourier coefficients in the stabilizer subgroup are special cases of our activation. Experiments on $SO(3)$ and $SE(3)$ show state-of-the-art performance in spherical vector field regression, point cloud classification, and molecular completion.