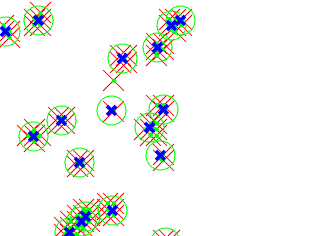
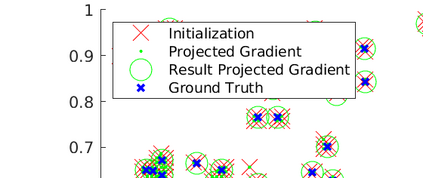
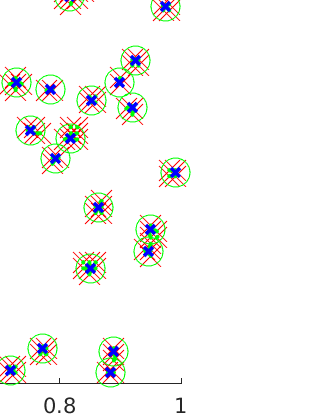
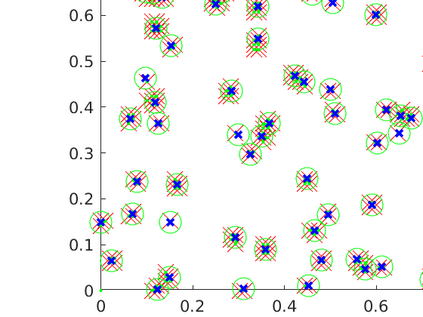
Theoretical results show that sparse off-the-grid spikes can be estimated from (possibly compressive) Fourier measurements under a minimum separation assumption. We propose a practical algorithm to minimize the corresponding non-convex functional based on a projected gradient descent coupled with an initialization procedure. We give qualitative insights on the theoretical foundations of the algorithm and provide experiments showing its potential for imaging problems.
翻译:理论结果显示,在最小分离假设下,从(可能压缩的)Fourier测量中可以估算出稀疏的网外峰值。 我们提出了一个实用的算法,以根据预测的梯度下降和初始化程序,尽量减少相应的非凝固功能。 我们对算法的理论基础有质的洞察力,并进行实验,展示算法在成像问题上的潜力。
相关内容
专知会员服务
21+阅读 · 2020年6月13日
专知会员服务
54+阅读 · 2020年3月5日
Arxiv
13+阅读 · 2020年6月24日