Efficient Information Geometry Approach for Massive MIMO-OFDM Channel Estimation

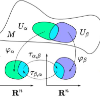
We investigate the channel estimation for massive multiple-input multiple-output orthogonal frequency division multiplexing (MIMO-OFDM) systems. We revisit the information geometry approach (IGA) for massive MIMO-OFDM channel estimation. By using the constant magnitude property of the entries of the measurement matrix, we find that the second-order natural parameters of the distributions on all the auxiliary manifolds are equivalent to each other, and the first-order natural parameters are asymptotically equivalent to each other at the fixed point. Motivated by these results, we simplify the process of IGA and propose an efficient IGA (EIGA) for massive MIMO-OFDM channel estimation, which allows efficient implementation with fast Fourier transformation (FFT). We then establish a sufficient condition of its convergence and accordingly find a range of the damping factor for the convergence. We show that this range of damping factor is sufficiently wide by using the specific properties of the measurement matrices. Further, we prove that at the fixed point, the a posteriori mean obtained by EIGA is asymptotically optimal. Simulations confirm that EIGA can achieve the optimal performance with low complexity in a limited number of iterations.