Theoretical analysis and numerical approximation for the stochastic thermal quasi-geostrophic model

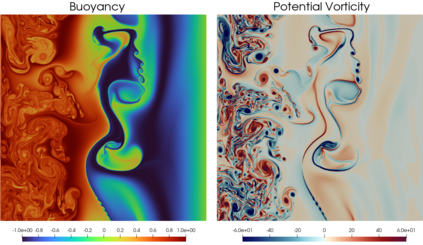
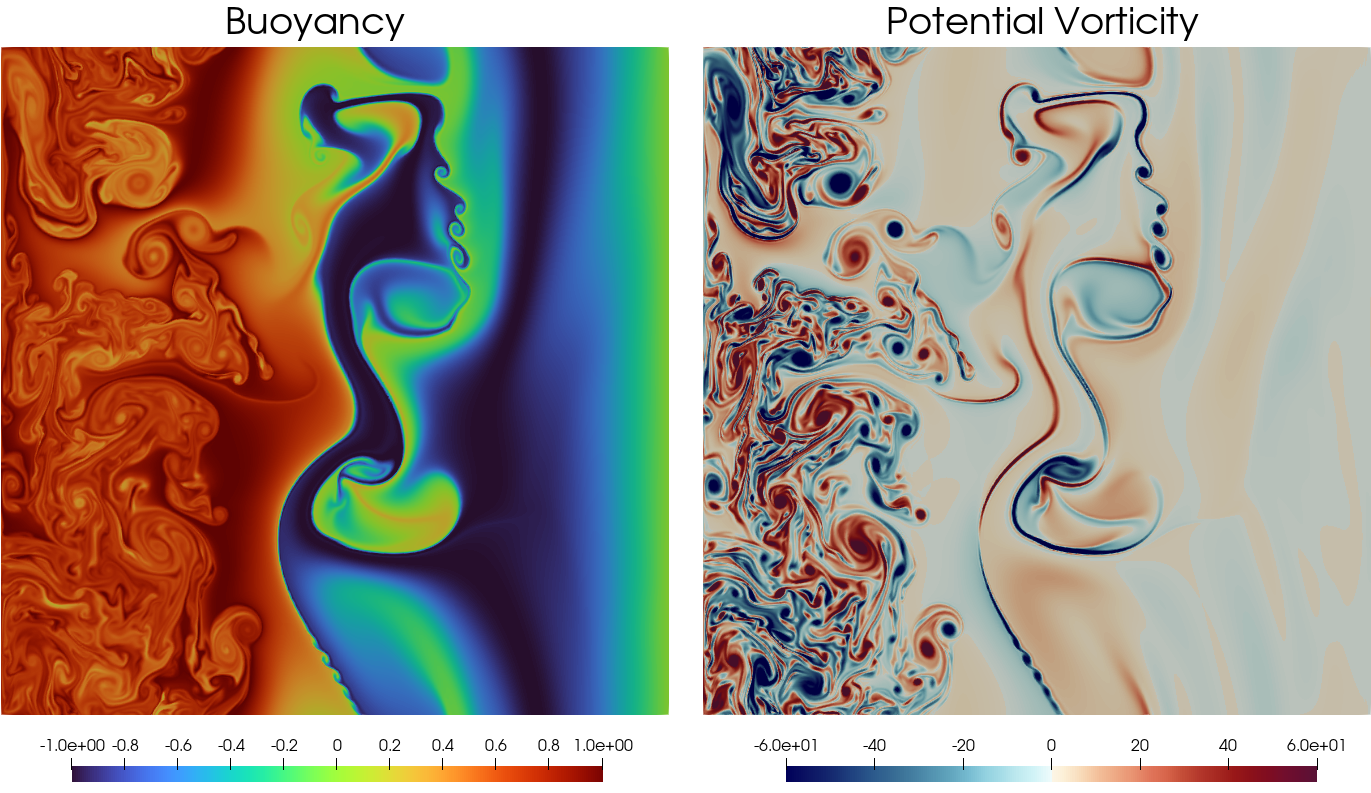
This paper investigates the mathematical properties of a stochastic version of the balanced 2D thermal quasigeostrophic (TQG) model of potential vorticity dynamics. This stochastic TQG model is intended as a basis for parametrisation of the dynamical creation of unresolved degrees of freedom in computational simulations of upper ocean dynamics when horizontal buoyancy gradients and bathymetry affect the dynamics, particularly at the submesoscale (250m-10km). Specifically, we have chosen the SALT (Stochastic Advection by Lie Transport) algorithm introduced in [25] and applied in [11,12] as our modelling approach. The SALT approach preserves the Kelvin circulation theorem and an infinite family of integral conservation laws for TQG. The goal of the SALT algorithm is to quantify the uncertainty in the process of up-scaling, or coarse-graining of either observed or synthetic data at fine scales, for use in computational simulations at coarser scales. The present work provides a rigorous mathematical analysis of the solution properties of the thermal quasigeostrophic (TQG) equations with stochastic advection by Lie transport (SALT) [27,28].