A Geometric Perspective on Double Robustness by Semiparametric Theory and Information Geometry

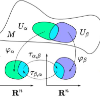
Double robustness (DR) is a widely-used property of estimators that provides protection against model misspecification and slow convergence of nuisance functions. While DR is a global property on the probability distribution manifold, it often coincides with influence curves, which only ensure orthogonality to nuisance directions locally. This apparent discrepancy raises fundamental questions about the theoretical underpinnings of DR. In this short communication, we address two key questions: (1) Why do influence curves frequently imply DR "for free"? (2) Under what conditions do DR estimators exist for a given statistical model and parameterization? Using tools from semiparametric theory, we show that convexity is the crucial property that enables influence curves to imply DR. We then derive necessary and sufficient conditions for the existence of DR estimators under a mean squared differentiable path-connected parameterization. Our main contribution also lies in the novel geometric interpretation of DR using information geometry. By leveraging concepts such as parallel transport, m-flatness, and m-curvature freeness, we characterize DR in terms of invariance along submanifolds. This geometric perspective deepens the understanding of when and why DR estimators exist. The results not only resolve apparent mysteries surrounding DR but also have practical implications for the construction and analysis of DR estimators. The geometric insights open up new connections and directions for future research. Our findings aim to solidify the theoretical foundations of a fundamental concept and contribute to the broader understanding of robust estimation in statistics.