Solving the decision-making differential equations from eye fixation data in Unity software by using Hermite Long-Short-Term Memory neural network

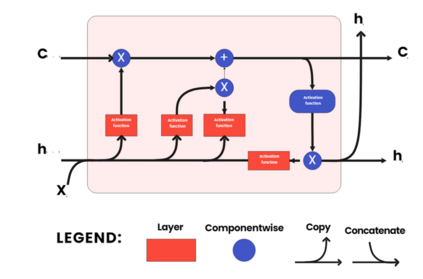
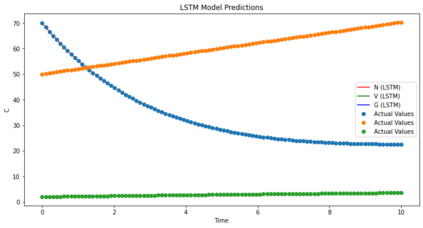
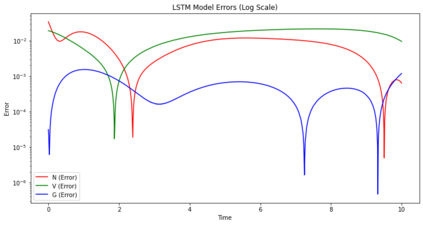
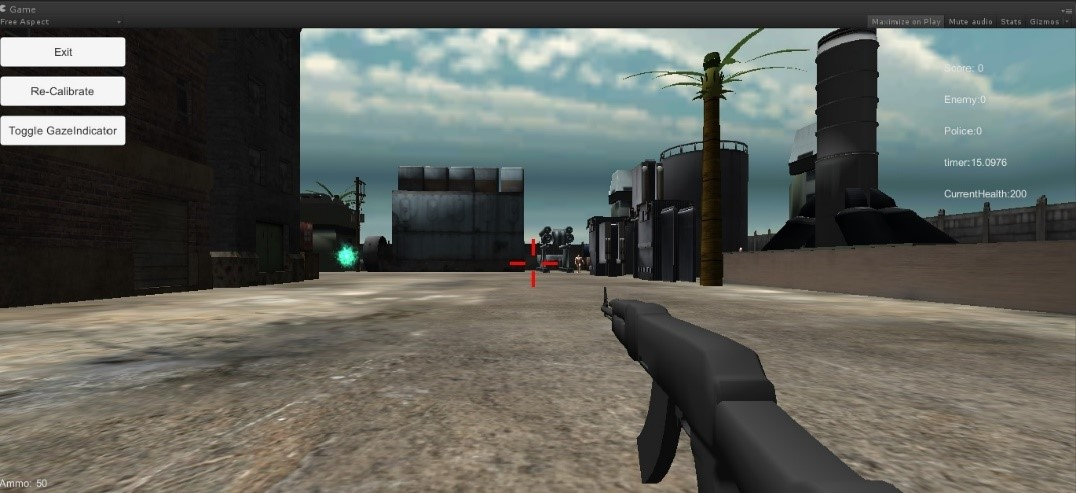
Cognitive decision-making processes are crucial aspects of human behavior, influencing various personal and professional domains. This research delves into the application of differential equations in analyzing decision-making accuracy by leveraging eye-tracking data within a virtual industrial town setting. The study unveils a systematic approach to transforming raw data into a differential equation, essential for deciphering the relationship between eye movements during decision-making processes. Mathematical relationship extraction and variable-parameter definition pave the way for deriving a differential equation that encapsulates the growth of fixations on characters. The key factors in this equation encompass the fixation rate $(\lambda)$ and separation rate $(\mu)$, reflecting user interaction dynamics and their impact on decision-making complexities tied to user engagement with virtual characters. For a comprehensive grasp of decision dynamics, solving this differential equation requires initial fixation counts, fixation rate, and separation rate. The formulation of differential equations incorporates various considerations such as engagement duration, character-player distance, relative speed, and character attributes, enabling the representation of fixation changes, speed dynamics, distance variations, and the effects of character attributes. This comprehensive analysis not only enhances our comprehension of decision-making processes but also provides a foundational framework for predictive modeling and data-driven insights for future research and applications in cognitive science and virtual reality environments.