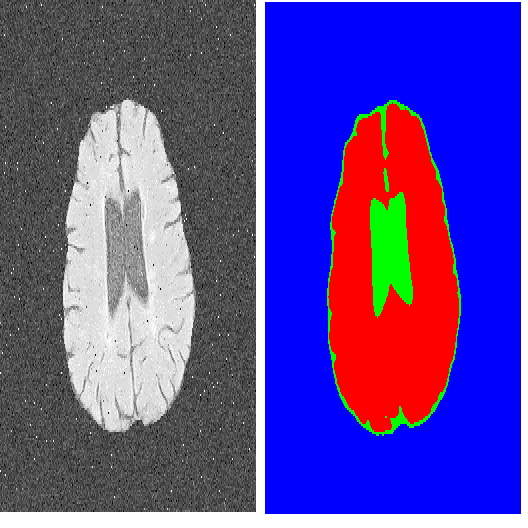
Probabilistic finite mixture models are widely used for unsupervised clustering. These models can often be improved by adapting them to the topology of the data. For instance, in order to classify spatially adjacent data points similarly, it is common to introduce a Laplacian constraint on the posterior probability that each data point belongs to a class. Alternatively, the mixing probabilities can be treated as free parameters, while assuming Gauss-Markov or more complex priors to regularize those mixing probabilities. However, these approaches are constrained by the shape of the prior and often lead to complicated or intractable inference. Here, we propose a new parametrization of the Dirichlet distribution to flexibly regularize the mixing probabilities of over-parametrized mixture distributions. Using the Expectation-Maximization algorithm, we show that our approach allows us to define any linear update rule for the mixing probabilities, including spatial smoothing regularization as a special case. We then show that this flexible design can be extended to share class information between multiple mixture models. We apply our algorithm to artificial and natural image segmentation tasks, and we provide quantitative and qualitative comparison of the performance of Gaussian and Student-t mixtures on the Berkeley Segmentation Dataset. We also demonstrate how to propagate class information across the layers of deep convolutional neural networks in a probabilistically optimal way, suggesting a new interpretation for feedback signals in biological visual systems. Our flexible approach can be easily generalized to adapt probabilistic mixture models to arbitrary data topologies.
翻译:概率有限混合模型被广泛用于不受监督的组合。 这些模型通常可以通过将其适应于数据表层来改进。 例如,为了对空间相邻数据点进行类似的分类,通常会对每个数据点属于某一类的远端概率采用拉巴西限制。 或者,混合概率可以被视为自由参数,而假设高斯-马尔科夫或更复杂的前期可以对这些混合概率进行规范。 但是,这些方法往往会受到先前的形状的制约,而且往往会导致复杂或难以调和的推断。 在这里,我们提议对dirichlet分布进行新的直观化,以便灵活地规范每个数据点属于某一类的相邻数据点的混合概率。我们使用期望-最大化算法,表明我们的方法可以界定混合概率的任何线性更新规则,包括空间平稳的调节作为特例。 然后我们表明,这种灵活设计可以轻易地扩展到多个混合模型之间共享类信息。 我们用我们的算法和自然均匀的直径直线性流数据分配方法, 也用我们的方法, 来在水平级结构中, 我们用一个定量和定性的平级数据级的比值分析方法, 来显示我们的数据的定性和定量的比值的等级的等级分析。