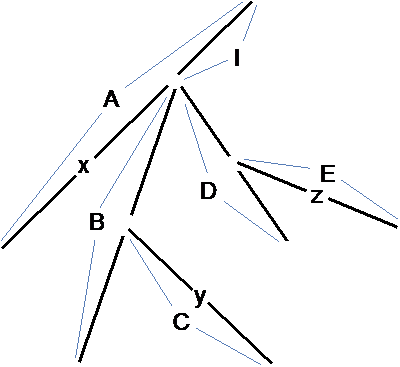
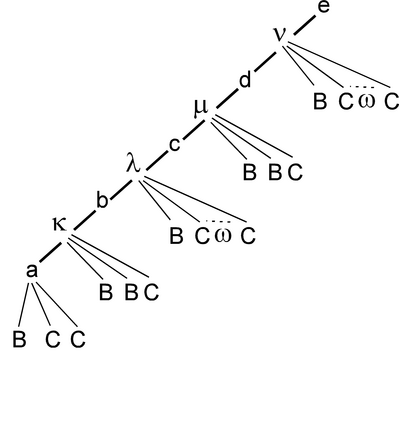
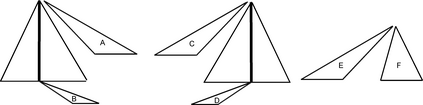
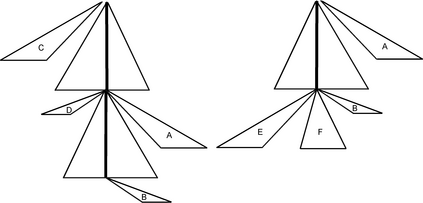
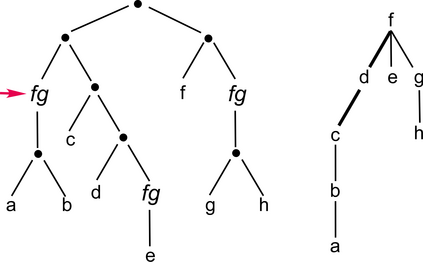
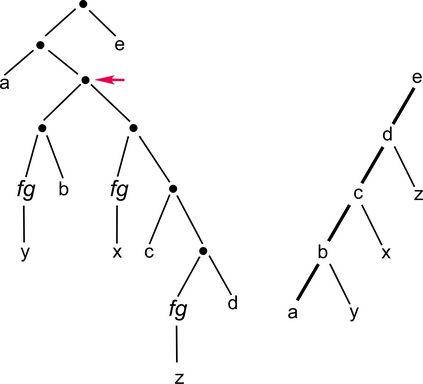
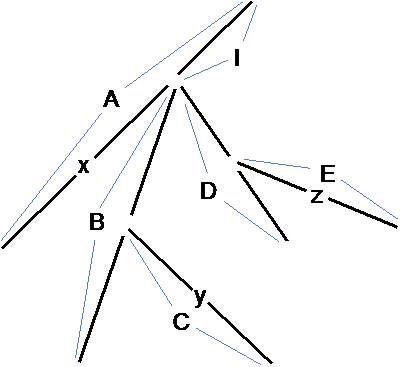
An order-theoretic forest is a countable partial order such that the set of elements larger than any element is linearly ordered. It is an order-theoretic tree if any two elements have an upper-bound. The order type of a branch can be any countable linear order. Such generalized infinite trees yield convenient definitions of the rank-width and the modular decomposition of countable graphs. We define an algebra based on only four operations that generate up to isomorphism and via infinite terms these order-theoretic trees and forests. We prove that the associated regular objects, those defined by regular terms, are exactly the ones that are the unique models of monadic second-order sentences.
翻译:定序理论森林是一个可计算的部分顺序, 使大于任何元素的元素组成为线性排列。 如果任何两个元素都有上限, 它是一个有定序理论的树。 分支的顺序类型可以是任何可计算线性顺序。 这种无穷无穷无穷的树可以产生对可计数图的位宽和模块分解的方便定义。 我们定义代数时仅以产生无形态论的四种操作为基础, 并且用无限的术语来定义这些定序理论的树木和森林。 我们证明, 相关的常规物体, 由常规术语定义的物体, 恰恰是莫纳迪二阶句的独特模型 。