A Sheaf-Theoretic Construction of Shape Space

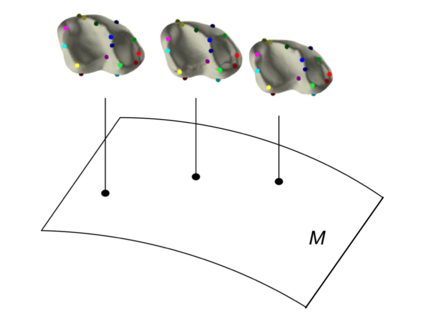
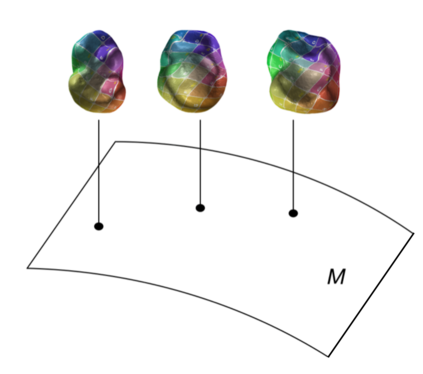
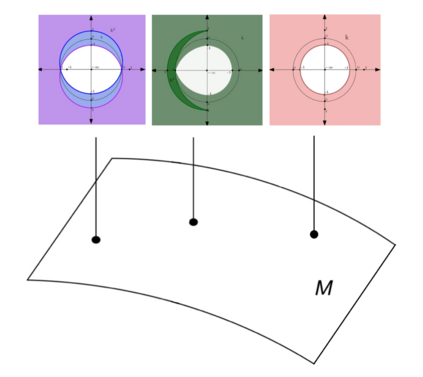
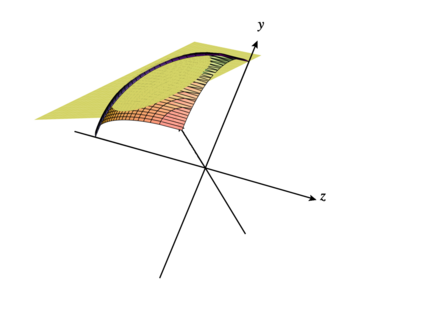
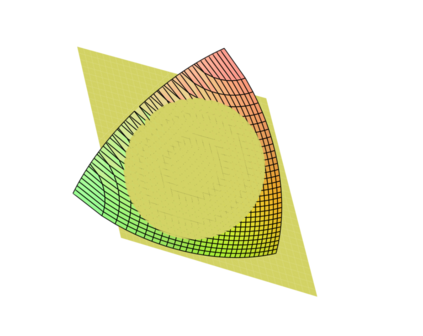
We present a sheaf-theoretic construction of shape space -- the space of all shapes. We do this by describing a homotopy sheaf on the poset category of constructible sets, where each set is mapped to its Persistent Homology Transform (PHT). Recent results that build on fundamental work of Schapira have shown that this transform is injective, thus making the PHT a good summary object for each shape. Our homotopy sheaf result allows us to "glue" PHTs of different shapes together to build up the PHT of a larger shape. In the case where our shape is a polyhedron we prove a generalized nerve lemma for the PHT. Finally, by re-examining the sampling result of Smale-Niyogi-Weinberger, we show that we can reliably approximate the PHT of a manifold by a polyhedron up to arbitrary precision.